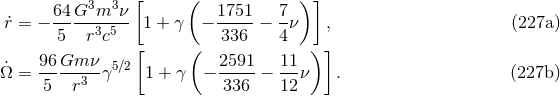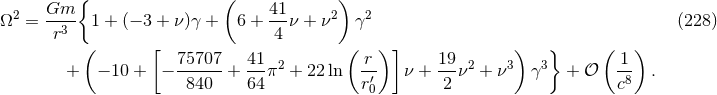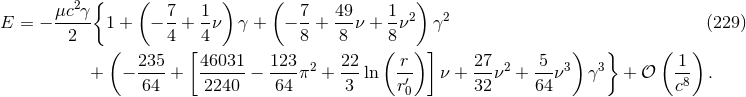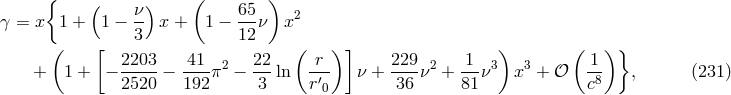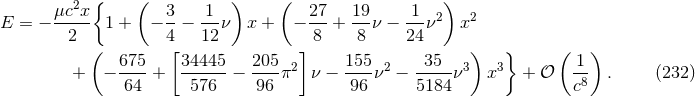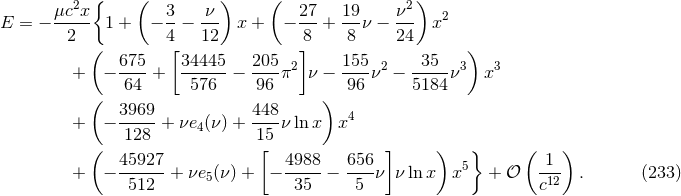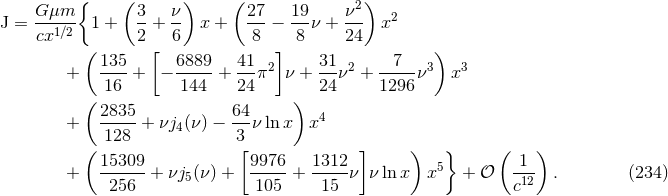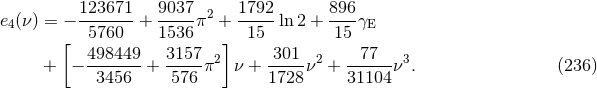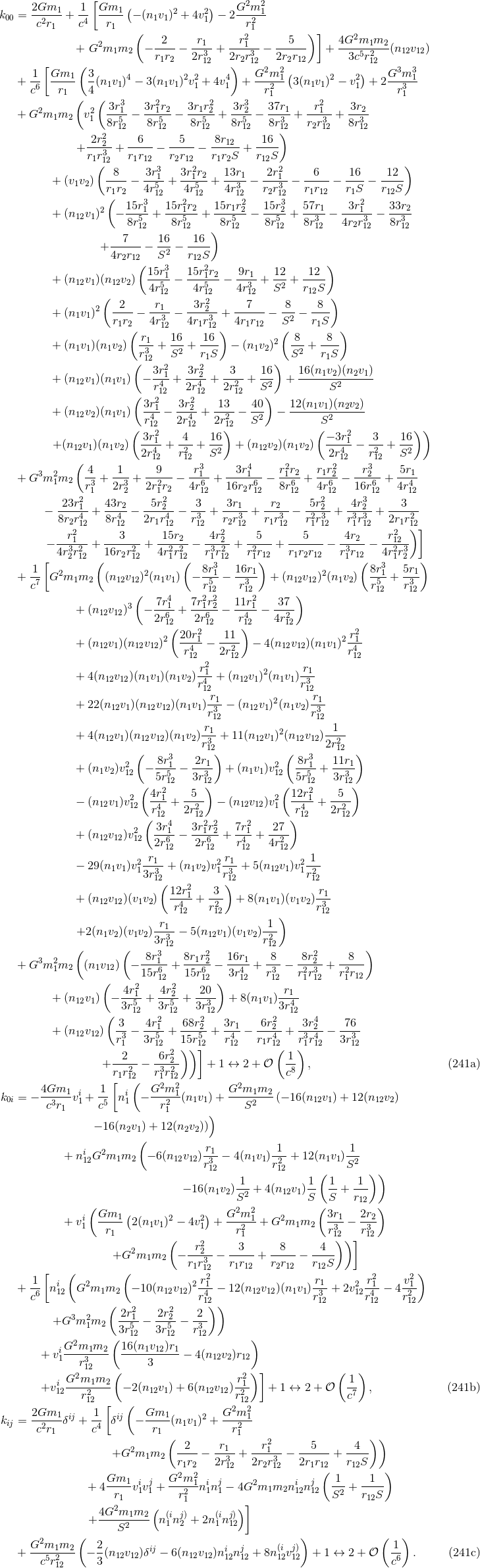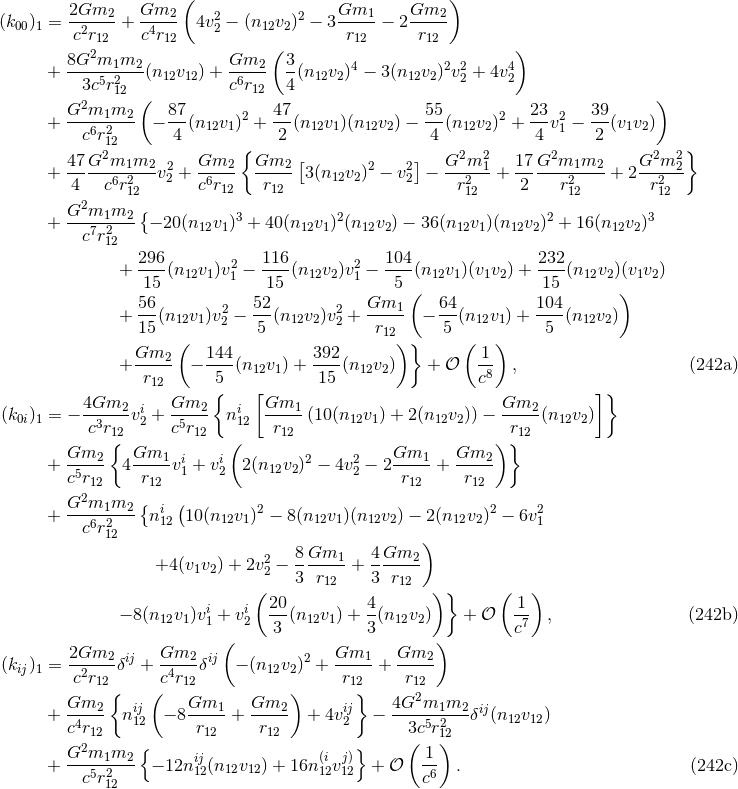7 Newtonian-like Equations of Motion
7.1 The 3PN acceleration and energy for particles
We present the acceleration of one of the particles, say the particle 1, at the 3PN order, as well as the 3PN
energy of the binary, which is conserved in the absence of radiation reaction. To get this result we used
essentially a “direct” post-Newtonian method (issued from Ref. [76*]), which consists of reducing the
3PN metric of an extended regular source, worked out in Eqs. (144), to the case where the
matter tensor is made of delta functions, and then curing the self-field divergences by means of
the Hadamard regularization technique. The equations of motion are simply the 3PN geodesic
equations explicitly provided in Eqs. (150*) – (152); the metric therein is the regularized metric
generated by the system of particles itself. Hadamard’s regularization permits to compute all
the terms but one, and the Hadamard ambiguity parameter 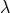 is obtained from dimensional
regularization; see Section 6.3. We also add the 3.5PN terms in harmonic coordinates which are
known from Refs. [258*, 259*, 260*, 336*, 278*, 322*, 254*]. These correspond to radiation reaction
effects at relative 1PN order (see Section 5.4 for discussion on radiation reaction up to 1.5PN
order).
is obtained from dimensional
regularization; see Section 6.3. We also add the 3.5PN terms in harmonic coordinates which are
known from Refs. [258*, 259*, 260*, 336*, 278*, 322*, 254*]. These correspond to radiation reaction
effects at relative 1PN order (see Section 5.4 for discussion on radiation reaction up to 1.5PN
order).
Though the successive post-Newtonian approximations are really a consequence of general relativity, the final equations of motion must be interpreted in a Newtonian-like fashion. That is, once a convenient general-relativistic (Cartesian) coordinate system is chosen, we should express the results in terms of the coordinate positions, velocities, and accelerations of the bodies, and view the trajectories of the particles as taking place in the absolute Euclidean space of Newton. But because the equations of motion are actually relativistic, they must:
- Stay manifestly invariant – at least in harmonic coordinates – when we perform a global post-Newtonian-expanded Lorentz transformation;
- Possess the correct “perturbative” limit, given by the geodesics of the (post-Newtonian-expanded) Schwarzschild metric, when one of the masses tends to zero;
- Be conservative, i.e., to admit a Lagrangian or Hamiltonian formulation, when the gravitational radiation reaction is turned off.
We denote by 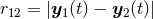 the harmonic-coordinate distance between the two particles, with
the harmonic-coordinate distance between the two particles, with
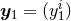 and
and 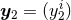 , by
, by 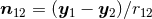 the corresponding unit direction, and by
the corresponding unit direction, and by
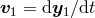 and
and 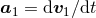 the coordinate velocity and acceleration of the particle 1 (and idem for
2). Sometimes we pose
the coordinate velocity and acceleration of the particle 1 (and idem for
2). Sometimes we pose 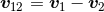 for the relative velocity. The usual Euclidean scalar product of
vectors is denoted with parentheses, e.g.,
for the relative velocity. The usual Euclidean scalar product of
vectors is denoted with parentheses, e.g., 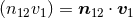 and
and 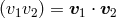 . The equations of the
body 2 are obtained by exchanging all the particle labels
. The equations of the
body 2 are obtained by exchanging all the particle labels 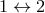 (remembering that
(remembering that  and
and  change sign in this operation):
change sign in this operation):
The 2.5PN and 3.5PN terms are associated with gravitational radiation
reaction.49
The 3PN harmonic-coordinates equations of motion depend on two arbitrary length scales 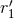 and
and 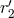 associated with the logarithms present at the 3PN order. It has been proved in Ref. [71*] that
associated with the logarithms present at the 3PN order. It has been proved in Ref. [71*] that 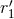 and
and 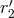 are merely linked with the choice of coordinates – we can refer to
are merely linked with the choice of coordinates – we can refer to 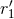 and
and 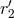 as “gauge constants”. In
our approach [69*, 71], the harmonic coordinate system is not uniquely fixed by the coordinate
condition
as “gauge constants”. In
our approach [69*, 71], the harmonic coordinate system is not uniquely fixed by the coordinate
condition 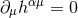 . In fact there are infinitely many “locally-defined” harmonic coordinate
systems. For general smooth matter sources, as in the general formalism of Part A, we expect
the existence and uniqueness of a global harmonic coordinate system. But here we have some
point-particles, with delta-function singularities, and in this case we do not have the notion of a global
coordinate system. We can always change the harmonic coordinates by means of the gauge vector
. In fact there are infinitely many “locally-defined” harmonic coordinate
systems. For general smooth matter sources, as in the general formalism of Part A, we expect
the existence and uniqueness of a global harmonic coordinate system. But here we have some
point-particles, with delta-function singularities, and in this case we do not have the notion of a global
coordinate system. We can always change the harmonic coordinates by means of the gauge vector
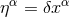 , satisfying
, satisfying 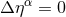 except at the location of the two particles (we assume that the
transformation is at the 3PN level, so we can consider simply a flat-space Laplace equation).
More precisely, we can show that the logarithms appearing in Eq. (203), together with the
constants
except at the location of the two particles (we assume that the
transformation is at the 3PN level, so we can consider simply a flat-space Laplace equation).
More precisely, we can show that the logarithms appearing in Eq. (203), together with the
constants 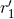 and
and 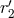 therein, can be removed by the coordinate transformation associated
with the 3PN gauge vector (with
therein, can be removed by the coordinate transformation associated
with the 3PN gauge vector (with 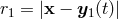 and
and 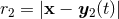 ; and
; and 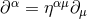 ):
):
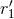 and
and 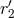 is innocuous on the physical point
of view, because the physical results must be gauge invariant. Indeed we shall verify that
is innocuous on the physical point
of view, because the physical results must be gauge invariant. Indeed we shall verify that 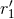 and
and 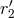 cancel out in our final results.
cancel out in our final results.
When retaining the “even” relativistic corrections at the 1PN, 2PN and 3PN orders, and neglecting the “odd” radiation reaction terms at the 2.5PN and 3.5PN orders, we find that the equations of motion admit a conserved energy (and a Lagrangian, as we shall see); that energy can be straightforwardly obtained by guess-work starting from Eq. (203), with the result
To the terms given above, we must add the same terms but corresponding to the relabelling 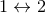 .
Actually, this energy is not conserved because of the radiation reaction. Thus its time derivative, as
computed by means of the 3PN equations of motion themselves (i.e., by order-reducing all the
accelerations), is purely equal to the 2.5PN effect,
.
Actually, this energy is not conserved because of the radiation reaction. Thus its time derivative, as
computed by means of the 3PN equations of motion themselves (i.e., by order-reducing all the
accelerations), is purely equal to the 2.5PN effect,
The resulting energy balance equation can be better expressed by transfering to the left-hand side certain 2.5PN terms so that we recognize in the right-hand side the familiar form of a total energy flux. Posing
we find agreement with the standard Einstein quadrupole formula (4*): where the Newtonian trace-free quadrupole moment reads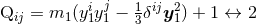 . We refer
to [258*, 259*] for the discussion of the energy balance equation up to the next 3.5PN order.
See also Eq. (158) for the energy balance equation at relative 1.5PN order for general fluid
systems.
. We refer
to [258*, 259*] for the discussion of the energy balance equation up to the next 3.5PN order.
See also Eq. (158) for the energy balance equation at relative 1.5PN order for general fluid
systems.
7.2 Lagrangian and Hamiltonian formulations
The conservative part of the equations of motion in harmonic coordinates (203) is derivable from a
generalized Lagrangian, depending not only on the positions and velocities of the bodies, but also on their
accelerations: 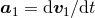 and
and 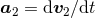 . As shown in Ref. [147], the accelerations in the
harmonic-coordinates Lagrangian occur already from the 2PN order. This fact is in accordance with
a general result [308] that N-body equations of motion cannot be derived from an ordinary
Lagrangian beyond the 1PN level, provided that the gauge conditions preserve the manifest Lorentz
invariance. Note that we can always arrange for the dependence of the Lagrangian upon the
accelerations to be linear, at the price of adding some so-called “multi-zero” terms to the Lagrangian,
which do not modify the equations of motion (see, e.g., Ref. [169]). At the 3PN level, we find
that the Lagrangian also depends on accelerations. It is notable that these accelerations are
sufficient – there is no need to include derivatives of accelerations. Note also that the Lagrangian is
not unique because we can always add to it a total time derivative
. As shown in Ref. [147], the accelerations in the
harmonic-coordinates Lagrangian occur already from the 2PN order. This fact is in accordance with
a general result [308] that N-body equations of motion cannot be derived from an ordinary
Lagrangian beyond the 1PN level, provided that the gauge conditions preserve the manifest Lorentz
invariance. Note that we can always arrange for the dependence of the Lagrangian upon the
accelerations to be linear, at the price of adding some so-called “multi-zero” terms to the Lagrangian,
which do not modify the equations of motion (see, e.g., Ref. [169]). At the 3PN level, we find
that the Lagrangian also depends on accelerations. It is notable that these accelerations are
sufficient – there is no need to include derivatives of accelerations. Note also that the Lagrangian is
not unique because we can always add to it a total time derivative 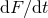 , where
, where 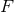 is
any function depending on the positions and velocities, without changing the dynamics. We
find [174*]
is
any function depending on the positions and velocities, without changing the dynamics. We
find [174*]
Witness the accelerations occurring at the 2PN and 3PN orders; see also the gauge-dependent
logarithms of 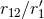 and
and 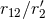 . We refer to [174*] for the explicit expressions of the ten conserved
quantities corresponding to the integrals of energy [also given in Eq. (205)], linear and angular momenta,
and center-of-mass position. Notice that while it is strictly forbidden to replace the accelerations by the
equations of motion in the Lagrangian, this can and should be done in the final expressions of the conserved
integrals derived from that Lagrangian.
. We refer to [174*] for the explicit expressions of the ten conserved
quantities corresponding to the integrals of energy [also given in Eq. (205)], linear and angular momenta,
and center-of-mass position. Notice that while it is strictly forbidden to replace the accelerations by the
equations of motion in the Lagrangian, this can and should be done in the final expressions of the conserved
integrals derived from that Lagrangian.
Now we want to exhibit a transformation of the particles’ dynamical variables – or contact
transformation, as it is called in the jargon – which transforms the 3PN harmonic-coordinates
Lagrangian (209) into a new Lagrangian, valid in some ADM or ADM-like coordinate system, and such
that the associated Hamiltonian coincides with the 3PN Hamiltonian that has been obtained by Jaranowski
& Schäfer [261*, 262*]. In ADM coordinates the Lagrangian will be ordinary, depending only on the
positions and velocities of the bodies. Let this contact transformation be  and
and
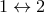 , where
, where 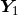 and
and 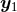 denote the trajectories in ADM and harmonic coordinates,
respectively. For this transformation to be able to remove all the accelerations in the initial
Lagrangian
denote the trajectories in ADM and harmonic coordinates,
respectively. For this transformation to be able to remove all the accelerations in the initial
Lagrangian 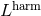 up to the 3PN order, we determine [174*] it to be necessarily of the form
up to the 3PN order, we determine [174*] it to be necessarily of the form
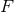 is a freely adjustable function of the positions and velocities, made of 2PN and 3PN terms, and
where
is a freely adjustable function of the positions and velocities, made of 2PN and 3PN terms, and
where 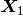 represents a special correction term, that is purely of order 3PN. The point is that once the
function
represents a special correction term, that is purely of order 3PN. The point is that once the
function 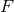 is specified there is a unique determination of the correction term
is specified there is a unique determination of the correction term 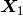 for the
contact transformation to work (see Ref. [174*] for the details). Thus, the freedom we have is
entirely encoded into the function
for the
contact transformation to work (see Ref. [174*] for the details). Thus, the freedom we have is
entirely encoded into the function 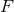 , and the work then consists in showing that there exists a
unique choice of
, and the work then consists in showing that there exists a
unique choice of 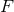 for which our Lagrangian
for which our Lagrangian 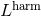 is physically equivalent, via the contact
transformation (210*), to the ADM Hamiltonian of Refs. [261*, 262*]. An interesting point is that
not only the transformation must remove all the accelerations in
is physically equivalent, via the contact
transformation (210*), to the ADM Hamiltonian of Refs. [261*, 262*]. An interesting point is that
not only the transformation must remove all the accelerations in 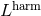 , but it should also
cancel out all the logarithms
, but it should also
cancel out all the logarithms 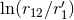 and
and 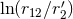 , because there are no logarithms
in ADM coordinates. The result we find, which can be checked to be in full agreement with
the expression of the gauge vector in Eq. (204*), is that
, because there are no logarithms
in ADM coordinates. The result we find, which can be checked to be in full agreement with
the expression of the gauge vector in Eq. (204*), is that 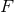 involves the logarithmic terms
together with many other non-logarithmic terms (indicated by dots) that are entirely specified by the
isometry of the harmonic and ADM descriptions of the motion. For this particular choice of
involves the logarithmic terms
together with many other non-logarithmic terms (indicated by dots) that are entirely specified by the
isometry of the harmonic and ADM descriptions of the motion. For this particular choice of 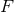 the ADM
Lagrangian reads
Inserting into this equation all our explicit expressions we find
the ADM
Lagrangian reads
Inserting into this equation all our explicit expressions we find
The notation is the same as in Eq. (209), except that we use upper-case letters to denote
the ADM-coordinates positions and velocities; thus, for instance 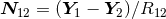 and
and
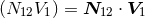 . The Hamiltonian is simply deduced from the latter Lagrangian by applying the usual
Legendre transformation. Posing
. The Hamiltonian is simply deduced from the latter Lagrangian by applying the usual
Legendre transformation. Posing 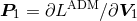 and
and 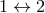 , we get [261*, 262*, 263, 162*, 174*]
, we get [261*, 262*, 263, 162*, 174*]
Arguably, the results given by the ADM-Hamiltonian formalism (for the problem at hand)
look simpler than their harmonic-coordinate counterparts. Indeed, the ADM Lagrangian is
ordinary – no accelerations – and there are no logarithms nor associated gauge constants  and
and
 .50
Of course, one is free to describe the binary motion in whatever coordinates one likes, and the two
formalisms, harmonic (209) and ADM (213) – (214), describe rigorously the same physics. On the other
hand, the higher complexity of the harmonic-coordinates Lagrangian (209) enables one to perform more
tests of the computations, notably by inquiring about the future of the constants
.50
Of course, one is free to describe the binary motion in whatever coordinates one likes, and the two
formalisms, harmonic (209) and ADM (213) – (214), describe rigorously the same physics. On the other
hand, the higher complexity of the harmonic-coordinates Lagrangian (209) enables one to perform more
tests of the computations, notably by inquiring about the future of the constants  and
and 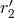 , that we
know must disappear from physical quantities such as the center-of-mass energy and the total
gravitational-wave flux.
, that we
know must disappear from physical quantities such as the center-of-mass energy and the total
gravitational-wave flux.
7.3 Equations of motion in the center-of-mass frame
In this section we translate the origin of coordinates to the binary’s center-of-mass by imposing the
vanishing of the binary’s mass dipole moment: 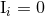 in the notation of Part A. Actually the dipole
moment is computed as the center-of-mass conserved integral associated with the boost symmetry of the
3PN equations of motion [174, 79*]. This condition results in the 3PN-accurate relationship between the
individual positions in the center-of-mass frame
in the notation of Part A. Actually the dipole
moment is computed as the center-of-mass conserved integral associated with the boost symmetry of the
3PN equations of motion [174, 79*]. This condition results in the 3PN-accurate relationship between the
individual positions in the center-of-mass frame 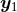 and
and 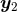 , and the relative position
, and the relative position 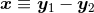 and
velocity
and
velocity 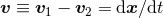 (formerly denoted
(formerly denoted 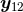 and
and 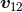 ). We shall also use the orbital
separation
). We shall also use the orbital
separation  , together with
, together with 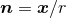 and
and 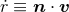 . Mass parameters are: The total mass
. Mass parameters are: The total mass
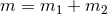 (to be distinguished from the ADM mass denoted by
(to be distinguished from the ADM mass denoted by 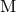 in Part A); the relative mass
difference
in Part A); the relative mass
difference 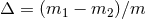 ; the reduced mass
; the reduced mass 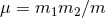 ; and the very useful symmetric mass
ratio
; and the very useful symmetric mass
ratio
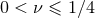 , with
, with 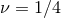 in the
case of equal masses, and
in the
case of equal masses, and 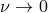 in the test-mass limit for one of the bodies. Thus
in the test-mass limit for one of the bodies. Thus 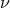 is numerically
rather small and may be viewed as a small expansion parameter. We also pose
is numerically
rather small and may be viewed as a small expansion parameter. We also pose 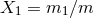 and
and
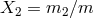 so that
so that 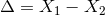 and
and 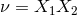 .
.
For reference we give the 3PN-accurate expressions of the individual positions in the center-of-mass frame in terms of relative variables. They are in the form
where all post-Newtonian corrections, beyond Newtonian order, are proportional to the mass
ratio 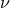 and the mass difference
and the mass difference 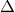 . The two dimensionless coefficients
. The two dimensionless coefficients 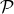 and
and 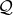 read
read
Up to 2.5PN order there is agreement with the circular-orbit limit of Eqs. (6.4) in Ref. [45]. Notice the
2.5PN radiation-reaction term entering the coefficient 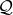 ; such 2.5PN term is explicitly displayed for
circular orbits in Eqs. (224) below. In Eqs. (217) the logarithms at the 3PN order appear only in the
coefficient
; such 2.5PN term is explicitly displayed for
circular orbits in Eqs. (224) below. In Eqs. (217) the logarithms at the 3PN order appear only in the
coefficient 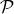 . They contain a particular combination
. They contain a particular combination 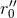 of the two gauge-constants
of the two gauge-constants 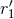 and
and 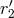 defined by
defined by
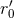 we shall find in the equations of relative
motion, see Eq. (221*).
we shall find in the equations of relative
motion, see Eq. (221*).
The 3PN and even 3.5PN center-of-mass equations of motion are obtained by replacing in the 3.5PN equations of motion (203) in a general frame, the positions and velocities by their center-of-mass expressions (216) – (217), applying as usual the order-reduction of all accelerations where necessary. We write the relative acceleration in the center-of-mass frame in the form
and find that the coefficients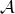 and
and 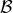 are [79*]
are [79*]
Up to the 2.5PN order the result agrees with Ref. [302]. The 3.5PN term is issued from
Refs. [258*, 259*, 260*, 336*, 278*, 322*, 254*]. At the 3PN order we have some gauge-dependent logarithms
containing a constant 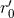 which is the “logarithmic barycenter” of the two constants
which is the “logarithmic barycenter” of the two constants 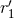 and
and 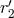 :
:
The logarithms in Eqs. (220), together with the constant 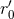 therein, can be removed by applying
the gauge transformation (204*), while still staying within the class of harmonic coordinates.
The resulting modification of the equations of motion will affect only the coefficients of the
3PN order in Eqs. (220); let us denote them by
therein, can be removed by applying
the gauge transformation (204*), while still staying within the class of harmonic coordinates.
The resulting modification of the equations of motion will affect only the coefficients of the
3PN order in Eqs. (220); let us denote them by 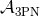 and
and 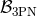 . The new values of these
coefficients, obtained after removal of the logarithms by the latter harmonic gauge transformation,
will be denoted
. The new values of these
coefficients, obtained after removal of the logarithms by the latter harmonic gauge transformation,
will be denoted 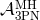 and
and 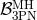 . Here MH stands for the modified harmonic coordinate
system, differing from the SH (standard harmonic) coordinate system containing logarithms at
the 3PN order in the coefficients
. Here MH stands for the modified harmonic coordinate
system, differing from the SH (standard harmonic) coordinate system containing logarithms at
the 3PN order in the coefficients 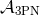 and
and 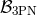 . See Ref. [9*] for a full description of the
coordinate transformation between SH and MH coordinates for various quantities. We have [320*, 9*]
. See Ref. [9*] for a full description of the
coordinate transformation between SH and MH coordinates for various quantities. We have [320*, 9*]
Again, the other terms in the equations of motion (219*) – (220) are unchanged. These gauge-transformed coefficients in MH coordinates are useful because they do not yield the usual complications associated with logarithms. However, they must be handled with care in applications such as in Ref. [320], because one must ensure that all other quantities in the problem (energy, angular momentum, gravitational-wave fluxes, etc.) are defined in the same specific MH gauge avoiding logarithms. In the following we shall no longer use the MH coordinate system leading to Eqs. (222), except when constructing the generalized quasi-Keplerian representation of the 3PN motion in Section 10.2. Therefore all expressions we shall derive below, notably all those concerning the radiation field, are valid in the SH coordinate system in which the equations of motion are fully given by Eq. (203) or, in the center-of-mass frame, by Eqs. (219*) – (220).
For future reference let also give the 3PN center-of-mass Hamiltonian in ADM coordinates derived in
Refs. [261, 262, 162]. In the center-of-mass frame the conjugate variables are the relative separation
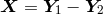 and the conjugate momentum (per unit reduced mass)
and the conjugate momentum (per unit reduced mass) 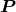 such that
such that 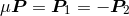 where
where 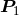 and
and 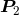 are defined in Section 7.2). Posing
are defined in Section 7.2). Posing 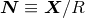 with
with  , together with
, together with
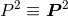 and
and 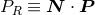 , we have
, we have
7.4 Equations of motion and energy for quasi-circular orbits
Most inspiralling compact binaries will have been circularized by the time they become visible by the
detectors LIGO and VIRGO; see Section 1.2. In the case of orbits that are circular – apart from the gradual
radiation-reaction inspiral – the complicated equations of motion simplify drastically, since we have
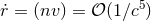 . For circular orbits, up to the 2.5PN order, the relation between center-of-mass
variables and the relative ones reads
. For circular orbits, up to the 2.5PN order, the relation between center-of-mass
variables and the relative ones reads
where we recall 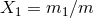 ,
, 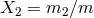 and
and 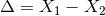 . See Eqs. (216) – (217) for more
general formulas. To conveniently display the successive post-Newtonian corrections, we employ the
post-Newtonian parameter
. See Eqs. (216) – (217) for more
general formulas. To conveniently display the successive post-Newtonian corrections, we employ the
post-Newtonian parameter
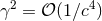 . See Ref. [79*] for a systematic calculation of Eqs. (224)
to higher order.
. See Ref. [79*] for a systematic calculation of Eqs. (224)
to higher order.
The relative acceleration 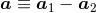 of two bodies moving on a circular orbit at the 3.5PN order is
then given by
of two bodies moving on a circular orbit at the 3.5PN order is
then given by
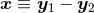 is the relative separation (in harmonic coordinates) and
is the relative separation (in harmonic coordinates) and 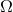 denotes the
angular frequency of the circular motion. The second term in Eq. (226*), opposite to the velocity
denotes the
angular frequency of the circular motion. The second term in Eq. (226*), opposite to the velocity
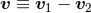 , represents the radiation reaction force up to 3.5PN order, which comes from the
reduction of the coefficients of
, represents the radiation reaction force up to 3.5PN order, which comes from the
reduction of the coefficients of 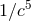 and
and 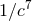 in Eqs. (220). The radiation-reaction force is
responsible for the secular decrease of the separation
in Eqs. (220). The radiation-reaction force is
responsible for the secular decrease of the separation  and increase of the orbital frequency
and increase of the orbital frequency 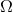 :
:
Concerning conservative effects, the main content of the 3PN equations (226*) is the relation between the
frequency 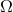 and the orbital separation
and the orbital separation  , which is given by the following generalized version of Kepler’s
third law:
, which is given by the following generalized version of Kepler’s
third law:
The length scale 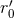 is given in terms of the two gauge-constants
is given in terms of the two gauge-constants 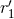 and
and 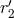 by Eq. (221*). As
for the energy, it is inferred from the circular-orbit reduction of the general result (205). We
have
by Eq. (221*). As
for the energy, it is inferred from the circular-orbit reduction of the general result (205). We
have
This expression is that of a physical observable 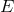 ; however, it depends on the choice of a coordinate
system, as it involves the post-Newtonian parameter
; however, it depends on the choice of a coordinate
system, as it involves the post-Newtonian parameter 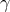 defined from the harmonic-coordinate separation
defined from the harmonic-coordinate separation
 . But the numerical value of
. But the numerical value of 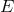 should not depend on the choice of a coordinate system, so
should not depend on the choice of a coordinate system, so 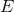 must
admit a frame-invariant expression, the same in all coordinate systems. To find it we re-express
must
admit a frame-invariant expression, the same in all coordinate systems. To find it we re-express 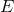 with
the help of the following frequency-related parameter
with
the help of the following frequency-related parameter 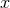 , instead of the post-Newtonian parameter
, instead of the post-Newtonian parameter
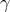 :51
:51
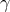 in terms of
in terms of 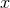 at 3PN order,
at 3PN order,
that we substitute back into Eq. (229), making all appropriate post-Newtonian re-expansions. As a
result, we gladly discover that the logarithms together with their associated gauge constant 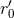 have
cancelled out. Therefore, our result is [160*, 69]
have
cancelled out. Therefore, our result is [160*, 69]
For circular orbits one can check that there are no terms of order 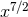 in Eq. (232), so this result is
actually valid up to the 3.5PN order. We shall discuss in Section 11 how the effects of the spins of the two
black holes affect the latter formula.
in Eq. (232), so this result is
actually valid up to the 3.5PN order. We shall discuss in Section 11 how the effects of the spins of the two
black holes affect the latter formula.
The formula (232) has been extended to include the logarithmic terms 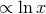 at the 4PN and 5PN orders [67*, 289*], that are due to tail effects occurring in
the near zone, see Sections 5.2 and 5.4. Adding also the Schwarzschild test-mass
limit52
up to 5PN order, we get:
at the 4PN and 5PN orders [67*, 289*], that are due to tail effects occurring in
the near zone, see Sections 5.2 and 5.4. Adding also the Schwarzschild test-mass
limit52
up to 5PN order, we get:
We can write also a similar expression for the angular momentum,
For circular orbits the energy 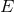 and angular momentum
and angular momentum 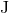 are known to be linked together by the
so-called “thermodynamic” relation
are known to be linked together by the
so-called “thermodynamic” relation
We have introduced in Eqs. (233) – (234) some non-logarithmic 4PN and 5PN coefficients 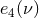 ,
,
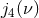 and
and 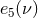 ,
, 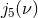 , which can however be proved to be polynomials in the symmetric mass ratio
, which can however be proved to be polynomials in the symmetric mass ratio
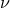 .53
Recent works on the 4PN approximation to the equations of motion by means of both EFT methods [204]
and the traditional ADM-Hamiltonian approach [264, 265], and complemented by an analytic computation
of the gravitational self-force in the small mass ratio
.53
Recent works on the 4PN approximation to the equations of motion by means of both EFT methods [204]
and the traditional ADM-Hamiltonian approach [264, 265], and complemented by an analytic computation
of the gravitational self-force in the small mass ratio 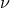 limit [36*], have yielded the next-order 4PN
coefficient as (
limit [36*], have yielded the next-order 4PN
coefficient as (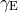 being Euler’s constant)
being Euler’s constant)
The numerical value 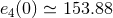 was predicted before thanks to a comparison with numerical
self-force calculations [289*, 287*].
was predicted before thanks to a comparison with numerical
self-force calculations [289*, 287*].
7.5 The 2.5PN metric in the near zone
The near-zone metric is given by Eqs. (144) for general post-Newtonian matter sources. For point-particles
binaries all the potentials 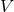 ,
, 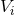 ,
, 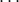 parametrizing the metric must be computed and iterated for
delta-function sources. Up to the 2.5PN order it is sufficient to cure the divergences due to singular sources
by means of the Hadamard self-field regularization. Let us point out that the computation is greatly helped
– and indeed is made possible at all – by the existence of the following solution
parametrizing the metric must be computed and iterated for
delta-function sources. Up to the 2.5PN order it is sufficient to cure the divergences due to singular sources
by means of the Hadamard self-field regularization. Let us point out that the computation is greatly helped
– and indeed is made possible at all – by the existence of the following solution 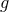 of the elementary
Poisson equation
of the elementary
Poisson equation
where 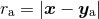 and
and 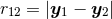 . Furthermore, to obtain the metric at the 2.5PN order, the
solutions of even more difficult elementary Poisson equations are required. Namely we meet
. Furthermore, to obtain the metric at the 2.5PN order, the
solutions of even more difficult elementary Poisson equations are required. Namely we meet
with 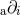 denoting the partial derivatives with respect to the source points
denoting the partial derivatives with respect to the source points 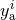 (and as usual
(and as usual 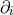 being the partial derivative with respect to the field point
being the partial derivative with respect to the field point 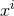 ). It is quite remarkable that
the solutions of the latter equations are known in closed analytic form. By combining several
earlier results from Refs. [120, 324, 377], one can write these solutions into the form [64*, 76*]
). It is quite remarkable that
the solutions of the latter equations are known in closed analytic form. By combining several
earlier results from Refs. [120, 324, 377], one can write these solutions into the form [64*, 76*]
where 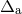 are the Laplacians with respect to the two source points.
are the Laplacians with respect to the two source points.
We report here the complete expression of the 2.5PN metric in harmonic coordinates valid at any field
point in the near zone. Posing 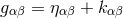 we have [76*]
we have [76*]
Here we pose 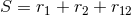 and
and 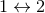 refers to the same quantity but with all particle labels
exchanged. To higher order one needs the solution of elementary equations still more intricate
than (239) and the 3PN metric valid in closed form all over the near zone is not currently
known.
refers to the same quantity but with all particle labels
exchanged. To higher order one needs the solution of elementary equations still more intricate
than (239) and the 3PN metric valid in closed form all over the near zone is not currently
known.
Let us also display the latter 2.5PN metric computed at the location of the particle 1 for instance, thanks to the Hadamard self-field regularization, i.e., in the sense of the Hadamard partie finie defined by Eq. (160*). We get
When regularized at the location of the particles, the metric can be computed to higher order, for instance 3PN. We shall need it when we compute the so-called redshift observable in Sections (8.3) and (8.4); indeed, see Eq. (276*).

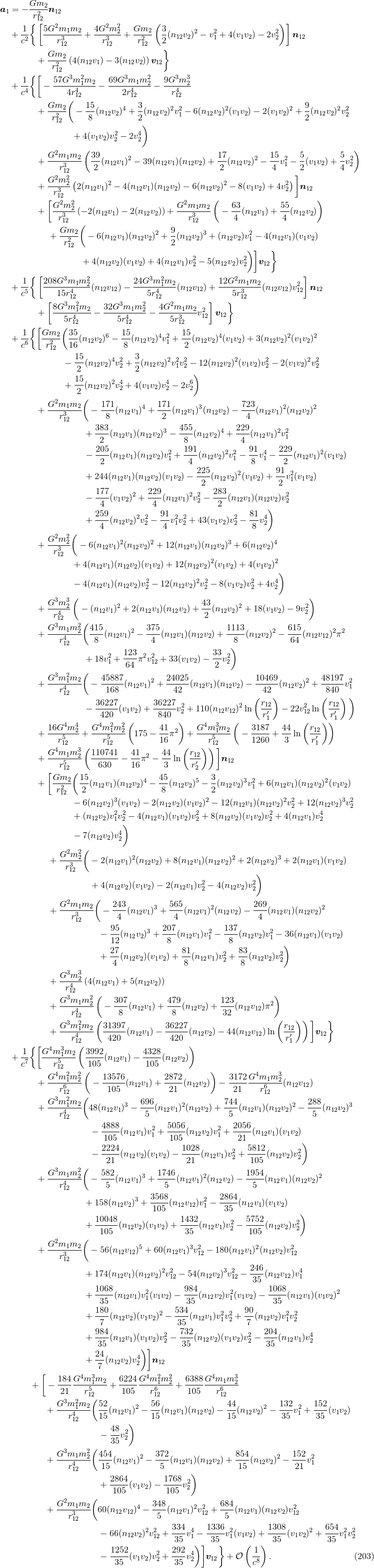
![[ ( ) ( ) ] α 22 G2m1m2 α Gm1 r12 Gm2 r12 η = − -------6----∂ -----ln --′ + -----ln --′ . (204 ) 3 c r2 r1 r1 r2](article1677x.gif)
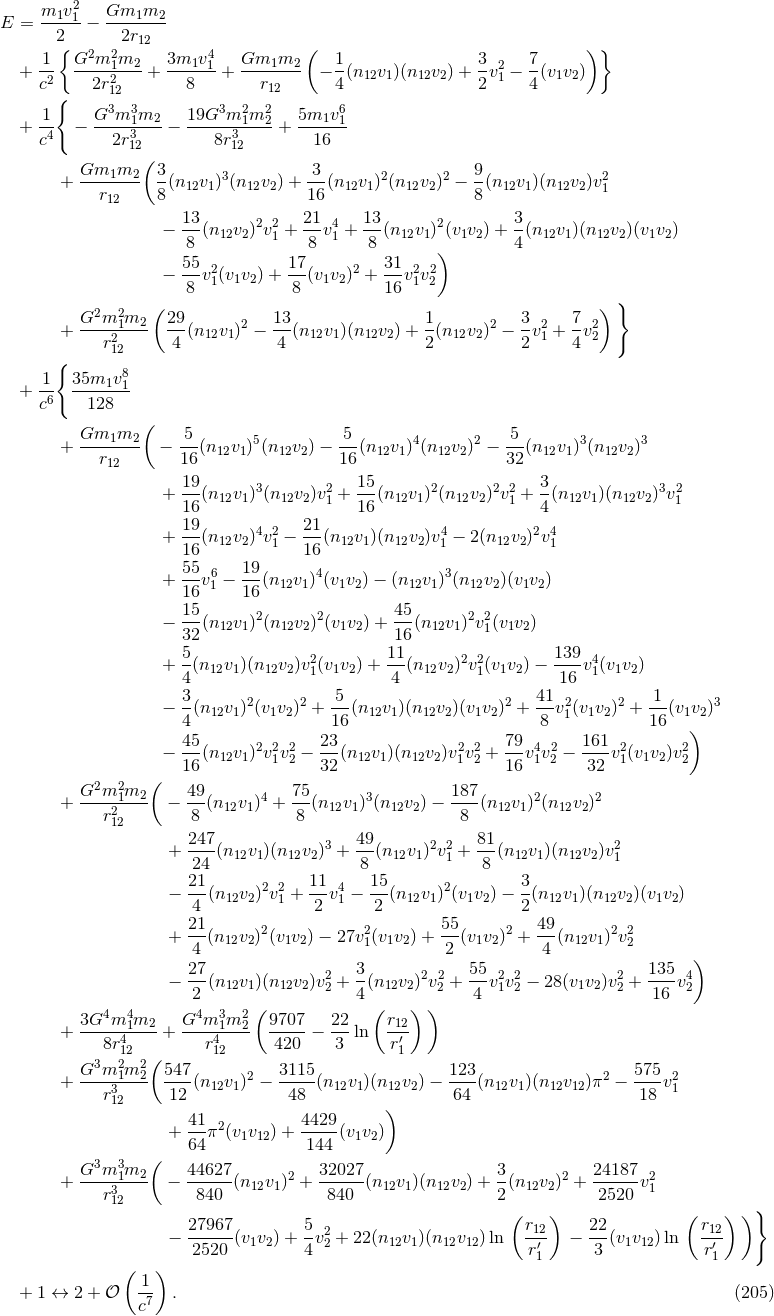
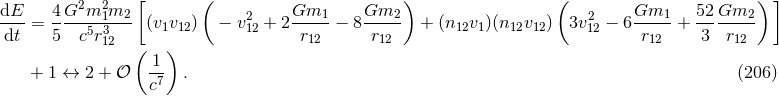
![2 2 [ ] E2.5PN = E + 4G--m-1m2-(n v ) v2 − 2G-(m1-−-m2-)- + 1 ↔ 2, (207 ) 5c5r212 12 1 12 r12](article1685x.gif)
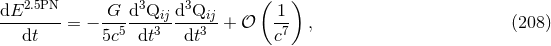
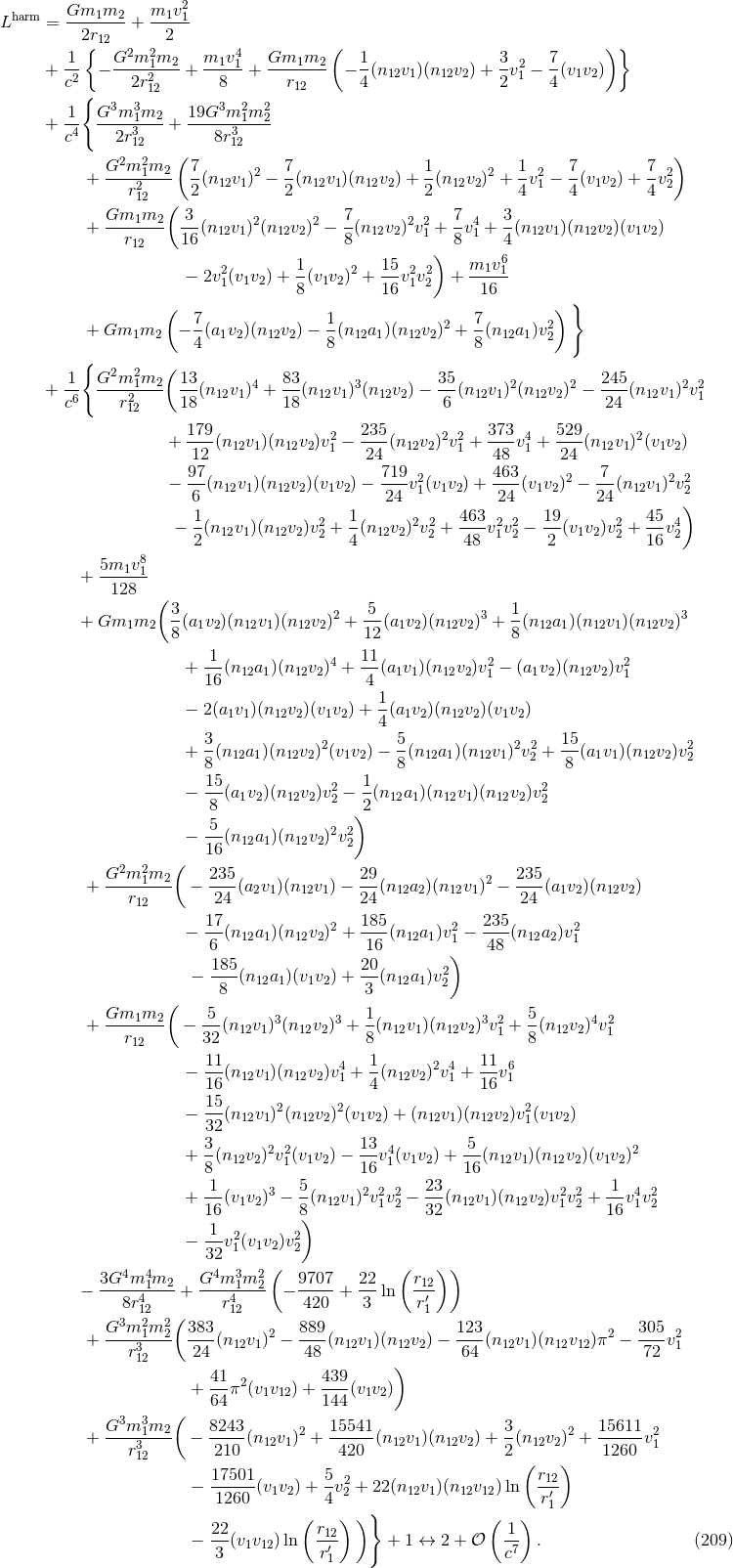
![1 [∂Lharm ∂F 1 ] ( 1 ) δy1 = --- ------- + ----+ -6X1 + 𝒪 -8 (and idem 1 ↔ 2), (210 ) m1 ∂a1 ∂v1 c c](article1700x.gif)
![[ ( ) ( ) ] 22-G3m1m2--- 2 r12 2 r12- F = 3 c6r2 m 1(n12v1 )ln r′ − m 2(n12v2)ln r′ + ⋅⋅⋅, (211 ) 12 1 2](article1712x.gif)
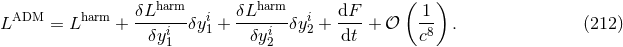
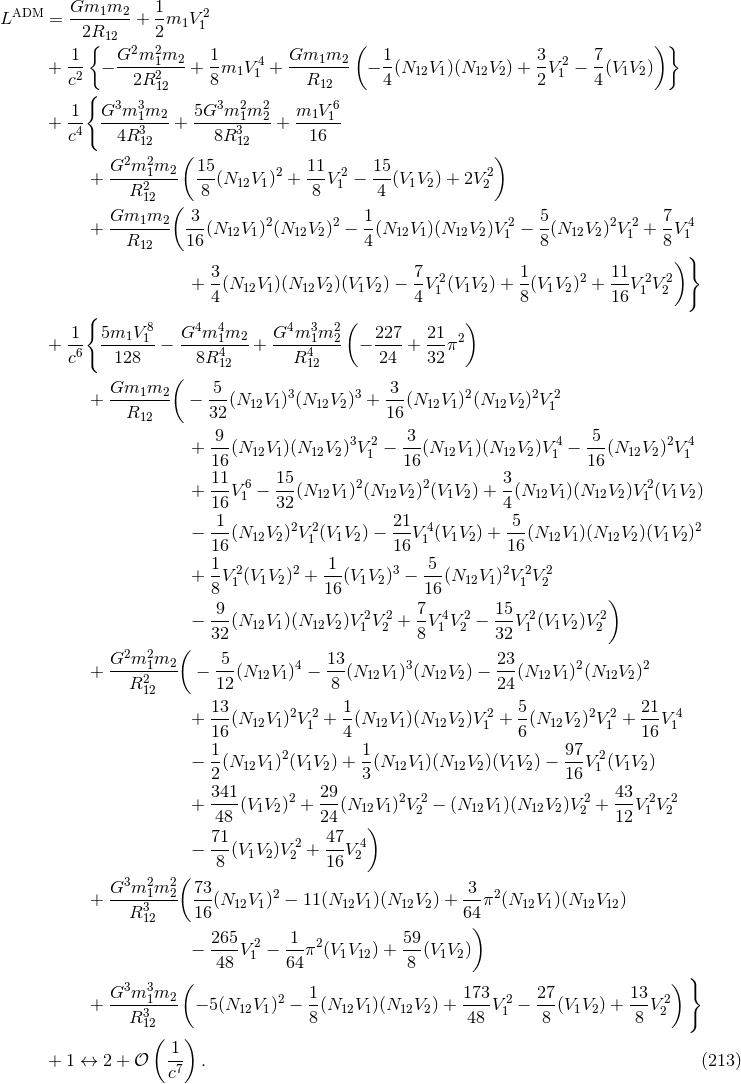


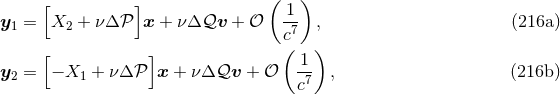
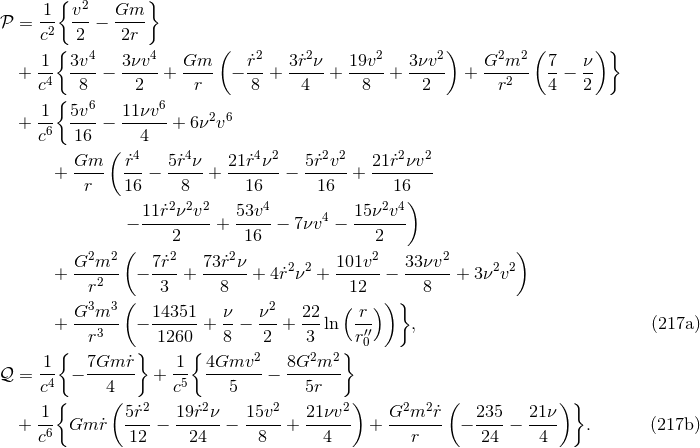

![[( ) ] ( ) dv-= − Gm-- 1 + 𝒜 n + ℬv + 𝒪 1- , (219 ) dt r2 c8](article1761x.gif)
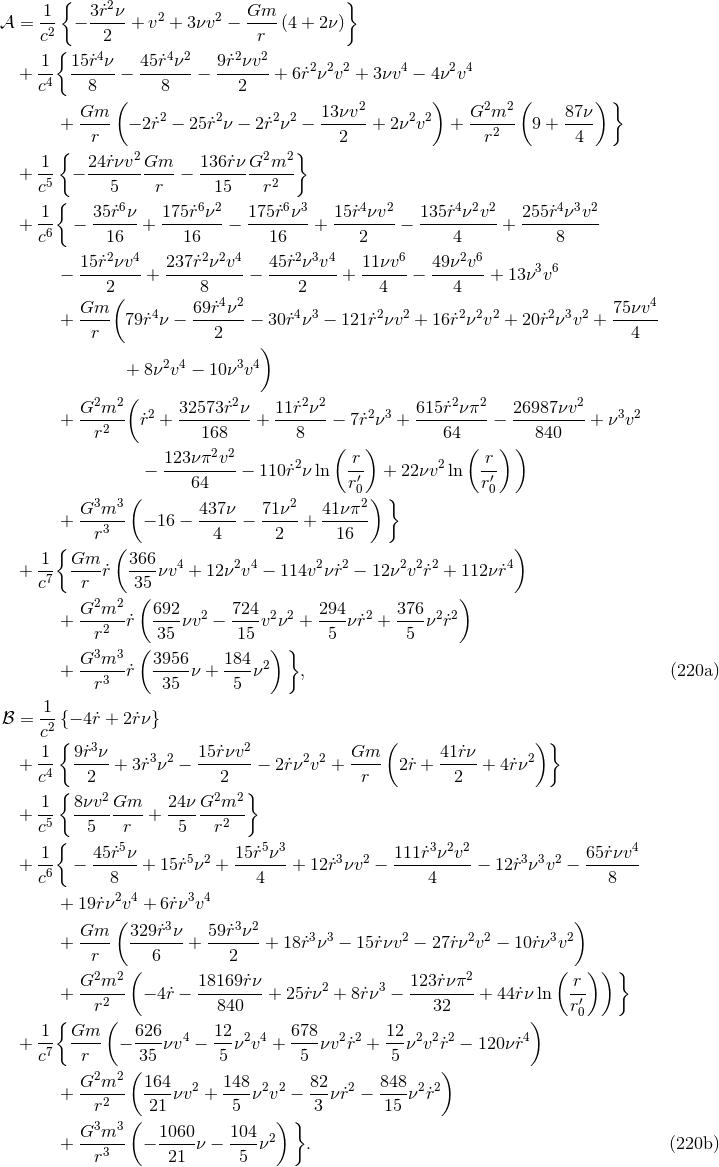

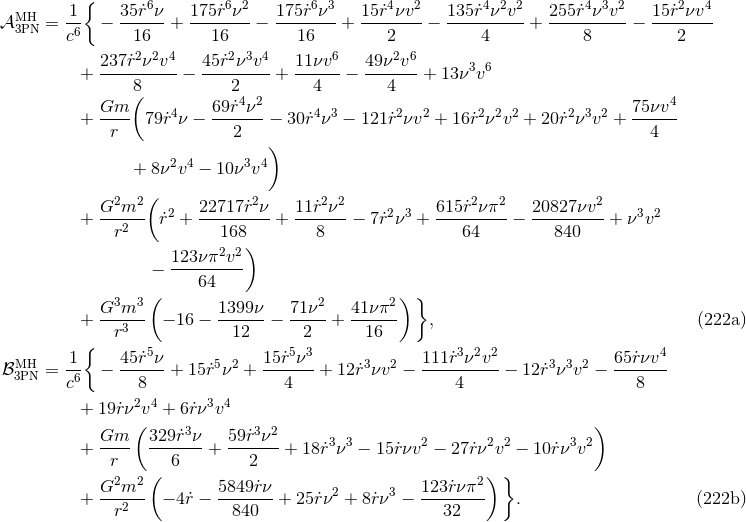
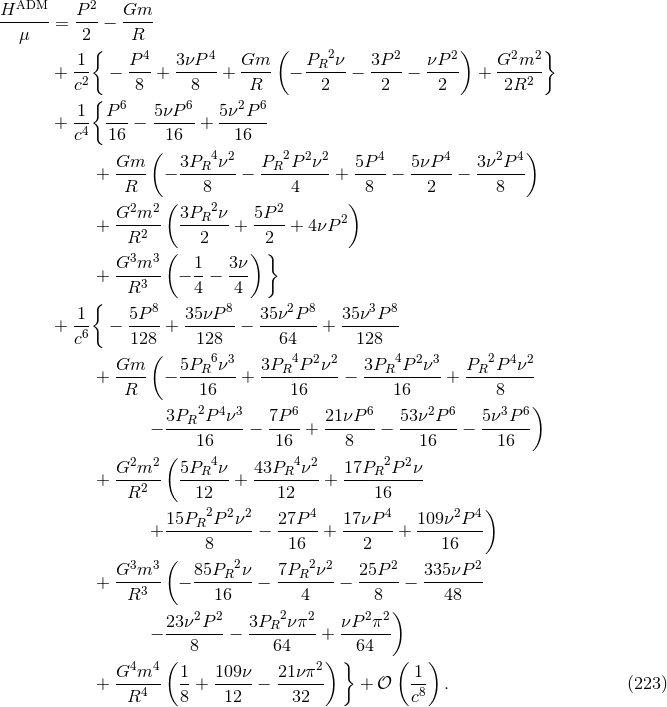
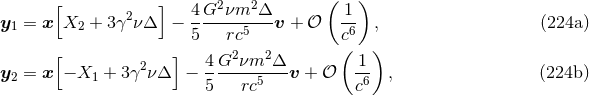

![3 3 [ ( )] ( ) a = − Ω2x − 32-G-m--ν- 1 + γ − 743-− 11ν v + 𝒪 -1 , (226 ) 5 c5r4 336 4 c8](article1795x.gif)