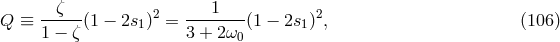5 Strong Gravity and Gravitational Waves: Tests for the 21st Century
5.1 Strong-field systems in general relativity
5.1.1 Defining weak and strong gravity
In the solar system, gravity is weak, in the sense that the Newtonian gravitational potential and related
variables (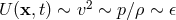 ) are much smaller than unity everywhere. This is the basis for the
post-Newtonian expansion and for the “parametrized post-Newtonian” framework described in Section 3.2.
“Strong-field” systems are those for which the simple 1PN approximation of the PPN framework is no
longer appropriate. This can occur in a number of situations:
) are much smaller than unity everywhere. This is the basis for the
post-Newtonian expansion and for the “parametrized post-Newtonian” framework described in Section 3.2.
“Strong-field” systems are those for which the simple 1PN approximation of the PPN framework is no
longer appropriate. This can occur in a number of situations:
- The system may contain strongly relativistic objects, such as neutron stars or black holes, near
and inside which
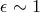 , and the post-Newtonian approximation breaks down. Nevertheless,
under some circumstances, the orbital motion may be such that the interbody potential and
orbital velocities still satisfy
, and the post-Newtonian approximation breaks down. Nevertheless,
under some circumstances, the orbital motion may be such that the interbody potential and
orbital velocities still satisfy 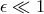 so that a kind of post-Newtonian approximation for
the orbital motion might work; however, the strong-field internal gravity of the bodies could
(especially in alternative theories of gravity) leave imprints on the orbital motion.
so that a kind of post-Newtonian approximation for
the orbital motion might work; however, the strong-field internal gravity of the bodies could
(especially in alternative theories of gravity) leave imprints on the orbital motion.
- The evolution of the system may be affected by the emission of gravitational radiation. The
1PN approximation does not contain the effects of gravitational radiation back-reaction. In the
expression for the metric given in Box 2, radiation back-reaction effects in GR do not occur
until
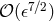 in
in 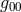 ,
, 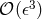 in
in 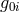 , and
, and 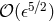 in
in 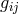 . Consequently, in order to
describe such systems, one must carry out a solution of the equations substantially beyond
1PN order, sufficient to incorporate the leading radiation damping terms at 2.5PN order. In
addition, the PPN metric described in Section 3.2 is valid in the near zone of the system, i.e.,
within one gravitational wavelength of the system’s center of mass. As such it cannot describe
the gravitational waves seen by a detector.
. Consequently, in order to
describe such systems, one must carry out a solution of the equations substantially beyond
1PN order, sufficient to incorporate the leading radiation damping terms at 2.5PN order. In
addition, the PPN metric described in Section 3.2 is valid in the near zone of the system, i.e.,
within one gravitational wavelength of the system’s center of mass. As such it cannot describe
the gravitational waves seen by a detector.
- The system may be highly relativistic in its orbital motion, so that
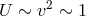 even
for the interbody field and orbital velocity. Systems like this include the late stage of the
inspiral of binary systems of neutron stars or black holes, driven by gravitational radiation
damping, prior to a merger and collapse to a final stationary state. Binary inspiral is one
of the leading candidate sources for detection by the existing LIGO-Virgo network of laser
interferometric gravitational-wave observatories and by a future space-based interferometer. A
proper description of such systems requires not only equations for the motion of the binary
carried to extraordinarily high PN orders (at least 3.5PN), but also requires equations for the
far-zone gravitational waveform measured at the detector, that are equally accurate to high
PN orders beyond the leading “quadrupole” approximation.
even
for the interbody field and orbital velocity. Systems like this include the late stage of the
inspiral of binary systems of neutron stars or black holes, driven by gravitational radiation
damping, prior to a merger and collapse to a final stationary state. Binary inspiral is one
of the leading candidate sources for detection by the existing LIGO-Virgo network of laser
interferometric gravitational-wave observatories and by a future space-based interferometer. A
proper description of such systems requires not only equations for the motion of the binary
carried to extraordinarily high PN orders (at least 3.5PN), but also requires equations for the
far-zone gravitational waveform measured at the detector, that are equally accurate to high
PN orders beyond the leading “quadrupole” approximation.
Of course, some systems cannot be properly described by any post-Newtonian approximation because their behavior is fundamentally controlled by strong gravity. These include the imploding cores of supernovae, the final merger of two compact objects, the quasinormal-mode vibrations of neutron stars and black holes, the structure of rapidly rotating neutron stars, and so on. Phenomena such as these must be analyzed using different techniques. Chief among these is the full solution of Einstein’s equations via numerical methods. This field of “numerical relativity” has become a mature branch of gravitational physics, whose description is beyond the scope of this review (see [247, 176, 35] for reviews). Another is black-hole perturbation theory (see [285*, 219*, 351*, 43] for reviews).
5.1.2 Compact bodies and the strong equivalence principle
When dealing with the motion and gravitational-wave generation by orbiting bodies, one finds a remarkable simplification within GR. As long as the bodies are sufficiently well-separated that one can ignore tidal interactions and other effects that depend upon the finite extent of the bodies (such as their quadrupole and higher multipole moments), then all aspects of their orbital behavior and gravitational-wave generation can be characterized by just two parameters: mass and angular momentum. Whether their internal structure is highly relativistic, as in black holes or neutron stars, or non-relativistic as in the Earth and Sun, only the mass and angular momentum are needed. Furthermore, both quantities are measurable in principle by examining the external gravitational field of the bodies, and make no reference whatsoever to their interiors.
Damour [100*] calls this the “effacement” of the bodies’ internal structure. It is a consequence of the strong equivalence principle (SEP), described in Section 3.1.2.
General relativity satisfies SEP because it contains one and only one gravitational field, the spacetime
metric 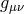 . Consider the motion of a body in a binary system, whose size is small compared to the binary
separation. Surround the body by a region that is large compared to the size of the body, yet small
compared to the separation. Because of the general covariance of the theory, one can choose a freely-falling
coordinate system which comoves with the body, whose spacetime metric takes the Minkowski form at its
outer boundary (ignoring tidal effects generated by the companion). There is thus no evidence of the
presence of the companion body, and the structure of the chosen body can be obtained using the field
equations of GR in this coordinate system. Far from the chosen body, the metric is characterized by the
mass and angular momentum (assuming that one ignores quadrupole and higher multipole
moments of the body) as measured far from the body using orbiting test particles and gyroscopes.
These asymptotically measured quantities are oblivious to the body’s internal structure. A black
hole of mass
. Consider the motion of a body in a binary system, whose size is small compared to the binary
separation. Surround the body by a region that is large compared to the size of the body, yet small
compared to the separation. Because of the general covariance of the theory, one can choose a freely-falling
coordinate system which comoves with the body, whose spacetime metric takes the Minkowski form at its
outer boundary (ignoring tidal effects generated by the companion). There is thus no evidence of the
presence of the companion body, and the structure of the chosen body can be obtained using the field
equations of GR in this coordinate system. Far from the chosen body, the metric is characterized by the
mass and angular momentum (assuming that one ignores quadrupole and higher multipole
moments of the body) as measured far from the body using orbiting test particles and gyroscopes.
These asymptotically measured quantities are oblivious to the body’s internal structure. A black
hole of mass 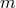 and a planet of mass
and a planet of mass 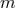 would produce identical spacetimes in this outer
region.
would produce identical spacetimes in this outer
region.
The geometry of this region surrounding the one body must be matched to the geometry provided by
the companion body. Einstein’s equations provide consistency conditions for this matching
that yield constraints on the motion of the bodies. These are the equations of motion. As a
result, the motion of two planets of mass and angular momentum 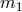 ,
, 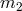 ,
, 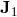 , and
, and 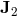 is
identical to that of two black holes of the same mass and angular momentum (again, ignoring tidal
effects).
is
identical to that of two black holes of the same mass and angular momentum (again, ignoring tidal
effects).
This effacement does not occur in an alternative gravitional theory like scalar–tensor gravity. There, in
addition to the spacetime metric, a scalar field 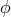 is generated by the masses of the bodies, and
controls the local value of the gravitational coupling constant (i.e.,
is generated by the masses of the bodies, and
controls the local value of the gravitational coupling constant (i.e., 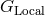 is a function of
is a function of 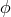 ).
Now, in the local frame surrounding one of the bodies in our binary system, while the metric
can still be made Minkowskian far away, the scalar field will take on a value
).
Now, in the local frame surrounding one of the bodies in our binary system, while the metric
can still be made Minkowskian far away, the scalar field will take on a value 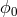 determined
by the companion body. This can affect the value of
determined
by the companion body. This can affect the value of 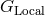 inside the chosen body, alter
its internal structure (specifically its gravitational binding energy) and hence alter its mass.
Effectively, each body can be characterized by several mass functions
inside the chosen body, alter
its internal structure (specifically its gravitational binding energy) and hence alter its mass.
Effectively, each body can be characterized by several mass functions 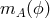 , which depend on
the value of the scalar field at its location, and several distinct masses come into play, such as
inertial mass, gravitational mass, “radiation” mass, etc. The precise nature of the functions
will depend on the body, specifically on its gravitational binding energy, and as a result, the
motion and gravitational radiation may depend on the internal structure of each body. For
compact bodies such as neutron stars and black holes these internal structure effects could be
large; for example, the gravitational binding energy of a neutron star can be 10 – 20 percent of
its total mass. At 1PN order, the leading manifestation of this phenomenon is the Nordtvedt
effect.
, which depend on
the value of the scalar field at its location, and several distinct masses come into play, such as
inertial mass, gravitational mass, “radiation” mass, etc. The precise nature of the functions
will depend on the body, specifically on its gravitational binding energy, and as a result, the
motion and gravitational radiation may depend on the internal structure of each body. For
compact bodies such as neutron stars and black holes these internal structure effects could be
large; for example, the gravitational binding energy of a neutron star can be 10 – 20 percent of
its total mass. At 1PN order, the leading manifestation of this phenomenon is the Nordtvedt
effect.
This is how the study of orbiting systems containing compact objects provides strong-field tests of GR. Even though the strong-field nature of the bodies is effaced in GR, it is not in other theories, thus any result in agreement with the predictions of GR constitutes a kind of “null” test of strong-field gravity.
5.2 Motion and gravitational radiation in general relativity: A history
At the most primitive level, the problem of motion in GR is relatively
straightforward, and was an integral part of the theory as proposed by
Einstein3.
The first attempts to treat the motion of multiple bodies, each with a finite mass, were made in the period
1916 – 1917 by Lorentz and Droste and by de Sitter [260, 124]. They derived the metric and equations of
motion for a system of 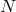 bodies, in what today would be called the first post-Newtonian approximation
of GR (de Sitter’s equations turned out to contain some important errors). In 1916, Einstein took the first
crack at a study of gravitational radiation, deriving the energy emitted by a body such as a
rotating rod or dumbbell, held together by non-gravitational forces [143, 144]. He made some
unjustified assumptions as well as a trivial numerical error (later corrected by Eddington [141]),
but the underlying conclusion that dynamical systems would radiate gravitational waves was
correct.
bodies, in what today would be called the first post-Newtonian approximation
of GR (de Sitter’s equations turned out to contain some important errors). In 1916, Einstein took the first
crack at a study of gravitational radiation, deriving the energy emitted by a body such as a
rotating rod or dumbbell, held together by non-gravitational forces [143, 144]. He made some
unjustified assumptions as well as a trivial numerical error (later corrected by Eddington [141]),
but the underlying conclusion that dynamical systems would radiate gravitational waves was
correct.
The next significant advance in the problem of motion came 20 years later. In 1938, Einstein, Infeld and
Hoffman published the now legendary “EIH” paper, a calculation of the 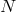 -body equations of motion
using only the vacuum field equations of GR [145]. They treated each body in the system as a spherically
symmetric object whose nearby vacuum exterior geometry approximated that of the Schwarzschild metric of
a static spherical star. They then solved the vacuum field equations for the metric between
each body in the system in a weak field, slow-motion approximation. Then, using a primitive
version of what today would be called “matched asymptotic expansions” they showed that, in
order for the nearby metric of each body to match smoothly to the interbody metric at each
order in the expansion, certain conditions on the motion of each body had to be met. Together,
these conditions turned out to be equivalent to the Droste–Lorentz
-body equations of motion
using only the vacuum field equations of GR [145]. They treated each body in the system as a spherically
symmetric object whose nearby vacuum exterior geometry approximated that of the Schwarzschild metric of
a static spherical star. They then solved the vacuum field equations for the metric between
each body in the system in a weak field, slow-motion approximation. Then, using a primitive
version of what today would be called “matched asymptotic expansions” they showed that, in
order for the nearby metric of each body to match smoothly to the interbody metric at each
order in the expansion, certain conditions on the motion of each body had to be met. Together,
these conditions turned out to be equivalent to the Droste–Lorentz 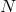 -body equations of
motion. The internal structure of each body was irrelevant, apart from the requirement that its
nearby field be approximately spherically symmetric, a clear illustration of the “effacement”
principle.
-body equations of
motion. The internal structure of each body was irrelevant, apart from the requirement that its
nearby field be approximately spherically symmetric, a clear illustration of the “effacement”
principle.
Around the same time, there occurred an unusual detour in the problem of motion. Using equations of motion based on de Sitter’s paper, specialized to two bodies, Levi-Civita [249] showed that the center of mass of a binary star system would suffer an acceleration in the direction of the pericenter of the orbit, in an amount proportional to the difference between the two masses, and to the eccentricity of the orbit. Such an effect would be a violation of the conservation of momentum for isolated systems caused by relativistic gravitational effects. Levi-Civita even went so far as to suggest looking for this effect in selected nearby close binary star systems. However, Eddington and Clark [140] quickly pointed out that Levi-Civita had based his calculations on de Sitter’s flawed work; when correct two-body equations of motion were used, the effect vanished, and momentum conservation was upheld. Robertson confirmed this using the EIH equations of motion [341]. Such an effect can only occur in theories of gravity that lack the appropriate conservation laws (Section 4.4.3).
There was ongoing confusion over whether gravitational waves are real or are artifacts of general covariance. Although Eddington was credited with making the unfortunate remark that gravitational waves propagate “with the speed of thought”, he did clearly elucidate the difference between the physical, coordinate independent modes and modes that were purely coordinate artifacts [141]. But in 1936, in a paper submitted to the Physical Review, Einstein and Rosen claimed to prove that gravitational waves could not exist; the anonymous referee of their paper found that they had made an error. Upset that the journal had sent his paper to a referee (a newly instituted practice), Einstein refused to publish there again. A corrected paper by Einstein and Rosen showing that gravitational waves did exist – cylindrical waves in this case – was published elsewhere [146]. Fifty years later it was revealed that the anonymous referee was H. P. Robertson [213].
Roughly 20 more years would pass before another major attack on the problem of motion. Fock in the USSR and Chandrasekhar in the US independently developed and systematized the post-Newtonian approximation in a form that laid the foundation for modern post-Newtonian theory [160, 77]. They developed a full post-Newtonian hydrodynamics, with the ability to treat realistic, self-gravitating bodies of fluid, such as stars and planets. In the suitable limit of “point” particles, or bodies whose size is small enough compared to the interbody separations that finite-size effects such as spin and tidal interactions can be ignored, their equations of motion could be shown to be equivalent to the EIH and the Droste-Lorentz equations of motion.
The next important period in the history of the problem of motion was 1974 – 1979, initiated by the 1974 discovery of the binary pulsar PSR 1913+16 by Hulse and Taylor [196]. Around the same time there occurred the first serious attempt to calculate the head-on collision of two black holes using purely numerical solutions of Einstein’s equations, by Smarr and collaborators [368].
The binary pulsar consists of two neutron stars, one an active pulsar detectable by radio telescopes, the other very likely an old, inactive pulsar (Section 6.1). Each neutron star has a mass of around 1.4 solar masses. The orbit of the system was seen immediately to be quite relativistic, with an orbital period of only eight hours, and a mean orbital speed of 200 km/s, some four times faster than Mercury in its orbit. Within weeks of its discovery, numerous authors pointed out that PSR 1913+16 would be an important new testing ground for GR. In particular, it could provide for the first time a test of the effects of the emission of gravitational radiation on the orbit of the system.
However, the discovery revealed an ugly truth about the “problem of motion”. As Ehlers et al. pointed out in an influential 1976 paper [142], the general relativistic problem of motion and radiation was full of holes large enough to drive trucks through. They pointed out that most treatments of the problem used “delta functions” as a way to approximate the bodies in the system as point masses. As a consequence, the “self-field”, the gravitational field of the body evaluated at its own location, becomes infinite. While this is not a major issue in Newtonian gravity or classical electrodynamics, the non-linear nature of GR requires that this infinite self-field contribute to gravity. In the past, such infinities had been simply swept under the rug. Similarly, because gravitational energy itself produces gravity it thus acts as a source throughout spacetime. This means that, when calculating radiative fields, integrals for the multipole moments of the source that are so useful in treating radiation begin to diverge. These divergent integrals had also been routinely swept under the rug. Ehlers et al. further pointed out that the true boundary condition for any problem involving radiation by an isolated system should be one of “no incoming radiation” from the past. Connecting this boundary condition with the routine use of retarded solutions of wave equations was not a trivial matter in GR. Finally, they pointed out that there was no evidence that the post-Newtonian approximation, so central to the problem of motion, was a convergent or even asymptotic sequence. Nor had the approximation been carried out to high enough order to make credible error estimates.
During this time, some authors even argued that the “quadrupole formula” for the gravitational energy emitted by a system (see below), while correct for a rotating dumbell as calculated by Einstein, was actually wrong for a binary system moving under its own gravity. The discovery in 1979 that the rate of decay of the orbit of the binary pulsar was in agreement with the standard quadrupole formula made some of these arguments moot. Yet the question raised by Ehlers et al. was still relevant: is the quadrupole formula for binary systems an actual prediction of GR?
Motivated by the Ehlers et al. critique, numerous workers began to address the holes in the problem of motion, and by the late 1990s most of the criticisms had been answered, particularly those related to divergences. For a detailed history of the ups and downs of the subject of motion and gravitational waves, see [214].
The problem of motion and radiation in GR has received renewed interest since 1990, with proposals for construction of large-scale laser interferometric gravitational-wave observatories. These proposals culminated in the construction and operation of LIGO in the US, VIRGO and GEO600 in Europe, and TAMA300 in Japan, the construction of an underground observatory KAGRA in Japan, and the possible construction of a version of LIGO in India. Advanced versions of LIGO and VIRGO are expected to be online and detecting gravitational waves around 2016. An interferometer in space has recently been selected by the European Space Agency for a launch in the 2034 time frame.
A leading candidate source of detectable waves is the inspiral, driven by gravitational radiation damping, of a binary system of compact objects (neutron stars or black holes) (for a review of sources of gravitational waves, see [352]). The analysis of signals from such systems will require theoretical predictions from GR that are extremely accurate, well beyond the leading-order prediction of Newtonian or even post-Newtonian gravity for the orbits, and well beyond the leading-order formulae for gravitational waves.
This presented a major theoretical challenge: to calculate the motion and radiation of systems of compact objects to very high PN order, a formidable algebraic task, while addressing the issues of principle raised by Ehlers et al., sufficiently well to ensure that the results were physically meaningful. This challenge has been largely met, so that we may soon see a remarkable convergence between observational data and accurate predictions of gravitational theory that could provide new, strong-field tests of GR.
5.3 Compact binary systems in general relativity
5.3.1 Einstein’s equations in “relaxed” form
Here we give a brief overview of the modern approach to the problem of motion and gravitational radiation in GR. For a full pedagogical treatment, see [324*].
The Einstein equations 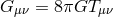 are elegant and deceptively simple, showing geometry (in the
form of the Einstein tensor
are elegant and deceptively simple, showing geometry (in the
form of the Einstein tensor 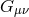 , which is a function of spacetime curvature) being generated by matter
(in the form of the material stress-energy tensor
, which is a function of spacetime curvature) being generated by matter
(in the form of the material stress-energy tensor 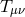 ). However, this is not the most useful form for
actual calculations. For post-Newtonian calculations, a far more useful form is the so-called
“relaxed” Einstein equations, which form the basis of the program of approximating solutions
of Einstein’s equations known as post-Minkowskian theory and post-Newtonian theory. The
starting point is the so-called “gothic inverse metric”, defined by
). However, this is not the most useful form for
actual calculations. For post-Newtonian calculations, a far more useful form is the so-called
“relaxed” Einstein equations, which form the basis of the program of approximating solutions
of Einstein’s equations known as post-Minkowskian theory and post-Newtonian theory. The
starting point is the so-called “gothic inverse metric”, defined by 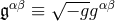 , where
, where 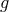 is the determinant of
is the determinant of 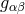 . One then defines the gravitational potential
. One then defines the gravitational potential 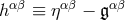 .
After imposing the de Donder or harmonic gauge condition
.
After imposing the de Donder or harmonic gauge condition 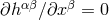 (summation on
repeated indices is assumed), one can recast the exact Einstein field equations into the form
(summation on
repeated indices is assumed), one can recast the exact Einstein field equations into the form
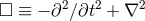 is the flat-spacetime wave operator. This form of Einstein’s equations bears a
striking similarity to Maxwell’s equations for the vector potential
is the flat-spacetime wave operator. This form of Einstein’s equations bears a
striking similarity to Maxwell’s equations for the vector potential 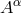 in Lorentz gauge:
in Lorentz gauge: 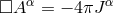 ,
,
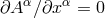 . There is a key difference, however: The source on the right hand side of Eq. (77*) is given
by the “effective” stress-energy pseudotensor
where
. There is a key difference, however: The source on the right hand side of Eq. (77*) is given
by the “effective” stress-energy pseudotensor
where 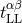 and
and 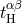 are the Landau–Lifshitz pseudotensor and a harmonic pseudotensor, given by terms
quadratic (and higher) in
are the Landau–Lifshitz pseudotensor and a harmonic pseudotensor, given by terms
quadratic (and higher) in 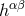 and its derivatives (see [324*], Eqs. (6.5, 6.52, 6.53) for explicit formulae).
In GR, the gravitational field itself generates gravity, a reflection of the nonlinearity of Einstein’s equations,
and in contrast to the linearity of Maxwell’s equations.
and its derivatives (see [324*], Eqs. (6.5, 6.52, 6.53) for explicit formulae).
In GR, the gravitational field itself generates gravity, a reflection of the nonlinearity of Einstein’s equations,
and in contrast to the linearity of Maxwell’s equations.
Eq. (77*) is exact, and depends only on the assumption that the relevant parts of spacetime can be
covered by harmonic coordinates. It is called “relaxed” because it can be solved formally as a functional
of source variables without specifying the motion of the source, in the form (with  )
)
 of the field point
of the field point 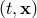 . The motion of
the source is then determined either by the equation
. The motion of
the source is then determined either by the equation 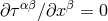 (which follows from the harmonic
gauge condition), or from the usual covariant equation of motion
(which follows from the harmonic
gauge condition), or from the usual covariant equation of motion 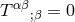 , where the subscript
, where the subscript 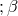 denotes a covariant divergence. This formal solution can then be iterated in a slow motion
(
denotes a covariant divergence. This formal solution can then be iterated in a slow motion
(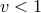 ) weak-field (
) weak-field (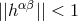 ) approximation. One begins by substituting
) approximation. One begins by substituting 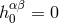 into
the source
into
the source 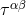 in Eq. (79*), and solving for the first iterate
in Eq. (79*), and solving for the first iterate 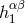 , and then repeating the
procedure sufficiently many times to achieve a solution of the desired accuracy. For example, to
obtain the 1PN equations of motion, two iterations are needed (i.e.,
, and then repeating the
procedure sufficiently many times to achieve a solution of the desired accuracy. For example, to
obtain the 1PN equations of motion, two iterations are needed (i.e., 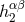 must be calculated);
likewise, to obtain the leading gravitational waveform for a binary system, two iterations are
needed.
must be calculated);
likewise, to obtain the leading gravitational waveform for a binary system, two iterations are
needed.
At the same time, just as in electromagnetism, the formal integral (79*) must be handled differently,
depending on whether the field point is in the far zone or the near zone. For field points in the far zone or
radiation zone, 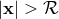 , where
, where 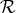 is a distance of the order of a gravitational wavelength, the field
can be expanded in inverse powers of
is a distance of the order of a gravitational wavelength, the field
can be expanded in inverse powers of 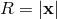 in a multipole expansion, evaluated at the
“retarded time”
in a multipole expansion, evaluated at the
“retarded time” 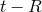 . The leading term in
. The leading term in 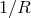 is the gravitational waveform. For field
points in the near zone or induction zone,
is the gravitational waveform. For field
points in the near zone or induction zone, 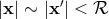 , the field is expanded in powers of
, the field is expanded in powers of
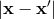 about the local time
about the local time 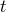 , yielding instantaneous potentials that go into the equations of
motion.
, yielding instantaneous potentials that go into the equations of
motion.
However, because the source 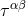 contains
contains 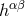 itself, it is not confined to a compact region,
but extends over all spacetime. As a result, there is a danger that the integrals involved in
the various expansions will diverge or be ill-defined. This consequence of the non-linearity of
Einstein’s equations has bedeviled the subject of gravitational radiation for decades. Numerous
approaches have been developed to try to handle this difficulty. The post-Minkowskian method
of Blanchet, Damour, and Iyer [52, 53, 54, 108, 55, 50] solves Einstein’s equations by two
different techniques, one in the near zone and one in the far zone, and uses the method of singular
asymptotic matching to join the solutions in an overlap region. The method provides a natural
“regularization” technique to control potentially divergent integrals (see [51*] for a thorough review). The
“Direct Integration of the Relaxed Einstein Equations” (DIRE) approach of Will, Wiseman, and
Pati [432, 316, 317] retains Eq. (79*) as the global solution, but splits the integration into one over
the near zone and another over the far zone, and uses different integration variables to carry
out the explicit integrals over the two zones. In the DIRE method, all integrals are finite and
convergent. Itoh and Futamase used an extension of the Einstein–Infeld–Hoffman matching
approach combined with a specific method for taking a point-particle limit [198], while Damour,
Jaranowski, and Schäfer pioneered an ADM Hamiltonian approach that focuses on the equations of
motion [206, 207, 109, 110, 111].
itself, it is not confined to a compact region,
but extends over all spacetime. As a result, there is a danger that the integrals involved in
the various expansions will diverge or be ill-defined. This consequence of the non-linearity of
Einstein’s equations has bedeviled the subject of gravitational radiation for decades. Numerous
approaches have been developed to try to handle this difficulty. The post-Minkowskian method
of Blanchet, Damour, and Iyer [52, 53, 54, 108, 55, 50] solves Einstein’s equations by two
different techniques, one in the near zone and one in the far zone, and uses the method of singular
asymptotic matching to join the solutions in an overlap region. The method provides a natural
“regularization” technique to control potentially divergent integrals (see [51*] for a thorough review). The
“Direct Integration of the Relaxed Einstein Equations” (DIRE) approach of Will, Wiseman, and
Pati [432, 316, 317] retains Eq. (79*) as the global solution, but splits the integration into one over
the near zone and another over the far zone, and uses different integration variables to carry
out the explicit integrals over the two zones. In the DIRE method, all integrals are finite and
convergent. Itoh and Futamase used an extension of the Einstein–Infeld–Hoffman matching
approach combined with a specific method for taking a point-particle limit [198], while Damour,
Jaranowski, and Schäfer pioneered an ADM Hamiltonian approach that focuses on the equations of
motion [206, 207, 109, 110, 111].
These methods assume from the outset that gravity is sufficiently weak that 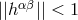 and harmonic
coordinates exists everywhere, including inside the bodies. Thus, in order to apply the results to cases where
the bodies may be neutron stars or black holes, one relies upon the SEP to argue that, if tidal forces are
ignored, and equations are expressed in terms of masses and spins, one can simply extrapolate the results
unchanged to the situation where the bodies are ultrarelativistic. While no general proof of this
exists, it has been shown to be valid in specific circumstances, such as through 2PN order in
the equations of motion [178, 290], and for black holes moving in a Newtonian background
field [100].
and harmonic
coordinates exists everywhere, including inside the bodies. Thus, in order to apply the results to cases where
the bodies may be neutron stars or black holes, one relies upon the SEP to argue that, if tidal forces are
ignored, and equations are expressed in terms of masses and spins, one can simply extrapolate the results
unchanged to the situation where the bodies are ultrarelativistic. While no general proof of this
exists, it has been shown to be valid in specific circumstances, such as through 2PN order in
the equations of motion [178, 290], and for black holes moving in a Newtonian background
field [100].
Methods such as these have resolved most of the issues that led to criticism of the foundations of gravitational radiation theory during the 1970s.
5.3.2 Equations of motion and gravitational waveform
Among the results of these approaches are formulae for the equations of motion and gravitational waveform of binary systems of compact objects, carried out to high orders in a PN expansion. For a review of the latest results of high-order PN calculations, see [51*]. Here we shall only state the key formulae that will be needed for this review. For example, the relative two-body equation of motion has the form
where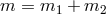 is the total mass,
is the total mass, 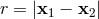 ,
, 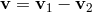 , and
, and 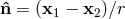 . The
notation
. The
notation 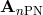 indicates that the term is
indicates that the term is 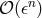 relative to the Newtonian term
relative to the Newtonian term 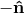 (recall that
(recall that
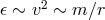 ). Explicit and unambiguous formulae for non-spinning bodies through 3.5PN order have
been calculated by various authors [51*]. Here we quote only the 1PN corrections and the leading
radiation-reaction terms at 2.5PN order:
where
). Explicit and unambiguous formulae for non-spinning bodies through 3.5PN order have
been calculated by various authors [51*]. Here we quote only the 1PN corrections and the leading
radiation-reaction terms at 2.5PN order:
where 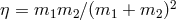 . The radiation-reaction acceleration is expressed in the so-called
Damour–Deruelle gauge. These terms are sufficient to analyze the orbit and evolution of the binary pulsar
(see Section 6.1). For example, the 1PN terms are responsible for the periastron advance of an eccentric
orbit, given by
where
. The radiation-reaction acceleration is expressed in the so-called
Damour–Deruelle gauge. These terms are sufficient to analyze the orbit and evolution of the binary pulsar
(see Section 6.1). For example, the 1PN terms are responsible for the periastron advance of an eccentric
orbit, given by
where  and
and  are the semi-major axis and eccentricity of the orbit, respectively, and
are the semi-major axis and eccentricity of the orbit, respectively, and 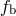 is the orbital
frequency, given to the needed order by Kepler’s third law
is the orbital
frequency, given to the needed order by Kepler’s third law 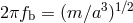 .
.
Another product is a formula for the gravitational field far from the system, written schematically in the form
where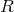 is the distance from the source, and the variables are to be evaluated at retarded time
is the distance from the source, and the variables are to be evaluated at retarded time 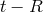 .
The leading term is the so-called quadrupole formula
where
.
The leading term is the so-called quadrupole formula
where 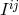 is the quadrupole moment of the source, and overdots denote time derivatives. For a binary
system this leads to
For binary systems, explicit formulae for the waveform through 3.5PN order have been derived (see [56] for
a ready-to-use presentation of the waveform to 2PN order for circular orbits; see [51*] for a full
review).
is the quadrupole moment of the source, and overdots denote time derivatives. For a binary
system this leads to
For binary systems, explicit formulae for the waveform through 3.5PN order have been derived (see [56] for
a ready-to-use presentation of the waveform to 2PN order for circular orbits; see [51*] for a full
review).
Given the gravitational waveform, one can compute the rate at which energy is carried off by the
radiation (schematically 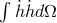 , the gravitational analog of the Poynting flux). The lowest-order
quadrupole formula leads to the gravitational wave energy flux
, the gravitational analog of the Poynting flux). The lowest-order
quadrupole formula leads to the gravitational wave energy flux
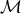 is the so-called “chirp” mass, given by
is the so-called “chirp” mass, given by 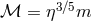 . Notice that by making precise
measurements of the phase
. Notice that by making precise
measurements of the phase 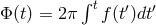 of either the orbit or the gravitational waves (for which
of either the orbit or the gravitational waves (for which
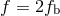 for the dominant component) as a function of the frequency, one in effect measures the “chirp”
mass of the system.
for the dominant component) as a function of the frequency, one in effect measures the “chirp”
mass of the system.
These formalisms have also been generalized to include the leading effects of spin-orbit and spin-spin coupling between the bodies as well as many next-to-leading-order corrections [51*].
Another approach to gravitational radiation is applicable to the special limit in which one mass is much
smaller than the other. This is the method of black hole perturbation theory. One begins with
an exact background spacetime of a black hole, either the non-rotating Schwarzschild or the
rotating Kerr solution, and perturbs it according to 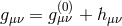 . The particle moves on
a geodesic of the background spacetime, and a suitably defined source stress-energy tensor
for the particle acts as a source for the gravitational perturbation and wave field
. The particle moves on
a geodesic of the background spacetime, and a suitably defined source stress-energy tensor
for the particle acts as a source for the gravitational perturbation and wave field 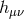 . This
method provides numerical results that are exact in
. This
method provides numerical results that are exact in  , as well as analytical results expressed as
series in powers of
, as well as analytical results expressed as
series in powers of  , both for non-rotating and for rotating black holes. For non-rotating
holes, the analytical expansions have been carried to the impressive level of 22PN order, or
, both for non-rotating and for rotating black holes. For non-rotating
holes, the analytical expansions have been carried to the impressive level of 22PN order, or
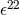 beyond the quadrupole approximation [168], and for rotating Kerr black holes, to 20PN
order [356]. All results of black hole perturbation agree precisely with the
beyond the quadrupole approximation [168], and for rotating Kerr black holes, to 20PN
order [356]. All results of black hole perturbation agree precisely with the 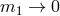 limit of the PN
results, up to the highest PN order where they can be compared (for reviews of earlier work
see [285, 219, 351]).
limit of the PN
results, up to the highest PN order where they can be compared (for reviews of earlier work
see [285, 219, 351]).
5.4 Compact binary systems in scalar–tensor theories
Because of the recent resurgence of interest in scalar–tensor theories of gravity, motivated in
part by string theory and 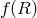 theories, considerable work has been done to analyze the
motion and gravitational radiation from systems of compact objects in this class of theories.
In earlier work, Eardley [139] was the first to point out the existence of dipole gravitational
radiation from self-gravitating bodies in Brans–Dicke theory, and Will [415] worked out the
lowest-order monopole, dipole and quadrupole radiation flux in general scalar–tensor theories
(as well as in a number of alternative theories) for bodies with weak self-gravity. Using the
approach pioneered by Eardley [139] for incorporating strongly self-gravitating bodies into
scalar–tensor calculations, Will and Zaglauer [434*] calculated the 1PN equations of motion along
with the monopole-quadrupole and dipole energy flux for compact binary systems; Alsing et
al. [7] extended these results to the case of Brans–Dicke theory with a massive scalar field.
However, the expressions for the energy flux in those works were incomplete, because they failed to
include some important post-Newtonian corrections in the scalar part of the radiation that
actually contribute at the same order as the quadrupole contributions from the tensor part.
Damour and Esposito-Farèse [105] obtained the correct monopole-quadrupole and dipole
energy flux, working in the Einstein-frame representation of scalar–tensor theories, and gave
partial results for the equations of motion to 2PN order. Mirshekari and Will [286] obtained
the complete compact-binary equations of motion in general scalar–tensor theories through
2.5PN order, and obtained the energy loss rate in complete agreement with the flux result from
Damour and Esposito-Farèse. Lang [241] obtained the tensor gravitational-wave signal to 2PN
order.
theories, considerable work has been done to analyze the
motion and gravitational radiation from systems of compact objects in this class of theories.
In earlier work, Eardley [139] was the first to point out the existence of dipole gravitational
radiation from self-gravitating bodies in Brans–Dicke theory, and Will [415] worked out the
lowest-order monopole, dipole and quadrupole radiation flux in general scalar–tensor theories
(as well as in a number of alternative theories) for bodies with weak self-gravity. Using the
approach pioneered by Eardley [139] for incorporating strongly self-gravitating bodies into
scalar–tensor calculations, Will and Zaglauer [434*] calculated the 1PN equations of motion along
with the monopole-quadrupole and dipole energy flux for compact binary systems; Alsing et
al. [7] extended these results to the case of Brans–Dicke theory with a massive scalar field.
However, the expressions for the energy flux in those works were incomplete, because they failed to
include some important post-Newtonian corrections in the scalar part of the radiation that
actually contribute at the same order as the quadrupole contributions from the tensor part.
Damour and Esposito-Farèse [105] obtained the correct monopole-quadrupole and dipole
energy flux, working in the Einstein-frame representation of scalar–tensor theories, and gave
partial results for the equations of motion to 2PN order. Mirshekari and Will [286] obtained
the complete compact-binary equations of motion in general scalar–tensor theories through
2.5PN order, and obtained the energy loss rate in complete agreement with the flux result from
Damour and Esposito-Farèse. Lang [241] obtained the tensor gravitational-wave signal to 2PN
order.
Notwithstanding the very tight bound on the scalar–tensor coupling parameter 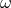 from Cassini
measurements in the solar system, this effort is motivated by a desire to test this theory in strong-field
situations, whether by binary pulsar observations, or by measurements of gravitational radiation from
compact binary inspiral. Here we summarize the key results in a manner that parallels the results for
GR.
from Cassini
measurements in the solar system, this effort is motivated by a desire to test this theory in strong-field
situations, whether by binary pulsar observations, or by measurements of gravitational radiation from
compact binary inspiral. Here we summarize the key results in a manner that parallels the results for
GR.
5.4.1 Scalar–tensor equations in “relaxed” form
The field equations of scalar–tensor theory can be cast in a form similar to the “relaxed” equations of
GR. Here one works in terms of an auxiliary metric 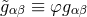 , where
, where 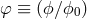 and
and
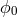 is the asymptotic value of the scalar field, and defines the auxiliary gothic inverse metric
is the asymptotic value of the scalar field, and defines the auxiliary gothic inverse metric
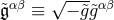 , and the auxiliary tensor gravitational potential
, and the auxiliary tensor gravitational potential 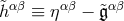 , along
with the harmonic gauge condition
, along
with the harmonic gauge condition 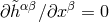 . The field equations then take the form
. The field equations then take the form
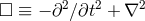 is again the flat-spacetime wave operator, and where
where
is again the flat-spacetime wave operator, and where
where 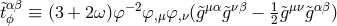 is a scalar stress-energy tensor, and where
is a scalar stress-energy tensor, and where 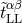 and
and
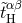 have exactly the same forms, when written in terms of
have exactly the same forms, when written in terms of  , as their counterparts in GR do in terms
of
, as their counterparts in GR do in terms
of 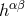 . Note that this is equivalent to formulating the relaxed equations of scalar–tensor theory in the
Einstein conformal frame. The field equation for the scalar field can be written in the form
. Note that this is equivalent to formulating the relaxed equations of scalar–tensor theory in the
Einstein conformal frame. The field equation for the scalar field can be written in the form
 , where
, where 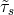 is a source consisting of a matter term, a scalar energy density term and a
term that mixes
is a source consisting of a matter term, a scalar energy density term and a
term that mixes 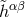 and
and 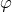 (see [286] for details).
(see [286] for details).
In order to incorporate the internal gravity of compact, self-gravitating bodies, it is common to adopt an
approach pioneered by Eardley [139], based in part on general arguments dating back to Dicke,
in which one treats the matter energy-momentum tensor as a sum of delta functions located
at the position of each body, but assumes that the mass of each body is a function 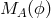 of the scalar field. This reflects the fact that the gravitational binding energy of the body is
controlled by the value of the gravitational constant, which is directly related to the value of
the background scalar field in which the body finds itself. The underlying assumption is that
the timescale for orbital motion is long compared to the internal dynamical timescale of the
body, so that the body’s structure evolves adiabatically in response to the changing scalar field.
Consequently, the matter action will have an effective dependence on
of the scalar field. This reflects the fact that the gravitational binding energy of the body is
controlled by the value of the gravitational constant, which is directly related to the value of
the background scalar field in which the body finds itself. The underlying assumption is that
the timescale for orbital motion is long compared to the internal dynamical timescale of the
body, so that the body’s structure evolves adiabatically in response to the changing scalar field.
Consequently, the matter action will have an effective dependence on 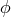 , and as a result the
field equations will depend on the “sensitivity” of the mass of each body to variations in the
scalar field, holding the total number of baryons fixed. The sensitivity of body
, and as a result the
field equations will depend on the “sensitivity” of the mass of each body to variations in the
scalar field, holding the total number of baryons fixed. The sensitivity of body 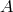 is defined by
is defined by
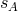 is
proportional to the Newtonian self-gravitational energy per unit mass of the body. From a theorem of
Hawking [185], for stationary black holes, it is known that
is
proportional to the Newtonian self-gravitational energy per unit mass of the body. From a theorem of
Hawking [185], for stationary black holes, it is known that 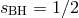 . This means, among other things,
that the source
. This means, among other things,
that the source 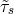 for the scalar field will contain an explicit term dependent upon
for the scalar field will contain an explicit term dependent upon 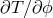 , because of
the dependence on
, because of
the dependence on 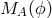 .
.
5.4.2 Equations of motion and gravitational waveform
By following the methods of post-Minkowskian theory adapted to scalar–tensor theory, it has been possible to derive the equations of motion for binary systems of compact bodies to 2.5PN order [286] and the gravitational-wave signal and energy flux to 1PN order beyond the quadrupole approximation. Here we shall quote selected results in parallel with those quoted in Section 5.3.2. The relative two-body equation of motion has the form
The key difference between this PN series and that in GR is the presence of a radiation-reaction term at 1.5PN order, caused by the emission of dipole gravitational radiation. The key parameters that appear in the two-body equations of motion are given in Table 6. Notice that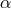 plays the role of a two-body
gravitational interaction parameter;
plays the role of a two-body
gravitational interaction parameter; 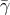 and
and  are the two-body versions of
are the two-body versions of 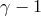 and
and 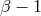 respectively. In the limit of weakly self-gravitating bodies (
respectively. In the limit of weakly self-gravitating bodies (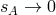 ),
), 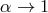 ,
, 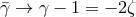 and
and
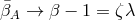 (compare with Table 3).
(compare with Table 3).
| Parameter | Definition |
|
Scalar–tensor parameters
|
|
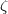 |
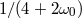 |
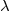 |
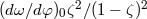 |
|
Sensitivities
|
|
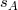 |
![[dlnMA (ϕ )∕d lnϕ]0](article1188x.gif) |
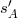 |
![[d2lnMA (ϕ )∕d ln ϕ2]0](article1190x.gif) |
|
Equation of motion parameters
|
|
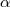 |
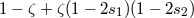 |
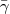 |
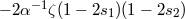 |
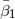 |
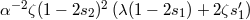 |
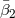 |
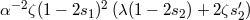 |
Here we quote only the 1PN corrections and the leading radiation-reaction terms at 1.5PN and 2.5PN order:
where where The periastron advance that results from these equations is given by where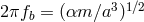 .
.
The tensor part of the gravitational waveform has the schematic form
where Contributions to the tensor waveform through 2PN order have been derived by Lang [241]. The scalar waveform is given by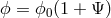 , where,
where, ignoring terms that are constant in time,
where
, where,
where, ignoring terms that are constant in time,
where 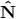 is a unit vector directed toward the observer.
is a unit vector directed toward the observer.
The energy flux is given by
where the first term is the contribution of dipole radiation (formally of –1PN order), and the second term (formally of 0PN order, according to the conventional rules of counting) is a combination of quadrupole radiation, PN corrections to monopole and dipole radiation, and even a cross-term between dipole and octupole radiation. The coefficients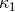 and
and 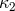 are given by [286]
These results are in complete agreement with the total energy flux to –1PN and 0PN orders, as
calculated by Damour and Esposito-Farèse [102]. They disagree with the flux formula of Will and
Zaglauer [434*], as repeated in earlier versions of this Living Review as well as in [7]. Will and
Zaglauer [434] failed to take into account PN corrections to the dipole term induced by PN
corrections in the equations of motion, and a dipole-octupole cross term in the scalar energy flux,
all of which contribute to the flux at the same 0PN order as the quadrupole and monopole
contributions.
are given by [286]
These results are in complete agreement with the total energy flux to –1PN and 0PN orders, as
calculated by Damour and Esposito-Farèse [102]. They disagree with the flux formula of Will and
Zaglauer [434*], as repeated in earlier versions of this Living Review as well as in [7]. Will and
Zaglauer [434] failed to take into account PN corrections to the dipole term induced by PN
corrections in the equations of motion, and a dipole-octupole cross term in the scalar energy flux,
all of which contribute to the flux at the same 0PN order as the quadrupole and monopole
contributions.
In the limit of weakly self-gravitating bodies the equations of motion and energy flux (including the dipole term) reduce to the standard results quoted in TEGP [420*].
5.4.3 Binary systems containing black holes
Roger Penrose was probably the first to conjecture, in a talk at the 1970 Fifth Texas Symposium, that black
holes in Brans–Dicke theory are identical to their GR counterparts [387]. Motivated by this remark, Thorne
and Dykla showed that during gravitational collapse to form a black hole, the Brans–Dicke scalar field is
radiated away, in accord with Price’s theorem, leaving only its constant asymptotic value, and a GR black
hole [387]. Hawking [185] proved on general grounds that stationary, asymptotically flat black holes in
vacuum in BD are the black holes of GR. The basic idea is that black holes in vacuum with non-singular
event horizons cannot support scalar “hair”. Hawking’s theorem was extended to the class of
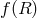 theories that can be transformed into generalized scalar–tensor theories by Sotiriou and
Faraoni [371].
theories that can be transformed into generalized scalar–tensor theories by Sotiriou and
Faraoni [371].
A consequence of these theorems is that, for a stationary black hole, 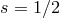 . Another way to see this
is to note that, because all information about the matter that formed the black hole has vanished behind
the event horizon, the only scale on which the mass of the hole can depend is the Planck scale, and thus
. Another way to see this
is to note that, because all information about the matter that formed the black hole has vanished behind
the event horizon, the only scale on which the mass of the hole can depend is the Planck scale, and thus
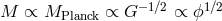 . Hence
. Hence 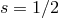 .
.
If both bodies in the binary system are black holes, then setting 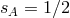 for each body, all the
parameters
for each body, all the
parameters 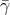 ,
, 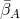 and
and 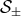 vanish identically, and
vanish identically, and 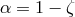 . But since
. But since 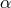 appears
only in the combination with
appears
only in the combination with 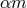 , a simple rescaling of each mass puts all equations into
complete agreement with those of GR. This is also true for the 2PN terms in the equations of
motion [286]. Thus, in the class of scalar–tensor theories discussed here, binary black holes
are observationally indistinguishable from their GR counterparts, at least to high orders in a
PN approximation. It has also been shown, in the extreme mass-ratio limit to first order in
the small mass, but to all PN orders, that binary black holes do not emit dipole gravitational
radiation [450].
, a simple rescaling of each mass puts all equations into
complete agreement with those of GR. This is also true for the 2PN terms in the equations of
motion [286]. Thus, in the class of scalar–tensor theories discussed here, binary black holes
are observationally indistinguishable from their GR counterparts, at least to high orders in a
PN approximation. It has also been shown, in the extreme mass-ratio limit to first order in
the small mass, but to all PN orders, that binary black holes do not emit dipole gravitational
radiation [450].
If one of the members of the binary system, say body 2, is a black hole, with 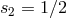 , then
, then
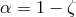 ,
, 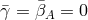 , and hence, through 1PN order, the motion is again identical to
that in GR. At 1.5PN order, dipole radiation reaction kicks in, since
, and hence, through 1PN order, the motion is again identical to
that in GR. At 1.5PN order, dipole radiation reaction kicks in, since 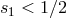 . In this case,
. In this case,
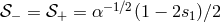 , and thus the 1.5PN coefficients in the relative equation of motion (94*) take
the form
, and thus the 1.5PN coefficients in the relative equation of motion (94*) take
the form
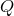 , as defined by
Eq. (106*).
, as defined by
Eq. (106*).
It should be pointed out that there are ways to induce scalar hair on a black hole. One is to introduce a
potential 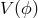 , which, depending on its form, can help to support a non-trivial scalar field outside a black
hole. Another is to introduce matter. A companion neutron star is an obvious choice, and such a binary
system in scalar–tensor theory is clearly different from its general relativistic counterpart. Another
possibility is a distribution of cosmological matter that can support a time-varying scalar field at infinity.
This possibility has been called “Jacobson’s miracle hair-growth formula” for black holes, based on work by
Jacobson [202, 191].
, which, depending on its form, can help to support a non-trivial scalar field outside a black
hole. Another is to introduce matter. A companion neutron star is an obvious choice, and such a binary
system in scalar–tensor theory is clearly different from its general relativistic counterpart. Another
possibility is a distribution of cosmological matter that can support a time-varying scalar field at infinity.
This possibility has been called “Jacobson’s miracle hair-growth formula” for black holes, based on work by
Jacobson [202, 191].

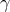


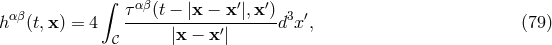
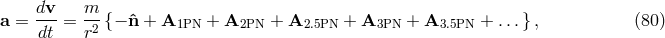
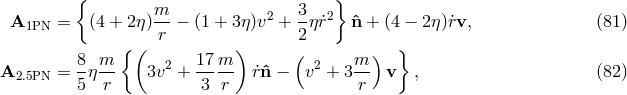

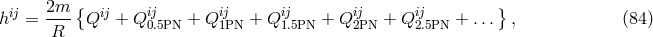


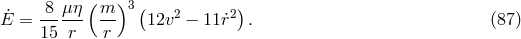
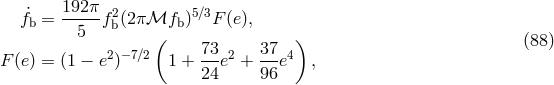

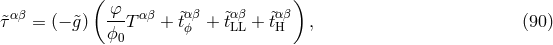

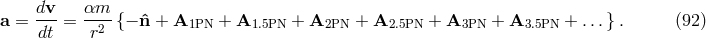
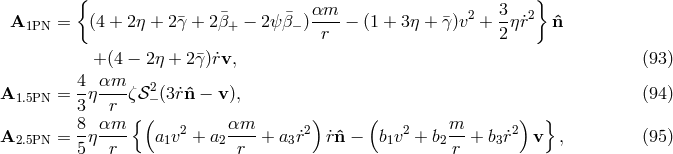
![5- 15-¯ 5- 2 15- a1 = 3 − 2γ¯+ 2 β+ + 8ζ𝒮 − (9 + 4¯γ − 2η) + 8 ζψ𝒮 − 𝒮+, [ ] ( ¯ ¯ ) a2 = 17-+ 35¯γ − 95-¯β+ − 5-ζ𝒮2− 135 + 56 ¯γ + 8η + 32¯β+ + 30ζ 𝒮− 𝒮−β+--+-𝒮+-β−- 3 6 6 24 ¯γ 5 ( 32 𝒮 β¯ + 𝒮 β¯ ) (𝒮 β¯ + 𝒮 β¯ )2 − -ζψ 𝒮− 𝒮+ − --𝒮− ¯β− + 16 -+--+----−--−- − 40ζ --+-+-----−--− , 8 3 ¯γ ¯γ 25-[ 2 ¯ ] a3 = 8 2¯γ − ζ𝒮 − (1 − 2η) − 4β+ − ζ ψ𝒮− 𝒮+ , 5 5 5 5 b1 = 1 − -γ¯+ -¯β+ − --ζ𝒮2− (7 + 4¯γ − 2η) +--ζψ𝒮 − 𝒮+, 6 2 24 8 ( ) 5- 5¯ 5-- 2[ ¯ ] 10- 𝒮−-¯β+-+-𝒮+-¯β−- b2 = 3 + 2¯γ − 2β+ − 24ζ𝒮 − 23 + 8 ¯γ − 8η + 8β+ + 3 ζ𝒮− ¯γ ( ¯ ¯ ) − 5ζψ 𝒮 𝒮 − 8𝒮 ¯β + 16-𝒮+-β+-+-𝒮−-β−- , 8 − + 3 − − 3 ¯γ 5 [ ] b3 = -- 6¯γ + ζ𝒮2− (13 + 8¯γ + 2η) − 12¯β+ − 3ζψ𝒮 − 𝒮+ , (96 ) 8](article1200x.gif)

![[ ¯ ¯ ] ˙ω = -6παmfb-- 1 + 2¯γ-−-β+-−--ψβ-− . (98 ) a (1 − e2) 3](article1202x.gif)
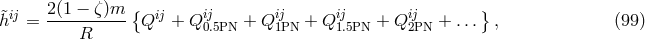

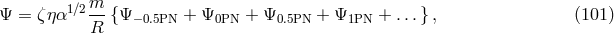
![Ψ −0.5PN = 4𝒮− (Nˆ ⋅ v), [ ˆ 2 αm-- ˆ 2] Ψ0PN = 2(𝒮+ − ψ𝒮− ) (N ⋅ v) − r (N ⋅ x) αm [ ( 𝒮 ¯β + 𝒮 ¯β ) ] − 2---- 3𝒮+ − ψ𝒮 − − 8 --+-+-----−-−- , r γ¯ ∂ { [ ( 𝒮+ ¯β+ + 𝒮− ¯β− ) (𝒮 −β¯+ + 𝒮+β¯−) ]} Ψ0.5PN = − --- (ˆN ⋅ x) (3 − 4η)𝒮 − − ψ𝒮+ + 8ψ -------------- − 8 -------------- ∂t ¯γ ¯γ 1- -∂3- ˆ 3 + 3 [(1 − 2η)𝒮 − − ψ𝒮+ ]∂t3(N ⋅ x) , (102 )](article1208x.gif)
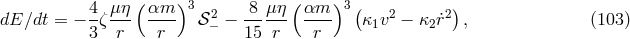
![( ¯ ¯ ) κ = 12 + 5 ¯γ − 5ζ𝒮2 (3 + γ¯+ 2 ¯β ) + 10ζ𝒮 𝒮-−β+-+-𝒮+-β−- 1 − + − ¯γ ( ¯ ¯ ) +10 ζ ψ𝒮2− ¯β− − 10 ζψ𝒮 − 𝒮+-β+-+-𝒮-−β−- , ¯γ 45 [ ] ( 𝒮 − ¯β+ + 𝒮+ ¯β− ) κ2 = 11 + --¯γ − 40 ¯β+ − 5ζ𝒮2− 17 + 6¯γ + η + 8β¯+ + 90ζ𝒮 − -------------- 4 ¯γ ( 𝒮+ ¯β+ + 𝒮 − ¯β− ) ( 𝒮+ ¯β+ + 𝒮 − ¯β− )2 +40 ζ ψ𝒮2− ¯β− − 30 ζψ𝒮 − -------------- − 120 ζ -------------- . (104 ) ¯γ ¯γ](article1213x.gif)