3 Metric Theories of Gravity and the PPN Formalism
3.1 Metric theories of gravity and the strong equivalence principle
3.1.1 Universal coupling and the metric postulates
The empirical evidence supporting the Einstein equivalence principle, discussed in Section 2, supports the conclusion that the only theories of gravity that have a hope of being viable are metric theories, or possibly theories that are metric apart from very weak or short-range non-metric couplings (as in string theory). Therefore for the remainder of this review, we shall turn our attention exclusively to metric theories of gravity, which assume that
- there exists a symmetric metric,
- test bodies follow geodesics of the metric, and
- in local Lorentz frames, the non-gravitational laws of physics are those of special relativity.
The property that all non-gravitational fields should couple in the same manner to a single gravitational field is sometimes called “universal coupling”. Because of it, one can discuss the metric as a property of spacetime itself rather than as a field over spacetime. This is because its properties may be measured and studied using a variety of different experimental devices, composed of different non-gravitational fields and particles, and, because of universal coupling, the results will be independent of the device. Thus, for instance, the proper time between two events is a characteristic of spacetime and of the location of the events, not of the clocks used to measure it.
Consequently, if EEP is valid, the non-gravitational laws of physics may be formulated by taking their
special relativistic forms in terms of the Minkowski metric 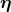 and simply “going over” to new forms
in terms of the curved spacetime metric
and simply “going over” to new forms
in terms of the curved spacetime metric 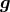 , using the mathematics of differential geometry.
The details of this “going over” can be found in standard textbooks (see [289*, 407, 355, 324*],
TEGP 3.2. [420*]).
, using the mathematics of differential geometry.
The details of this “going over” can be found in standard textbooks (see [289*, 407, 355, 324*],
TEGP 3.2. [420*]).
3.1.2 The strong equivalence principle
In any metric theory of gravity, matter and non-gravitational fields respond only to the spacetime metric
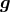 . In principle, however, there could exist other gravitational fields besides the metric, such as scalar
fields, vector fields, and so on. If, by our strict definition of metric theory, matter does not couple to these
fields, what can their role in gravitation theory be? Their role must be that of mediating the manner in
which matter and non-gravitational fields generate gravitational fields and produce the metric; once
determined, however, the metric alone acts back on the matter in the manner prescribed by
EEP.
. In principle, however, there could exist other gravitational fields besides the metric, such as scalar
fields, vector fields, and so on. If, by our strict definition of metric theory, matter does not couple to these
fields, what can their role in gravitation theory be? Their role must be that of mediating the manner in
which matter and non-gravitational fields generate gravitational fields and produce the metric; once
determined, however, the metric alone acts back on the matter in the manner prescribed by
EEP.
What distinguishes one metric theory from another, therefore, is the number and kind of gravitational fields it contains in addition to the metric, and the equations that determine the structure and evolution of these fields. From this viewpoint, one can divide all metric theories of gravity into two fundamental classes: “purely dynamical” and “prior-geometric”.
By “purely dynamical metric theory” we mean any metric theory whose gravitational fields have
their structure and evolution determined by coupled partial differential field equations. In other
words, the behavior of each field is influenced to some extent by a coupling to at least one of
the other fields in the theory. By “prior geometric” theory, we mean any metric theory that
contains “absolute elements”, fields or equations whose structure and evolution are given a priori,
and are independent of the structure and evolution of the other fields of the theory. These
“absolute elements” typically include flat background metrics 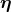 or cosmic time coordinates
or cosmic time coordinates
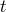 .
.
General relativity is a purely dynamical theory since it contains only one gravitational field, the metric
itself, and its structure and evolution are governed by partial differential equations (Einstein’s equations).
Brans–Dicke theory and its generalizations are purely dynamical theories; the field equation for the metric
involves the scalar field (as well as the matter as source), and that for the scalar field involves the metric.
Visser’s bimetric massive gravity theory [401*] is a prior-geometric theory: It has a non-dynamical,
Riemann-flat background metric 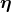 , and the field equations for the physical metric
, and the field equations for the physical metric 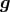 involve
involve
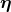 .
.
By discussing metric theories of gravity from this broad point of view, it is possible to draw some general conclusions about the nature of gravity in different metric theories, conclusions that are reminiscent of the Einstein equivalence principle, but that are subsumed under the name “strong equivalence principle”.
Consider a local, freely falling frame in any metric theory of gravity. Let this frame be small enough that
inhomogeneities in the external gravitational fields can be neglected throughout its volume. On the other
hand, let the frame be large enough to encompass a system of gravitating matter and its associated
gravitational fields. The system could be a star, a black hole, the solar system, or a Cavendish experiment.
Call this frame a “quasi-local Lorentz frame”. To determine the behavior of the system we must
calculate the metric. The computation proceeds in two stages. First we determine the external
behavior of the metric and gravitational fields, thereby establishing boundary values for the fields
generated by the local system, at a boundary of the quasi-local frame “far” from the local
system. Second, we solve for the fields generated by the local system. But because the metric is
coupled directly or indirectly to the other fields of the theory, its structure and evolution will be
influenced by those fields, and in particular by the boundary values taken on by those fields far
from the local system. This will be true even if we work in a coordinate system in which the
asymptotic form of 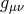 in the boundary region between the local system and the external
world is that of the Minkowski metric. Thus the gravitational environment in which the local
gravitating system resides can influence the metric generated by the local system via the boundary
values of the auxiliary fields. Consequently, the results of local gravitational experiments may
depend on the location and velocity of the frame relative to the external environment. Of course,
local non-gravitational experiments are unaffected since the gravitational fields they generate
are assumed to be negligible, and since those experiments couple only to the metric, whose
form can always be made locally Minkowskian at a given spacetime event. Local gravitational
experiments might include Cavendish experiments, measurement of the acceleration of massive
self-gravitating bodies, studies of the structure of stars and planets, or analyses of the periods of
“gravitational clocks”. We can now make several statements about different kinds of metric theories.
in the boundary region between the local system and the external
world is that of the Minkowski metric. Thus the gravitational environment in which the local
gravitating system resides can influence the metric generated by the local system via the boundary
values of the auxiliary fields. Consequently, the results of local gravitational experiments may
depend on the location and velocity of the frame relative to the external environment. Of course,
local non-gravitational experiments are unaffected since the gravitational fields they generate
are assumed to be negligible, and since those experiments couple only to the metric, whose
form can always be made locally Minkowskian at a given spacetime event. Local gravitational
experiments might include Cavendish experiments, measurement of the acceleration of massive
self-gravitating bodies, studies of the structure of stars and planets, or analyses of the periods of
“gravitational clocks”. We can now make several statements about different kinds of metric theories.
- A theory which contains only the metric
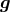 yields local gravitational physics which is
independent of the location and velocity of the local system. This follows from the fact that the
only field coupling the local system to the environment is
yields local gravitational physics which is
independent of the location and velocity of the local system. This follows from the fact that the
only field coupling the local system to the environment is 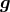 , and it is always possible to find
a coordinate system in which
, and it is always possible to find
a coordinate system in which 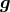 takes the Minkowski form at the boundary between the local
system and the external environment (neglecting inhomogeneities in the external gravitational
field). Thus the asymptotic values of
takes the Minkowski form at the boundary between the local
system and the external environment (neglecting inhomogeneities in the external gravitational
field). Thus the asymptotic values of 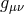 are constants independent of location, and are
asymptotically Lorentz invariant, thus independent of velocity. GR is an example of such a
theory.
are constants independent of location, and are
asymptotically Lorentz invariant, thus independent of velocity. GR is an example of such a
theory.
- A theory which contains the metric
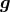 and dynamical scalar fields
and dynamical scalar fields 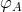 yields local
gravitational physics which may depend on the location of the frame but which is independent of
the velocity of the frame. This follows from the asymptotic Lorentz invariance of the Minkowski
metric and of the scalar fields, but now the asymptotic values of the scalar fields may depend on
the location of the frame. An example is Brans–Dicke theory, where the asymptotic scalar field
determines the effective value of the gravitational constant, which can thus vary as
yields local
gravitational physics which may depend on the location of the frame but which is independent of
the velocity of the frame. This follows from the asymptotic Lorentz invariance of the Minkowski
metric and of the scalar fields, but now the asymptotic values of the scalar fields may depend on
the location of the frame. An example is Brans–Dicke theory, where the asymptotic scalar field
determines the effective value of the gravitational constant, which can thus vary as 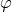 varies.
On the other hand, a form of velocity dependence in local physics can enter indirectly if the
asymptotic values of the scalar field vary with time cosmologically. Then the rate of variation
of the gravitational constant could depend on the velocity of the frame.
varies.
On the other hand, a form of velocity dependence in local physics can enter indirectly if the
asymptotic values of the scalar field vary with time cosmologically. Then the rate of variation
of the gravitational constant could depend on the velocity of the frame.
- A theory which contains the metric
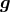 and additional dynamical vector or tensor fields
or prior-geometric fields yields local gravitational physics which may have both location
and velocity-dependent effects. An example is the Einstein-Æther theory, which contains a
dynamical timelike four-vector field; the large-scale distribution of matter establishes a frame
in which the vector has no spatial components, and systems moving relative to that frame can
experience motion-dependent effects.
and additional dynamical vector or tensor fields
or prior-geometric fields yields local gravitational physics which may have both location
and velocity-dependent effects. An example is the Einstein-Æther theory, which contains a
dynamical timelike four-vector field; the large-scale distribution of matter establishes a frame
in which the vector has no spatial components, and systems moving relative to that frame can
experience motion-dependent effects.
These ideas can be summarized in the strong equivalence principle (SEP), which states that:
- WEP is valid for self-gravitating bodies as well as for test bodies.
- The outcome of any local test experiment is independent of the velocity of the (freely falling) apparatus.
- The outcome of any local test experiment is independent of where and when in the universe it is performed.
The distinction between SEP and EEP is the inclusion of bodies with self-gravitational interactions (planets, stars) and of experiments involving gravitational forces (Cavendish experiments, gravimeter measurements). Note that SEP contains EEP as the special case in which local gravitational forces are ignored. For further discussion of SEP and EEP, see [128].
The above discussion of the coupling of auxiliary fields to local gravitating systems indicates that if SEP
is strictly valid, there must be one and only one gravitational field in the universe, the metric 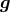 . These
arguments are only suggestive however, and no rigorous proof of this statement is available at present.
Empirically it has been found that almost every metric theory other than GR introduces auxiliary
gravitational fields, either dynamical or prior geometric, and thus predicts violations of SEP at some level
(here we ignore quantum-theory inspired modifications to GR involving “
. These
arguments are only suggestive however, and no rigorous proof of this statement is available at present.
Empirically it has been found that almost every metric theory other than GR introduces auxiliary
gravitational fields, either dynamical or prior geometric, and thus predicts violations of SEP at some level
(here we ignore quantum-theory inspired modifications to GR involving “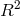 ” terms). The
one exception is Nordström’s 1913 conformally-flat scalar theory [303], which can be written
purely in terms of the metric; the theory satisfies SEP, but unfortunately violates experiment by
predicting no deflection of light. General relativity seems to be the only viable metric theory that
embodies SEP completely. In Section 4.3, we shall discuss experimental evidence for the validity of
SEP.
” terms). The
one exception is Nordström’s 1913 conformally-flat scalar theory [303], which can be written
purely in terms of the metric; the theory satisfies SEP, but unfortunately violates experiment by
predicting no deflection of light. General relativity seems to be the only viable metric theory that
embodies SEP completely. In Section 4.3, we shall discuss experimental evidence for the validity of
SEP.
3.2 The parametrized post-Newtonian formalism
Despite the possible existence of long-range gravitational fields in addition to the metric in various metric
theories of gravity, the postulates of those theories demand that matter and non-gravitational fields be
completely oblivious to them. The only gravitational field that enters the equations of motion is the metric
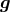 . The role of the other fields that a theory may contain can only be that of helping to generate the
spacetime curvature associated with the metric. Matter may create these fields, and they plus the matter
may generate the metric, but they cannot act back directly on the matter. Matter responds only to the
metric.
. The role of the other fields that a theory may contain can only be that of helping to generate the
spacetime curvature associated with the metric. Matter may create these fields, and they plus the matter
may generate the metric, but they cannot act back directly on the matter. Matter responds only to the
metric.
Thus the metric and the equations of motion for matter become the primary entities for calculating observable effects, and all that distinguishes one metric theory from another is the particular way in which matter and possibly other gravitational fields generate the metric.
The comparison of metric theories of gravity with each other and with experiment becomes particularly
simple when one takes the slow-motion, weak-field limit. This approximation, known as the post-Newtonian
limit, is sufficiently accurate to encompass most solar-system tests that can be performed in the foreseeable
future. It turns out that, in this limit, the spacetime metric 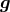 predicted by nearly every metric theory of
gravity has the same structure. It can be written as an expansion about the Minkowski metric
(
predicted by nearly every metric theory of
gravity has the same structure. It can be written as an expansion about the Minkowski metric
(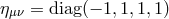 ) in terms of dimensionless gravitational potentials of varying degrees of smallness.
These potentials are constructed from the matter variables (see Box 2) in imitation of the Newtonian
gravitational potential
) in terms of dimensionless gravitational potentials of varying degrees of smallness.
These potentials are constructed from the matter variables (see Box 2) in imitation of the Newtonian
gravitational potential
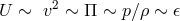 ,
,
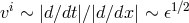 , and so on (we use units in which
, and so on (we use units in which 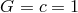 ; see Box 2 for definitions and
conventions).
; see Box 2 for definitions and
conventions).
A consistent post-Newtonian limit requires determination of 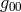 correct through
correct through 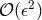 ,
, 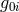 through
through 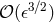 , and
, and 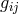 through
through 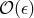 (for details see TEGP 4.1 [420*]). The only way that one
metric theory differs from another is in the numerical values of the coefficients that appear in front of the
metric potentials. The parametrized post-Newtonian (PPN) formalism inserts parameters in place of
these coefficients, parameters whose values depend on the theory under study. In the current
version of the PPN formalism, summarized in Box 2, ten parameters are used, chosen in such
a manner that they measure or indicate general properties of metric theories of gravity (see
Table 2). Under reasonable assumptions about the kinds of potentials that can be present
at post-Newtonian order (basically only Poisson-like potentials of conventional perfect fluid
sources, absence of parity-violating potentials), one finds that ten PPN parameters exhaust the
possibilities.
(for details see TEGP 4.1 [420*]). The only way that one
metric theory differs from another is in the numerical values of the coefficients that appear in front of the
metric potentials. The parametrized post-Newtonian (PPN) formalism inserts parameters in place of
these coefficients, parameters whose values depend on the theory under study. In the current
version of the PPN formalism, summarized in Box 2, ten parameters are used, chosen in such
a manner that they measure or indicate general properties of metric theories of gravity (see
Table 2). Under reasonable assumptions about the kinds of potentials that can be present
at post-Newtonian order (basically only Poisson-like potentials of conventional perfect fluid
sources, absence of parity-violating potentials), one finds that ten PPN parameters exhaust the
possibilities.
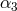 has been shown twice to
indicate that it is a measure of two effects).
has been shown twice to
indicate that it is a measure of two effects).|
Parameter |
What it measures relative to GR |
Value
in GR
|
Value in
semiconservative
theories
|
Value in
fully
conservative
theories
|
|
|
How much space-curvature produced by unit rest mass? |
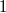 |
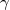 |
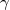 |
|
|
|
|
|
|
|
|
How much “nonlinearity” in the superposition law for gravity? |
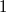 |
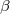 |
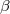 |
|
|
Preferred-location effects? |
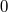 |
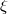 |
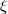 |
|
|
Preferred-frame effects? |
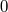 |
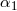 |
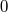 |
|
|
|
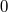 |
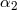 |
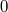 |
|
|
|
 |
 |
 |
|
|
Violation of conservation |
 |
 |
 |
|
|
of total momentum? |
 |
 |
 |
|
|
|
 |
 |
 |
|
|
|
 |
 |
 |
|
|
|
 |
 |
 |
The parameters 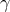 and
and 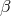 are the usual Eddington–Robertson–Schiff parameters used to describe the
“classical” tests of GR, and are in some sense the most important; they are the only non-zero parameters in
GR and scalar–tensor gravity. The parameter
are the usual Eddington–Robertson–Schiff parameters used to describe the
“classical” tests of GR, and are in some sense the most important; they are the only non-zero parameters in
GR and scalar–tensor gravity. The parameter 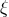 is non-zero in any theory of gravity that predicts
preferred-location effects such as a galaxy-induced anisotropy in the local gravitational constant
is non-zero in any theory of gravity that predicts
preferred-location effects such as a galaxy-induced anisotropy in the local gravitational constant 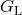 (also
called “Whitehead” effects);
(also
called “Whitehead” effects); 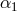 ,
, 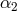 ,
, 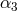 measure whether or not the theory predicts post-Newtonian
preferred-frame effects;
measure whether or not the theory predicts post-Newtonian
preferred-frame effects; 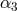 ,
, 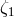 ,
, 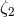 ,
, 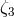 ,
, 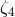 measure whether or not the theory predicts violations of
global conservation laws for total momentum. In Table 2 we show the values these parameters
take
measure whether or not the theory predicts violations of
global conservation laws for total momentum. In Table 2 we show the values these parameters
take
- in GR,
- in any theory of gravity that possesses conservation laws for total momentum, called “semi-conservative” (any theory that is based on an invariant action principle is semi-conservative), and
- in any theory that in addition possesses six global conservation laws for angular momentum, called “fully conservative” (such theories automatically predict no post-Newtonian preferred-frame effects).
Semi-conservative theories have five free PPN parameters (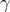 ,
, 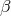 ,
, 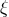 ,
, 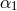 ,
, 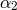 ) while fully conservative
theories have three (
) while fully conservative
theories have three (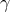 ,
, 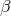 ,
, 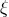 ).
).
The PPN formalism was pioneered by Kenneth Nordtvedt [305], who studied the post-Newtonian metric of a system of gravitating point masses, extending earlier work by Eddington, Robertson and Schiff (TEGP 4.2 [420*]). Will [413] generalized the framework to perfect fluids. A general and unified version of the PPN formalism was developed by Will and Nordtvedt [431*]. The canonical version, with conventions altered to be more in accord with standard textbooks such as [289], is discussed in detail in TEGP 4 [420*]. Other versions of the PPN formalism have been developed to deal with point masses with charge, fluid with anisotropic stresses, bodies with strong internal gravity, and post-post-Newtonian effects (TEGP 4.2, 14.2 [420*]). Additional parameters or potentials are needed to deal with some theories, such as theories with massive fields (Yukawa-type potentials replace Poisson potentials), or theories like Chern–Simons theory, which permit parity violation in gravity.
Box 2. The Parametrized Post-Newtonian formalism
- Coordinate system:
-
The framework uses a nearly globally Lorentz coordinate system in which the coordinates are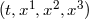 . Three-dimensional, Euclidean vector notation is used throughout. All coordinate
arbitrariness (“gauge freedom”) has been removed by specialization of the coordinates to the standard
PPN gauge (TEGP 4.2 [420*]). Units are chosen so that
. Three-dimensional, Euclidean vector notation is used throughout. All coordinate
arbitrariness (“gauge freedom”) has been removed by specialization of the coordinates to the standard
PPN gauge (TEGP 4.2 [420*]). Units are chosen so that 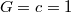 , where
, where 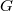 is the physically
measured Newtonian constant far from the solar system.
is the physically
measured Newtonian constant far from the solar system.
- Matter variables:
-
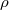 : density of rest mass as measured in a local freely falling frame momentarily comoving with
the gravitating matter.
: density of rest mass as measured in a local freely falling frame momentarily comoving with
the gravitating matter.
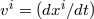 : coordinate velocity of the matter.
: coordinate velocity of the matter.
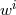 : coordinate velocity of the PPN coordinate system relative to the mean rest-frame of the
universe.
: coordinate velocity of the PPN coordinate system relative to the mean rest-frame of the
universe.
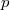 : pressure as measured in a local freely falling frame momentarily comoving with the matter.
: pressure as measured in a local freely falling frame momentarily comoving with the matter.
 : internal energy per unit rest mass (it includes all forms of non-rest-mass, non-gravitational
energy, e.g., energy of compression and thermal energy).
: internal energy per unit rest mass (it includes all forms of non-rest-mass, non-gravitational
energy, e.g., energy of compression and thermal energy).
- PPN parameters:
-
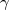 ,
, 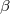 ,
, 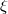 ,
, 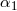 ,
, 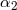 ,
, 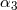 ,
, 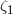 ,
, 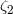 ,
, 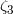 ,
, 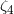 .
.
- Metric:
-
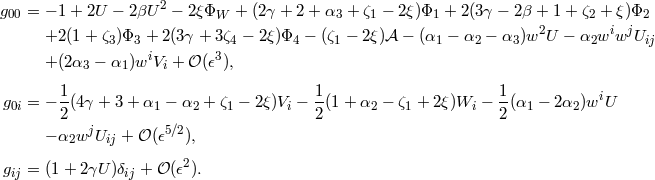
- Metric potentials:
-
![∫ ---ρ′-- 3 ′ U = |x− x ′|d x , ∫ ρ′(x-−-x′)i(x-−-x′)j- 3 ′ Uij = |x − x′|3 d x, ∫ ( ) ρ′ρ′′(x-−-x-′) -x′ −-x′′ x-−-x-′′- 3 ′3 ′′ ΦW = |x − x′|3 ⋅ |x − x′′| − |x′ − x′′| d x d x , ∫ ρ′[v′ ⋅-(x-−-x′)]2 3 ′ 𝒜 = |x − x′|3 d x , ∫ -ρ′v′2- 3 ′ Φ1 = |x− x ′|d x , ∫ -ρ-′U-′- 3 ′ Φ2 = |x− x ′|d x , ∫ --ρ′Π-′- 3 ′ Φ3 = |x− x ′|d x , ∫ ---p′-- 3 ′ Φ4 = |x− x ′|d x , ∫ --ρ′v′i-- 3 ′ Vi = |x− x ′|d x , ∫ W = ρ′[v′ ⋅-(x-−-x′)](x-−-x′)id3x ′. i |x − x′|3](article467x.gif)
- Stress–energy tensor (perfect fluid):
-
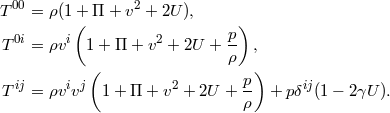
- Equations of motion:
-
- Stressed matter:
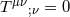 .
.
- Test bodies:
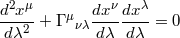 .
.
- Maxwell’s equations:
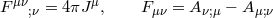 .
.
- Stressed matter:
3.3 Competing theories of gravity
One of the important applications of the PPN formalism is the comparison and classification of alternative metric theories of gravity. The population of viable theories has fluctuated over the years as new effects and tests have been discovered, largely through the use of the PPN framework, which eliminated many theories thought previously to be viable. The theory population has also fluctuated as new, potentially viable theories have been invented.
In this review, we shall focus on GR, the general class of scalar–tensor modifications of it, of which the Jordan–Fierz–Brans–Dicke theory (Brans–Dicke, for short) is the classic example, and vector-tensor theories. The reasons are several-fold:
- A full compendium of alternative theories circa 1981 is given in TEGP 5 [420*].
- Many alternative metric theories developed during the 1970s and 1980s could be viewed as “straw-man” theories, invented to prove that such theories exist or to illustrate particular properties. Few of these could be regarded as well-motivated theories from the point of view, say, of field theory or particle physics.
- A number of theories fall into the class of “prior-geometric” theories, with absolute elements such as a flat background metric in addition to the physical metric. Most of these theories predict “preferred-frame” effects, that have been tightly constrained by observations (see Section 4.3.2). An example is Rosen’s bimetric theory.
- A large number of alternative theories of gravity predict gravitational wave emission substantially different from that of GR, in strong disagreement with observations of the binary pulsar (see Section 9).
- Scalar–tensor modifications of GR have become very popular in unification schemes such as string theory, and in cosmological model building. Because the scalar fields could be massive, the potentials in the post-Newtonian limit could be modified by Yukawa-like terms.
- Theories that also incorporate vector fields have attracted recent attention, in the spirit of the SME (see Section 2.2.4), as models for violations of Lorentz invariance in the gravitational sector, and as potential candidates to account for phenomena such as galaxy rotation curves without resorting to dark matter.
3.3.1 General relativity
The metric 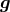 is the sole dynamical field, and the theory contains no arbitrary functions or parameters,
apart from the value of the Newtonian coupling constant
is the sole dynamical field, and the theory contains no arbitrary functions or parameters,
apart from the value of the Newtonian coupling constant 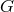 , which is measurable in laboratory
experiments. Throughout this article, we ignore the cosmological constant
, which is measurable in laboratory
experiments. Throughout this article, we ignore the cosmological constant 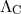 . We do this despite recent
evidence, from supernova data, of an accelerating universe, which would indicate either a non-zero
cosmological constant or a dynamical “dark energy” contributing about 70 percent of the critical density.
Although
. We do this despite recent
evidence, from supernova data, of an accelerating universe, which would indicate either a non-zero
cosmological constant or a dynamical “dark energy” contributing about 70 percent of the critical density.
Although 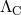 has significance for quantum field theory, quantum gravity, and cosmology, on the scale of
the solar-system or of stellar systems its effects are negligible, for the values of
has significance for quantum field theory, quantum gravity, and cosmology, on the scale of
the solar-system or of stellar systems its effects are negligible, for the values of 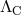 inferred from
supernova observations.
inferred from
supernova observations.
The field equations of GR are derivable from an invariant action principle 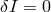 , where
, where
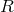 is the Ricci scalar, and
is the Ricci scalar, and 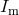 is the matter action, which depends on matter fields
is the matter action, which depends on matter fields 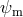 universally coupled to the metric
universally coupled to the metric 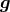 . By varying the action with respect to
. By varying the action with respect to 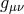 , we obtain the field
equations
where
, we obtain the field
equations
where 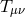 is the matter energy-momentum tensor. General covariance of the matter action implies the
equations of motion
is the matter energy-momentum tensor. General covariance of the matter action implies the
equations of motion 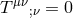 ; varying
; varying 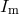 with respect to
with respect to 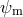 yields the matter field equations of the
standard model. By virtue of the absence of prior-geometric elements, the equations of motion are also a
consequence of the field equations via the Bianchi identities
yields the matter field equations of the
standard model. By virtue of the absence of prior-geometric elements, the equations of motion are also a
consequence of the field equations via the Bianchi identities 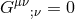 . According to our choice of units,
we set
. According to our choice of units,
we set 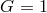 .
.
The general procedure for deriving the post-Newtonian limit of metric theories is spelled out in TEGP 5.1 [420*], and is described in detail for GR in TEGP 5.2 [420*] (see also Chapters 6 – 8 of [324*]). The PPN parameter values are listed in Table 3.
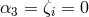 for all cases). The
parameters
for all cases). The
parameters 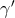 ,
, 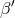 ,
, 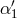 , and
, and 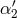 denote complicated functions of the arbitrary constants and
matching parameters.
denote complicated functions of the arbitrary constants and
matching parameters.| Theory | Arbitrary | Cosmic |
PPN parameters
|
||||
| functions | matching |
_______________________________________
|
|||||
| or constants | parameters | 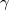 |
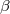 |
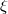 |
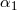 |
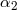 |
|
| General relativity | none | none |  |
 |
 |
 |
 |
| Scalar–tensor | |||||||
| Brans–Dicke | 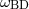 |
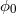 |
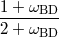 |
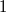 |
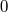 |
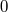 |
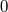 |
General, 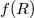 |
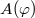 , ,  |
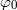 |
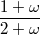 |
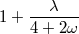 |
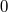 |
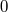 |
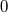 |
| Vector–tensor | |||||||
| Unconstrained | 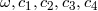 |
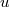 |
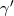 |
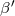 |
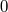 |
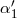 |
 |
| Einstein-Æther | 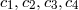 |
none | 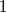 |
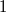 |
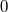 |
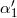 |
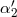 |
| Khronometric | 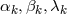 |
none | 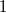 |
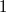 |
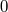 |
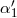 |
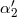 |
| Tensor–Vector–Scalar | 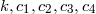 |
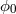 |
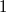 |
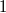 |
 |
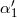 |
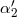 |
3.3.2 Scalar–tensor theories
These theories contain the metric 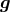 , a scalar field
, a scalar field 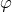 , a potential function
, a potential function 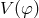 , and a
coupling function
, and a
coupling function 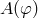 (generalizations to more than one scalar field have also been carried
out [102*]). For some purposes, the action is conveniently written in a non-metric representation,
sometimes denoted the “Einstein frame”, in which the gravitational action looks exactly like
that of GR and the scalar action looks like a minimally coupled scalar field with a potential:
(generalizations to more than one scalar field have also been carried
out [102*]). For some purposes, the action is conveniently written in a non-metric representation,
sometimes denoted the “Einstein frame”, in which the gravitational action looks exactly like
that of GR and the scalar action looks like a minimally coupled scalar field with a potential:
 is the Ricci scalar of the “Einstein” metric
is the Ricci scalar of the “Einstein” metric 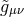 . (Apart from the scalar potential
term
. (Apart from the scalar potential
term 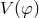 , this corresponds to Eq. (28*) with
, this corresponds to Eq. (28*) with  ,
, 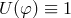 , and
, and 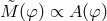 .)
This representation is a “non-metric” one because the matter fields
.)
This representation is a “non-metric” one because the matter fields 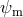 couple to a combination of
couple to a combination of 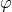 and
and 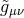 . Despite appearances, however, it is a metric theory, because it can be put into a metric
representation by identifying the “physical metric”
The action can then be rewritten in the metric form
where
The Einstein frame is useful for discussing general characteristics of such theories, for numerical relativity
calculations, and for some cosmological applications, while the metric representation is most useful for
calculating observable effects. The field equations, post-Newtonian limit and PPN parameters are discussed
in TEGP 5.3 [420*] (see also Section 13.5 of [324*]), and the values of the PPN parameters are listed in
Table 3.
. Despite appearances, however, it is a metric theory, because it can be put into a metric
representation by identifying the “physical metric”
The action can then be rewritten in the metric form
where
The Einstein frame is useful for discussing general characteristics of such theories, for numerical relativity
calculations, and for some cosmological applications, while the metric representation is most useful for
calculating observable effects. The field equations, post-Newtonian limit and PPN parameters are discussed
in TEGP 5.3 [420*] (see also Section 13.5 of [324*]), and the values of the PPN parameters are listed in
Table 3.
The parameters that enter the post-Newtonian limit are
where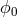 is the value of
is the value of 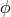 today far from the system being studied, as determined by appropriate
cosmological boundary conditions. The Newtonian gravitational constant
today far from the system being studied, as determined by appropriate
cosmological boundary conditions. The Newtonian gravitational constant 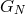 , which is set
equal to unity by our choice of units, is related to the coupling constant
, which is set
equal to unity by our choice of units, is related to the coupling constant 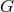 ,
,  and
and 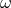 by
In Brans–Dicke theory (
by
In Brans–Dicke theory (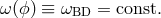 ), the larger the value of
), the larger the value of 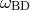 , the smaller the effects of the
scalar field, and in the limit
, the smaller the effects of the
scalar field, and in the limit 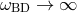 (
(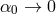 ), the theory becomes indistinguishable from GR
in all its predictions. In more general theories, the function
), the theory becomes indistinguishable from GR
in all its predictions. In more general theories, the function 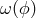 could have the property
that, at the present epoch, and in weak-field situations, the value of the scalar field
could have the property
that, at the present epoch, and in weak-field situations, the value of the scalar field 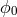 is
such that
is
such that 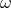 is very large and
is very large and 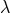 is very small (theory almost identical to GR today), but
that for past or future values of
is very small (theory almost identical to GR today), but
that for past or future values of 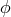 , or in strong-field regions such as the interiors of neutron
stars,
, or in strong-field regions such as the interiors of neutron
stars,  and
and 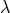 could take on values that would lead to significant differences from GR.
It is useful to point out that all versions of scalar–tensor gravity predict that
could take on values that would lead to significant differences from GR.
It is useful to point out that all versions of scalar–tensor gravity predict that 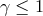 (see
Table 3).
(see
Table 3).
Damour and Esposito-Farèse [102*] have adopted an alternative parametrization of scalar–tensor
theories, in which one expands 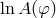 about a cosmological background field value
about a cosmological background field value 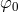 :
:
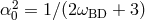 , or
, or
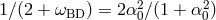 . The function
. The function 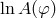 acts as a potential for the scalar field
acts as a potential for the scalar field 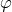 within
matter, and, if
within
matter, and, if 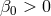 , then during cosmological evolution, the scalar field naturally evolves toward the
minimum of the potential, i.e., toward
, then during cosmological evolution, the scalar field naturally evolves toward the
minimum of the potential, i.e., toward 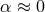 ,
, 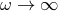 , or toward a theory close to, though not
precisely GR [112, 113]. Estimates of the expected relic deviations from GR today in such
theories depend on the cosmological model, but range from
, or toward a theory close to, though not
precisely GR [112, 113]. Estimates of the expected relic deviations from GR today in such
theories depend on the cosmological model, but range from 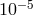 to a few times
to a few times 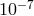 for
for
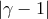 .
.
Negative values of 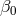 correspond to a “locally unstable” scalar potential (the overall theory is still
stable in the sense of having no tachyons or ghosts). In this case, objects such as neutron stars can
experience a “spontaneous scalarization”, whereby the interior values of
correspond to a “locally unstable” scalar potential (the overall theory is still
stable in the sense of having no tachyons or ghosts). In this case, objects such as neutron stars can
experience a “spontaneous scalarization”, whereby the interior values of 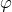 can be very different from the
exterior values, through non-linear interactions between strong gravity and the scalar field, dramatically
affecting the stars’ internal structure and leading to strong violations of SEP [103, 104]. There is evidence
from recent numerical simulations of the occurrence of a dynamically induced scalarization during the
inspirals of compact binary systems containing neutron stars, which can affect both the final motion and the
gravitational-wave emission [32, 313, 364].
can be very different from the
exterior values, through non-linear interactions between strong gravity and the scalar field, dramatically
affecting the stars’ internal structure and leading to strong violations of SEP [103, 104]. There is evidence
from recent numerical simulations of the occurrence of a dynamically induced scalarization during the
inspirals of compact binary systems containing neutron stars, which can affect both the final motion and the
gravitational-wave emission [32, 313, 364].
On the other hand, in the case 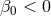 , one must confront that fact that, with an unstable
, one must confront that fact that, with an unstable 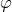 potential, cosmological evolution would presumably drive the system away from the peak where
potential, cosmological evolution would presumably drive the system away from the peak where 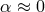 ,
toward parameter values that could be excluded by solar system experiments.
,
toward parameter values that could be excluded by solar system experiments.
Scalar fields coupled to gravity or matter are also ubiquitous in particle-physics-inspired models of unification, such as string theory [384, 266, 117, 114, 115]. In some models, the coupling to matter may lead to violations of EEP, which could be tested or bounded by the experiments described in Section 2.1. In many models the scalar field could be massive; if the Compton wavelength is of macroscopic scale, its effects are those of a “fifth force”. Only if the theory can be cast as a metric theory with a scalar field of infinite range or of range long compared to the scale of the system in question (solar system) can the PPN framework be strictly applied. If the mass of the scalar field is sufficiently large that its range is microscopic, then, on solar-system scales, the scalar field is suppressed, and the theory is essentially equivalent to general relativity.
For a detailed review of scalar–tensor theories see [167].
3.3.3 f(R) theories
These are theories whose action has the form
where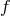 is a function chosen so that at cosmological scales, the universe will experience accelerated
expansion without resorting to either a cosmological constant or dark energy. However, it turns out that
such theories are equivalent to scalar–tensor theories: replace
is a function chosen so that at cosmological scales, the universe will experience accelerated
expansion without resorting to either a cosmological constant or dark energy. However, it turns out that
such theories are equivalent to scalar–tensor theories: replace 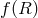 by
by 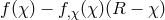 , where
, where 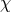 is a dynamical scalar field. Varying the action with respect to
is a dynamical scalar field. Varying the action with respect to 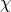 yields
yields  , which implies
that
, which implies
that 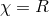 as long as
as long as 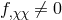 . Then defining a scalar field
. Then defining a scalar field 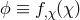 one puts the action into the
form of a scalar–tensor theory given by Eq. (35*), with
one puts the action into the
form of a scalar–tensor theory given by Eq. (35*), with 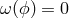 and
and 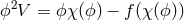 . As we
will see, this value of
. As we
will see, this value of 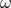 would ordinarily strongly violate solar-system experiments, but it turns out that
in many models, the potential
would ordinarily strongly violate solar-system experiments, but it turns out that
in many models, the potential 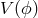 has the effect of giving the scalar field a large effective mass in the
presence of matter (the so-called “chameleon mechanism” [216]), so that the scalar field is suppressed at
distances that extend outside bodies like the Sun and Earth. In this way, with only modest
fine tuning,
has the effect of giving the scalar field a large effective mass in the
presence of matter (the so-called “chameleon mechanism” [216]), so that the scalar field is suppressed at
distances that extend outside bodies like the Sun and Earth. In this way, with only modest
fine tuning, 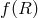 theories can claim to obey standard tests, while providing interesting, non
general-relativistic behavior on cosmic scales. For detailed reviews of this class of theories, see [370] and
[122].
theories can claim to obey standard tests, while providing interesting, non
general-relativistic behavior on cosmic scales. For detailed reviews of this class of theories, see [370] and
[122].
3.3.4 Vector–tensor theories
These theories contain the metric 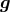 and a dynamical, typically timelike, four-vector field
and a dynamical, typically timelike, four-vector field 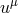 . In some
models, the four-vector is unconstrained, while in others, called Einstein-Æther theories it is constrained to
be timelike with unit norm. The most general action for such theories that is quadratic in derivatives of the
vector is given by
. In some
models, the four-vector is unconstrained, while in others, called Einstein-Æther theories it is constrained to
be timelike with unit norm. The most general action for such theories that is quadratic in derivatives of the
vector is given by
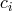 are arbitrary. In the unconstrained theories,
are arbitrary. In the unconstrained theories, 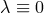 and
and 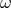 is arbitrary. In the
constrained theories,
is arbitrary. In the
constrained theories, 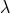 is a Lagrange multiplier, and by virtue of the constraint
is a Lagrange multiplier, and by virtue of the constraint 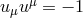 , the factor
, the factor
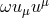 in front of the Ricci scalar can be absorbed into a rescaling of
in front of the Ricci scalar can be absorbed into a rescaling of 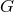 ; equivalently, in the
constrained theories, we can set
; equivalently, in the
constrained theories, we can set 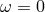 . Note that the possible term
. Note that the possible term 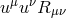 can be shown under
integration by parts to be equivalent to a linear combination of the terms involving
can be shown under
integration by parts to be equivalent to a linear combination of the terms involving 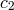 and
and
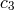 .
.
Unconstrained theories were studied during the 1970s as “straw-man” alternatives to GR. In addition to
having up to four arbitrary parameters, they also left the magnitude of the vector field arbitrary, since it
satisfies a linear homogenous vacuum field equation of the form 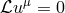 (
(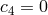 in all
such cases studied). Indeed, this latter fact was one of most serious defects of these theories.
Each unconstrained theory studied corresponds to a special case of the action (41*), all with
in all
such cases studied). Indeed, this latter fact was one of most serious defects of these theories.
Each unconstrained theory studied corresponds to a special case of the action (41*), all with
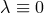 :
:
-
General vector–tensor theory;
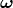 ,
, 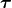 ,
,  ,
, 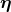
-
The gravitational Lagrangian for this class of theories had the form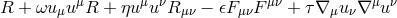 , where
, where 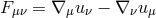 ,
corresponding to the values
,
corresponding to the values 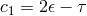 ,
, 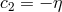 ,
, 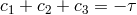 ,
, 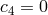 . In these
theories
. In these
theories 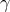 ,
, 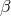 ,
, 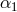 , and
, and 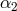 are complicated functions of the parameters and of
are complicated functions of the parameters and of
 , while the rest vanish (see TEGP 5.4 [420*]).
, while the rest vanish (see TEGP 5.4 [420*]).
- Will–Nordtvedt theory
-
This is the special case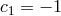 ,
,  . In this theory, the PPN parameters are
given by
. In this theory, the PPN parameters are
given by 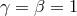 ,
, 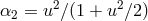 , and zero for the rest [431]).
, and zero for the rest [431]).
-
Hellings–Nordtvedt theory;
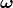
-
This is the special case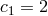 ,
, 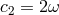 ,
, 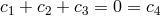 . Here
. Here 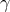 ,
,  ,
, 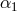 and
and
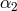 are complicated functions of the parameters and of
are complicated functions of the parameters and of 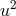 , while the rest vanish [187].
, while the rest vanish [187].
-
Einstein-Æther theory;
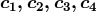
-
The Einstein-Æther theories were motivated in part by a desire to explore possibilities for violations of Lorentz invariance in gravity, in parallel with similar studies in matter interactions, such as the SME. The general class of theories was analyzed by Jacobson and collaborators [204, 274, 205*, 147, 163*], motivated in part by [230]. Analyzing the post-Newtonian limit,1 they were able to infer values of the PPN parameters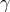 and
and 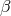 as follows [163]:
where
as follows [163]:
where 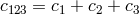 ,
, 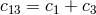 ,
, 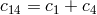 , subject to the constraints
, subject to the constraints 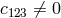 ,
,
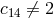 ,
, 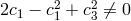 . By requiring that gravitational-wave modes have real (as opposed
to imaginary) frequencies, one can impose the bounds
. By requiring that gravitational-wave modes have real (as opposed
to imaginary) frequencies, one can impose the bounds 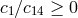 and
and  .
Considerations of positivity of energy impose the constraints
.
Considerations of positivity of energy impose the constraints 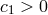 ,
, 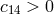 and
and
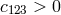 .
.
-
Khronometric theory;
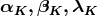
-
This is the low-energy limit of “Hořava gravity”, a proposal for a gravity theory that is power-counting renormalizable [190]. The vector field is required to be hypersurface orthogonal (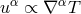 , where
, where 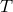 is a scalar field related to a preferred time direction;
equivalently the twist
is a scalar field related to a preferred time direction;
equivalently the twist ![ωαβ = ∇ [αuβ] + u [αa β]](article683x.gif) must vanish, where
must vanish, where 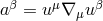 ), so
that higher spatial derivative terms could be introduced to effectuate renormalizability.
A “healthy” version of the theory [61, 62] can be shown to correspond to the values
), so
that higher spatial derivative terms could be introduced to effectuate renormalizability.
A “healthy” version of the theory [61, 62] can be shown to correspond to the values
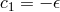
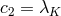 ,
, 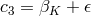 and
and 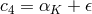 , where the limit
, where the limit 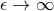 is to be
taken. (The idea is to extract
is to be
taken. (The idea is to extract  times
times 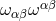 from the Einstein-Æther action and let
from the Einstein-Æther action and let
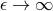 to enforce the twist-free condition [203].) In this case
to enforce the twist-free condition [203].) In this case 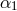 and
and 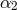 are given by
are given by
3.3.5 Tensor–vector–scalar (TeVeS) theories
This class of theories was invented to provide a fully relativistic theory of gravity that could mimic the
phenomenological behavior of so-called Modified Newtonian Dynamics (MOND). MOND is a
phenomenological mechanism [283] whereby Newton’s equation of motion 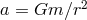 holds as long as
holds as long as
 is large compared to some fundamental scale
is large compared to some fundamental scale 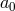 , but in a regime where
, but in a regime where 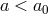 , the equation of
motion takes the form
, the equation of
motion takes the form 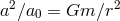 . With such a behavior, the rotational velocity of a
particle far from a central mass would have the form
. With such a behavior, the rotational velocity of a
particle far from a central mass would have the form 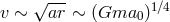 , thus reproducing
the flat rotation curves observed for spiral galaxies, without invoking a distribution of dark
matter.
, thus reproducing
the flat rotation curves observed for spiral galaxies, without invoking a distribution of dark
matter.
Devising a relativistic theory that would embody the MOND phenomenology turned out to be no simple
matter, and the final result, TeVeS was rather complicated [36]. Furthermore, it was shown to have
unexpected singular behavior that was most simply cured by incorporating features of the Einstein-Æther
theory [366]. The extended theory is based on an “Einstein” metric 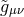 , related to the physical metric
, related to the physical metric
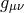 by
by
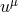 is a vector field, and
is a vector field, and 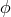 is a scalar field. The action for gravity is the standard GR action of
Eq. (31*), but defined using the Einstein metric
is a scalar field. The action for gravity is the standard GR action of
Eq. (31*), but defined using the Einstein metric 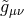 , while the matter action is that of a standard metric
theory, using
, while the matter action is that of a standard metric
theory, using 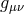 . These are supplemented by the vector action, given by that of Einstein-Æther theory,
Eq. (41*), and a scalar action, given by
where
. These are supplemented by the vector action, given by that of Einstein-Æther theory,
Eq. (41*), and a scalar action, given by
where 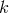 is a constant,
is a constant, 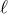 is a distance, and
is a distance, and 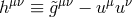 , indices being raised and lowered using
the Einstein metric. The function
, indices being raised and lowered using
the Einstein metric. The function 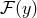 is chosen so that
is chosen so that 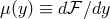 is unity in the
high-acceleration, or normal Newtonian and post-Newtonian regimes, and nearly zero in the MOND
regime.
is unity in the
high-acceleration, or normal Newtonian and post-Newtonian regimes, and nearly zero in the MOND
regime.
The PPN parameters of the theory [346] have the values 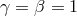 and
and 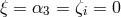 , while the
parameters
, while the
parameters 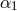 and
and 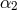 are given by
are given by
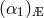 and
and 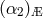 are given by Eqs. (46*) and (47*), where
where
are given by Eqs. (46*) and (47*), where
where 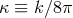 ,
and
,
and 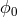 is the asymptotic value of the scalar field. In the limit
is the asymptotic value of the scalar field. In the limit 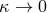 and
and 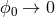 ,
, 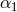 and
and 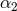 reduce to their Einstein-Æther forms.
reduce to their Einstein-Æther forms.
However, these PPN parameter values are computed in the limit where the function 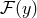 is a linear
function of its argument
is a linear
function of its argument 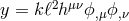 . When one takes into account the fact that the function
. When one takes into account the fact that the function
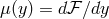 must interpolate between unity and zero to reach the MOND regime, it has been found
that the dynamics of local systems is more strongly affected by the fields of surrounding matter than was
anticipated. This “external field effect” (EFE) [284, 57, 58] produces a quadrupolar contribution to the
local Newtonian gravitational potential that depends on the external distribution of matter and on
the shape of the function
must interpolate between unity and zero to reach the MOND regime, it has been found
that the dynamics of local systems is more strongly affected by the fields of surrounding matter than was
anticipated. This “external field effect” (EFE) [284, 57, 58] produces a quadrupolar contribution to the
local Newtonian gravitational potential that depends on the external distribution of matter and on
the shape of the function 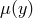 , and that can be significantly larger than the galactic tidal
contribution. Although the calculations of EFE have been carried out using phenomenological MOND
equations, it should be a generic phenomenon, applicable to TeVeS as well. Analysis of the orbit of
Saturn using Cassini data has placed interesting constraints on the MOND interpolating function
, and that can be significantly larger than the galactic tidal
contribution. Although the calculations of EFE have been carried out using phenomenological MOND
equations, it should be a generic phenomenon, applicable to TeVeS as well. Analysis of the orbit of
Saturn using Cassini data has placed interesting constraints on the MOND interpolating function
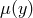 [186].
[186].
For thorough reviews of MOND and TeVeS, and their confrontation with the dark-matter paradigm, see [367, 150].
3.3.6 Quadratic gravity and Chern–Simons theories
Quadratic gravity is a recent incarnation of an old idea of adding to the action of GR terms quadratic in the Riemann and Ricci tensors or the Ricci scalar, as “effective field theory” models for more fundamental string or quantum gravity theories. The general action for such theories can be written as
where ,
, 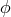 is a scalar field,
is a scalar field, 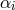 are dimensionless coupling constants (if the
functions
are dimensionless coupling constants (if the
functions 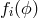 are dimensionless), and
are dimensionless), and 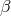 is a constant whose dimension depends on that of
is a constant whose dimension depends on that of
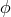 , and where
, and where 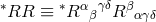 , where
, where 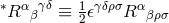 is the dual Riemann
tensor.
is the dual Riemann
tensor.
One challenge inherent in these theories is to find an argument or a mechanism that evades making the
natural choice for each of the 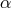 parameters to be of order unity. Such a choice makes the effects of
the additional terms essentially unobservable in most laboratory or astrophysical situations
because of the enormous scale of
parameters to be of order unity. Such a choice makes the effects of
the additional terms essentially unobservable in most laboratory or astrophysical situations
because of the enormous scale of 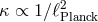 in the leading term. This class of theories is
too vast and diffuse to cover in this review, and no comprehensive review is available, to our
knowledge.
in the leading term. This class of theories is
too vast and diffuse to cover in this review, and no comprehensive review is available, to our
knowledge.
Chern–Simons gravity is the special case of this class of theories in which only the parity-violating term
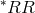 is present (
is present (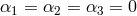 ) [201]. It can arise in various anomaly cancellation schemes in the
standard model of particle physics, in cancelling the Green–Schwarz anomaly in string theory, or in effective
field theories of inflation [408]. It can also arise in loop quantum gravity [382, 276]. The action in this case
is given by
) [201]. It can arise in various anomaly cancellation schemes in the
standard model of particle physics, in cancelling the Green–Schwarz anomaly in string theory, or in effective
field theories of inflation [408]. It can also arise in loop quantum gravity [382, 276]. The action in this case
is given by
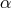 and
and 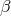 are coupling constants with dimensions
are coupling constants with dimensions 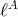 , and
, and 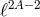 , assuming that the scalar field
has dimensions
, assuming that the scalar field
has dimensions 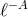 .
.
There are two different versions of Chern–Simons theory, a non-dynamical version in which
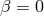 , so that
, so that 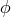 , given a priori as some specified function of spacetime, plays the role of a
Lagrange multiplier enforcing the constraint
, given a priori as some specified function of spacetime, plays the role of a
Lagrange multiplier enforcing the constraint 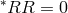 , and a dynamical version, in which
, and a dynamical version, in which
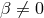 .
.
The PPN parameters for a non-dynamical version of the theory with 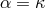 and
and 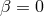 are identical
to those of GR; however, there is an additional, parity-even potential in the
are identical
to those of GR; however, there is an additional, parity-even potential in the 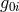 component of the metric
that does not appear in the standard PPN framework, given by
component of the metric
that does not appear in the standard PPN framework, given by
Einstein-Dilaton-Gauss–Bonnet gravity is another special case, in which the Chern–Simons term is
neglected (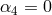 ), and the three other curvature-squared terms collapse to the Gauss–Bonnet
invariant,
), and the three other curvature-squared terms collapse to the Gauss–Bonnet
invariant, 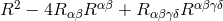 , i.e.
, i.e. 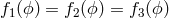 and
and 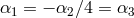 (see [292, 314]).
(see [292, 314]).
3.3.7 Massive gravity
Massive gravity theories attempt to give the putative “graviton” a mass. The simplest attempt to implement this in a ghost-free manner suffers from the so-called van Dam–Veltman–Zakharov (vDVZ) discontinuity [398, 453]. Because of the 3 additional helicity states available to the massive spin-2 graviton, the limit of small graviton mass does not coincide with pure GR, and the predicted perihelion advance, for example, violates experiment. A model theory by Visser [401*] attempts to circumvent the vDVZ problem by introducing a non-dynamical flat-background metric. This theory is truly continuous with GR in the limit of vanishing graviton mass; on the other hand, its observational implications have been only partially explored. Braneworld scenarios predict a tower or a continuum of massive gravitons, and may avoid the vDVZ discontinuity, although the full details are still a work in progress [125, 96]. Attempts to avert the vDVZ problem involve treating non-linear aspects of the theory at the fundamental level; many models incorporate a second tensor field in addition to the metric. For recent reviews, see [188, 123], and a focus issue in Vol. 30, Number 18 of Classical and Quantum Gravity.

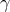
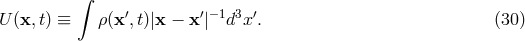
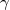
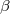
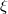
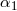
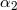
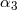
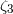
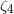
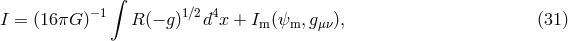
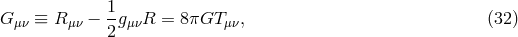
![∫ [ ] ( ) I&tidle;= (16 πG )−1 &tidle;R − 2 &tidle;gμν∂μφ∂ νφ − V (φ) (− &tidle;g)1∕2d4x + Im ψm, A2 (φ)&tidle;gμν , (33 )](article552x.gif)

![∫ −1 [ −1 μν 2 ] 1∕2 4 I = (16πG ) ϕR − ϕ ω (ϕ)g ∂μϕ∂ νϕ − ϕ V (− g) d x + Im (ψm, gμν), (35 )](article563x.gif)

![[ ] ϕdω∕d ϕ ω ≡ ω (ϕ0), λ ≡ (3-+-2ω)(4-+-2ω-) , (37 ) ϕ0](article565x.gif)

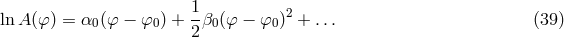
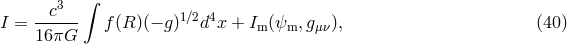
![∫ −1 [ μ μν α β μ ] 1∕2 4 I = (16 πG ) (1 + ωu μu )R − K αβ∇ μu ∇ νu + λ (uμu + 1) (− g) d x + Im(ψm, gμν), (41 )](article620x.gif)
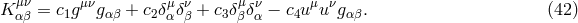
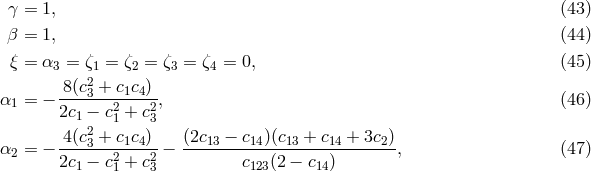
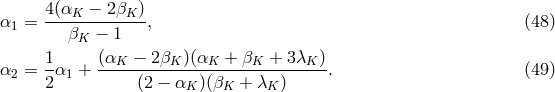

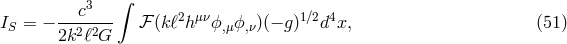
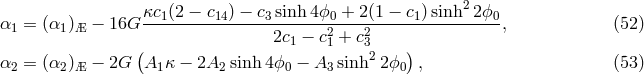

![∫ [ I = κR + α1f1(ϕ)R2 + α2f2 (ϕ)RαβR αβ + α3f3 (ϕ )RαβγδR αβγδ + α4f4 (ϕ)∗RR ( ) ] β μν 1∕2 4 − -- g ∂μϕ ∂νϕ + 2V (ϕ) (− g) d x + Im (ψm,gμν), (58 ) 2](article735x.gif)
![∫ [ α β( ) ] I = κR + --ϕ∗RR − -- gμν∂μϕ ∂νϕ + 2V (ϕ) (− g)1∕2d4x + Im(ψm, gμν), (59 ) 4 2](article748x.gif)