List of Footnotes
| 1 | We work in geometrised units throughout. | |
| 2 | Of course, a charged extremal black hole can always discharge in the presence of charged matter. | |
| 3 | The emergence of an AdS3 factor for solutions with certain null singularities has been previously observed [68, 18 ]. We
will only consider regular horizons, so the area of spatial cross sections of the horizon is necessarily non-zero. ]. We
will only consider regular horizons, so the area of spatial cross sections of the horizon is necessarily non-zero. |
|
| 4 | Although spacetimes correspond to Lorentzian metrics, one can often analytically continue these to complete
Riemannian metrics. Indeed, the first example of an inhomogeneous Einstein metric on a compact manifold was
found by Page, by taking a certain limit of the Kerr–de Sitter metrics [185 ], giving a metric on ], giving a metric on 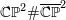 . . |
|
| 5 | Albeit, under some technical assumptions such as analyticity of the metric. | |
| 6 | Two oriented manifolds are said to be oriented-cobordant if there exists some other oriented manifold whose boundary (with the induced orientation) is their disjoint union. | |
| 7 | Similarly, any such black hole in Einstein–Maxwell-dilaton theory with a purely electric field strength must be given by the RN solution [96, 97]. | |
| 8 | Indeed counterexamples are known in both senses. | |
| 9 | In fact our constructions only assume the metric is 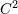 in a neighbourhood of the horizon. This encompasses certain
examples of multi–black-hole spacetimes with non-smooth horizons [33]. in a neighbourhood of the horizon. This encompasses certain
examples of multi–black-hole spacetimes with non-smooth horizons [33]. |
|
| 10 | To avoid proliferation of indices we will denote both coordinate and vielbein indices on 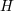 by lower case latin letters by lower case latin letters
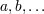 . . |
|
| 11 | A Kundt spacetime is one that admits a null geodesic vector field with vanishing expansion, shear and twist. | |
| 12 | A Kundt spacetime is said to be degenerate if the Riemann tensor and all its covariant derivatives are type II with respect
to the defining null vector field [184 ]. ]. |
|
| 13 | The remaining components of the Einstein equations for the full spacetime restricted to 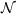 give equations for extrinsic
data (i.e., give equations for extrinsic
data (i.e.,  -derivatives of -derivatives of 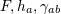 that do not appear in the near-horizon geometry). On the other hand, the rest of the
Einstein equations for the near-horizon geometry restricted to the horizon vanish. that do not appear in the near-horizon geometry). On the other hand, the rest of the
Einstein equations for the near-horizon geometry restricted to the horizon vanish. |
|
| 14 | The Komar integral associated with the null generator of the horizon 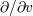 vanishes identically. In fact, one can show
that for a general non-extremal Killing horizon, this integral is merely proportional to vanishes identically. In fact, one can show
that for a general non-extremal Killing horizon, this integral is merely proportional to  . . |
|
| 15 | The borderline case of 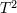 topology was only excluded later using topological censorship [44]. Furthermore, these results
were generalised to the non-stationary case and asymptotically AdS case [90]. topology was only excluded later using topological censorship [44]. Furthermore, these results
were generalised to the non-stationary case and asymptotically AdS case [90]. |
|
| 16 | A borderline case also arises in this proof, corresponding to the induced metric on  being Ricci flat. This was in fact
later excluded [88]. being Ricci flat. This was in fact
later excluded [88]. |
|
| 17 | An isometry group whose surfaces of transitivity are 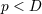 dimensional is said to be orthogonally transitive if there
exists dimensional is said to be orthogonally transitive if there
exists 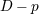 dimensional surfaces orthogonal to the surfaces of transitivity at every point. dimensional surfaces orthogonal to the surfaces of transitivity at every point. |
|
| 18 | This can be obtained by analytically continuing Eq. (74 ) by ) by 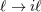 . . |
|
| 19 | A complex manifold is Fano if its first Chern class is positive, i.e., 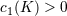 . It follows that any Kähler–Einstein
metric on such a manifold must have positive Einstein constant. . It follows that any Kähler–Einstein
metric on such a manifold must have positive Einstein constant. |
|
| 20 | This is a conserved charge for such asymptotically KK spacetimes. | |
| 21 | Similarly, higher-dimensional pure-AdS spacetime is unstable to the formation of small black holes [27]. | |
| 22 | This stems from the fact that such spacetimes admit null geodesic congruences with vanishing expansion, rotation, and shear (i.e., they are Kundt spacetimes and hence algebraically special). | |
| 23 | We would like to thank Carmen Li for verifying this. | |
| 24 | There is a vast literature on this problem, which we will not attempt to review here. |

