6 Solutions with Gauge Fields
In this section we will consider general near-horizon geometries coupled to non-trivial gauge fields. We will mostly focus on theories that are in the bosonic sector of minimal supergravity theories (since these are the best understood cases). Extremal, non-supersymmetric, near-horizon geometries may be thought of as interpolating between vacuum and supersymmetric solutions. They consist of a much larger class of solutions, which, at least in higher dimensions, are much more difficult to classify. In particular, we consider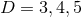 Einstein–Maxwell theory, possibly coupled to a Chern–Simons term in odd dimensions, and
Einstein–Maxwell theory, possibly coupled to a Chern–Simons term in odd dimensions, and
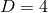 Einstein–Yang–Mills theory.
Einstein–Yang–Mills theory.
6.1 Three dimensional Einstein–Maxwell–Chern–Simons theory
The classification of near-horizon geometries in 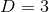 Einstein–Maxwell theory with a cosmological
constant can be completely solved. To the best of our knowledge this has not been presented before, so for
completeness we include it here. It should be noted though that partial results which capture the main
result were previously shown in [175].
Einstein–Maxwell theory with a cosmological
constant can be completely solved. To the best of our knowledge this has not been presented before, so for
completeness we include it here. It should be noted though that partial results which capture the main
result were previously shown in [175].
The method parallels the vacuum case in Section 4.2 closely. As in that case the near-horizon metric
data reads 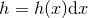 and
and 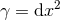 . Since cross sections
. Since cross sections 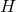 of the horizon are one-dimensional, the
Maxwell 2-form induced on
of the horizon are one-dimensional, the
Maxwell 2-form induced on 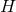 must vanish identically. Hence, the most general near-horizon Maxwell field
(23
must vanish identically. Hence, the most general near-horizon Maxwell field
(23 ) in three dimensions is
) in three dimensions is 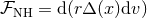 . It is straightforward to show that the 3D Maxwell
equation
. It is straightforward to show that the 3D Maxwell
equation 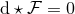 , where
, where  is the Hodge dual with respect to the spacetime metric, is equivalent to
the following equation on
is the Hodge dual with respect to the spacetime metric, is equivalent to
the following equation on 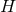 :
:
 ) and (18
) and (18 ) are simply
) are simply
Theorem 6.1. Consider a near-horizon geometry with a compact horizon cross section 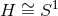 in Einstein–Maxwell-
in Einstein–Maxwell- theory. If
theory. If 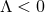 the near-horizon geometry must be either
the near-horizon geometry must be either 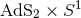 with a constant AdS2 Maxwell field, or the quotient of AdS3 Eq. (73
with a constant AdS2 Maxwell field, or the quotient of AdS3 Eq. (73 ) with a vanishing Maxwell field.
If
) with a vanishing Maxwell field.
If 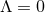 the only solution is the trivial flat near-horizon geometry
the only solution is the trivial flat near-horizon geometry 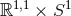 . If
. If 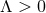 there
are no solutions.
there
are no solutions.
Proof: Rather that solving the above ODEs we may use a global argument. Compactness requires 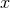 to be
a periodic coordinate on
to be
a periodic coordinate on  and since
and since 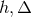 are globally defined they must be periodic functions of
are globally defined they must be periodic functions of
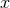 . For
. For 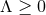 simply integrate Eq. (100
simply integrate Eq. (100 ) over
) over 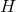 to find that the only solution is the trivial flat one
to find that the only solution is the trivial flat one
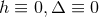 and
and 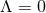 . For
. For 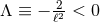 we may argue as follows. Multiply Eq. (100
we may argue as follows. Multiply Eq. (100 ) by
) by 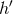 and
integrate over
and
integrate over 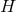 to obtain
to obtain
 ). Hence
). Hence 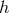 must be a constant
and
must be a constant
and 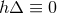 . Equation (100
. Equation (100 ) then implies
) then implies 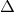 is also a constant. We deduce the only possible solutions
are
is also a constant. We deduce the only possible solutions
are 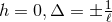 , or
, or 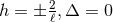 . The former gives a near-horizon geometry
. The former gives a near-horizon geometry 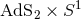 and the
latter is the vacuum solution locally isometric to AdS3.
and the
latter is the vacuum solution locally isometric to AdS3.
This result implies that the near-horizon limit of any charged rotating black-hole solution to 3D
Einstein–Maxwell-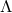 theory either has vanishing charge or angular momentum. The
theory either has vanishing charge or angular momentum. The 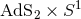 solution
is the near-horizon limit of the non-rotating extremal charged BTZ black hole, whereas the AdS3
solution is the near-horizon limit of the vacuum rotating extremal BTZ [15]. Charged rotating
black holes were first obtained within a wide class of stationary and axisymmetric solutions to
Einstein–Maxwell-
solution
is the near-horizon limit of the non-rotating extremal charged BTZ black hole, whereas the AdS3
solution is the near-horizon limit of the vacuum rotating extremal BTZ [15]. Charged rotating
black holes were first obtained within a wide class of stationary and axisymmetric solutions to
Einstein–Maxwell-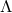 theory [45], and later by applying a solution generating technique to the charged
non-rotating black hole [46, 174]. We have checked that in the extremal limit their near-horizon
geometry is the
theory [45], and later by applying a solution generating technique to the charged
non-rotating black hole [46, 174]. We have checked that in the extremal limit their near-horizon
geometry is the 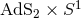 solution, so the angular momentum is lost in the near-horizon limit,
in agreement with the above analysis. It would be interesting to investigate whether charged
rotating black holes exist that instead possess a locally AdS3 near-horizon geometry. In this case,
charge would not be captured by the near-horizon geometry, a phenomenon that is known to
occur for five-dimensional supersymmetric black rings whose near-horizon geometry is locally
solution, so the angular momentum is lost in the near-horizon limit,
in agreement with the above analysis. It would be interesting to investigate whether charged
rotating black holes exist that instead possess a locally AdS3 near-horizon geometry. In this case,
charge would not be captured by the near-horizon geometry, a phenomenon that is known to
occur for five-dimensional supersymmetric black rings whose near-horizon geometry is locally
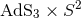 .
.
In 2 + 1 dimensions an Abelian gauge field monopole is not isolated. Electric charge can be “screened”
by adding a mass term to the gauge field. A natural way to do this is to add a Chern–Simons term
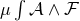 to the spacetime action, resulting in a topologically-massive gauge theory. This only modifies
the Maxwell equation:
to the spacetime action, resulting in a topologically-massive gauge theory. This only modifies
the Maxwell equation:
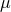 is the mass parameter of the gauge field. For the near-horizon Maxwell field it can be shown that
this is equivalent to
As in the pure Einstein–Maxwell case, a complete classification of near-horizon geometries to this theory is
possible. To the best of our knowledge this has not been presented before.
is the mass parameter of the gauge field. For the near-horizon Maxwell field it can be shown that
this is equivalent to
As in the pure Einstein–Maxwell case, a complete classification of near-horizon geometries to this theory is
possible. To the best of our knowledge this has not been presented before.
Theorem 6.2. Consider a near-horizon geometry with a cross section 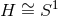 in Einstein–Maxwell-
in Einstein–Maxwell-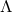 theory with a Chern–Simons term and mass
theory with a Chern–Simons term and mass 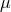 . If
. If 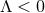 the functions
the functions
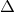 and
and 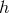 are constant and the near-horizon geometry is the homogeneous
are constant and the near-horizon geometry is the homogeneous 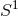 -bundle over AdS
2
(106
-bundle over AdS
2
(106 ). If
). If 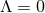 the only solution is the trivial flat near-horizon geometry
the only solution is the trivial flat near-horizon geometry 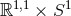 . If
. If 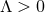 there are no solutions.
there are no solutions.
For 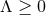 the proof of this is identical to the Einstein–Maxwell case above. For
the proof of this is identical to the Einstein–Maxwell case above. For 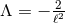 one can
also use the same argument as the Einstein–Maxwell case. Using the horizon equation (100
one can
also use the same argument as the Einstein–Maxwell case. Using the horizon equation (100 ) and Maxwell
equation (104
) and Maxwell
equation (104 ) one can show
) one can show
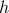 must be a constant and
must be a constant and 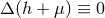 . The horizon equation then implies
. The horizon equation then implies  is a
constant. If
is a
constant. If  one gets the vacuum AdS3 solution. If
one gets the vacuum AdS3 solution. If  then
then 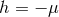 and
and 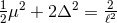 .
The rest of the near-horizon data is given by
.
The rest of the near-horizon data is given by 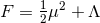 and hence the near-horizon geometry is
and
and hence the near-horizon geometry is
and 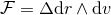 . Note that if
. Note that if 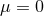 we recover the
we recover the 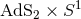 solution, whereas if
solution, whereas if 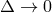 we
recover the vacuum AdS3 solution.
we
recover the vacuum AdS3 solution.
This implies that the near-horizon geometry of any charged rotating black-hole solution to this theory is
either the vacuum AdS3 solution, or the non-trivial solution (106 ), which is sometimes referred to as
“warped AdS3”. Examples of charged rotating black-hole solutions in this theory have been
found [2].
), which is sometimes referred to as
“warped AdS3”. Examples of charged rotating black-hole solutions in this theory have been
found [2].
6.2 Four dimensional Einstein–Maxwell theory
The spacetime Einstein–Maxwell equations are Eqs. (15 ), (22
), (22 ) with
) with 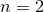 and
and 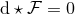 , where
, where  is
the Hodge dual with respect to the spacetime metric, and the Bianchi identity
is
the Hodge dual with respect to the spacetime metric, and the Bianchi identity 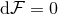 . The near-horizon
Maxwell field is given by (23
. The near-horizon
Maxwell field is given by (23 ). The near-horizon geometry Einstein–Maxwell equations are given by
Eqs. (17
). The near-horizon geometry Einstein–Maxwell equations are given by
Eqs. (17 ) and (18
) and (18 ), where
), where
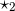 is the Hodge dual with respect to the horizon metric
is the Hodge dual with respect to the horizon metric 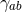 . Observe that
. Observe that 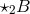 is a function on
is a function on
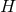 .
.
Static near-horizon geometries have been completely classified. For 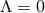 this was first derived in [43
this was first derived in [43 ],
and generalised to
],
and generalised to 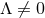 in [154
in [154 ].
].
Theorem 6.3 ([43, 154 ]). Consider a
static near-horizon geometry in
]). Consider a
static near-horizon geometry in 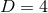 Einstein–Maxwell-
Einstein–Maxwell-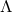 theory, with compact horizon cross
section
theory, with compact horizon cross
section 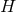 . For
. For 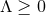 it must be
it must be 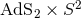 . For
. For 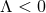 it must be
it must be 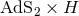 where
where 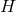 is one of the constant curvature surfaces
is one of the constant curvature surfaces 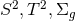 .
.
It is worth remarking that if one removes the assumption of compactness one can still completely classify near-horizon geometries. The extra solution one obtains can be written as a warped product
where , which is an analyticaly continued
Reissner–Nordström-
, which is an analyticaly continued
Reissner–Nordström-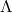 solution.
solution.
Non-static near-horizon geometries are not fully classified, except under the additional assumption of axisymmetry.
Theorem 6.4 ([163 , 154
, 154 ]). Any axisymmetric, non-static near-horizon geometry in
]). Any axisymmetric, non-static near-horizon geometry in 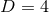 Einstein–Maxwell-
Einstein–Maxwell-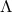 theory, with a compact horizon cross section, must be given by the near-horizon
geometry of an extremal Kerr–Newman-
theory, with a compact horizon cross section, must be given by the near-horizon
geometry of an extremal Kerr–Newman-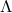 black hole.
black hole.
[163] solved the 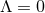 in the context of isolated degenerate horizons, where the same
equations on
in the context of isolated degenerate horizons, where the same
equations on 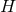 arise. [154
arise. [154 ] solved the case with
] solved the case with 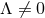 . Note that the horizon topology theorem
excludes the possibility of toroidal horizon cross sections for
. Note that the horizon topology theorem
excludes the possibility of toroidal horizon cross sections for 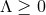 . [164
. [164 ] also excluded the
possibility of a toroidal horizon cross section if
] also excluded the
possibility of a toroidal horizon cross section if 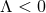 under the assumptions of the above
theorem.
under the assumptions of the above
theorem.
It is worth noting that the results presented in this section, as well as the techniques used to establish them, are entirely analogous to the vacuum case presented in Section 4.3.
6.3 Five dimensional Einstein–Maxwell–Chern–Simons theory
The field equations of 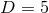 Einstein–Maxwell theory coupled to a Chern–Simons term are given by
Eqs. (15
Einstein–Maxwell theory coupled to a Chern–Simons term are given by
Eqs. (15 ) and (22
) and (22 ) with
) with 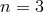 and
and
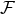 is the Maxwell two form and
is the Maxwell two form and 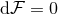 . The cases of most interest are
. The cases of most interest are 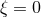 and
and 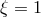 ,
which correspond to pure Einstein–Maxwell theory and the bosonic sector of minimal supergravity
respectively. The near-horizon Maxwell field is given by (23
,
which correspond to pure Einstein–Maxwell theory and the bosonic sector of minimal supergravity
respectively. The near-horizon Maxwell field is given by (23 ). The corresponding near-horizon geometry
equations are given by Eqs. (17
). The corresponding near-horizon geometry
equations are given by Eqs. (17 ) and (18
) and (18 ) and
where
) and
where 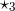 is the Hodge dual with respect to the 3D horizon metric
is the Hodge dual with respect to the 3D horizon metric 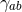 . In this section we summarise
what is known about solutions to these equations, which is mostly restricted to the
. In this section we summarise
what is known about solutions to these equations, which is mostly restricted to the 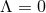 case. Hence,
unless otherwise stated, we assume
case. Hence,
unless otherwise stated, we assume 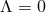 in this section.
in this section.
A number of new complications arise that render the classification problem more difficult, most
obviously the lack of electro-magnetic duality. Therefore, purely electric solutions, which correspond to
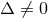 and
and 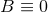 , are qualitatively different to purely magnetic solutions, which correspond to
, are qualitatively different to purely magnetic solutions, which correspond to 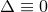 and
and 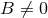 .
.
6.3.1 Static
Perhaps somewhat surprisingly a complete classification of static near-horizon geometries in this theory has
not yet been achieved. Nevertheless, a number of results have been proved under various extra assumptions.
All the results summarised in this section were proved in [153 ].
].
As in other five dimensional near-horizon geometry classifications, the assumption of 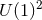 rotational
symmetry proves to be useful. Static near-horizon geometries in this class in general are either
warped products of AdS2 and
rotational
symmetry proves to be useful. Static near-horizon geometries in this class in general are either
warped products of AdS2 and 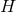 , or AdS3 and a 2D manifold, see the Corollary 3.1. The
AdS3 near-horizon geometries are necessarily purely magnetic and can be classified for any
, or AdS3 and a 2D manifold, see the Corollary 3.1. The
AdS3 near-horizon geometries are necessarily purely magnetic and can be classified for any
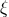 .
.
Proposition 6.1. Any static AdS3 near-horizon geometry with a 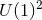 -rotational symmetry, in
Einstein–Maxwell–Chern–Simons theory, with a compact horizon cross section, is the direct product
of a quotient of AdS3 and a round
-rotational symmetry, in
Einstein–Maxwell–Chern–Simons theory, with a compact horizon cross section, is the direct product
of a quotient of AdS3 and a round 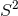 .
.
This classifies a subset of purely magnetic geometries. By combining the results of [153 ] together with
Proposition 6.4 of [155
] together with
Proposition 6.4 of [155 ], it can be deduced that for
], it can be deduced that for 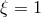 there are no purely magnetic AdS2
geometries; therefore, with these symmetries, one has a complete classification of purely magnetic
geometries.
there are no purely magnetic AdS2
geometries; therefore, with these symmetries, one has a complete classification of purely magnetic
geometries.
Corollary 6.1. Any static, purely magnetic, near-horizon geometry in minimal supergravity,
possessing 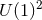 -rotational symmetry and compact cross sections
-rotational symmetry and compact cross sections 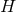 , must be locally isometric
to
, must be locally isometric
to 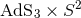 with
with 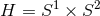 .
.
We now turn to purely electric geometries.
Proposition 6.2. Consider a static, purely electric, near-horizon geometry
in Einstein–Maxwell–Chern–Simons theory, with a 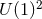 -rotational symmetry and compact cross
section. It must be given by either
-rotational symmetry and compact cross
section. It must be given by either 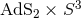 , or a warped product of AdS
2 and an inhomogeneous
, or a warped product of AdS
2 and an inhomogeneous
 .
.
The latter non-trivial solution is in fact the near-horizon limit of an extremal RN black hole immersed in a background electric field (this can be generated via a Harrison type transformation).
Finally, we turn to the case where the near-horizon geometry possess both electric and magnetic fields. In fact one can prove a general result in this case, i.e., without the assumption of rotational symmetries.
Proposition 6.3. Any static near-horizon geometry with compact cross sections 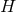 , in
Einstein–Maxwell–Chern–Simons theory with coupling
, in
Einstein–Maxwell–Chern–Simons theory with coupling 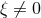 , with non-trivial electric and magnetic
fields, is a direct product of
, with non-trivial electric and magnetic
fields, is a direct product of 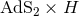 , where the metric on
, where the metric on 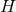 is not Einstein.
is not Einstein.
Explicit examples for 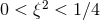 were also found, which all have
were also found, which all have 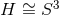 with
with 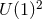 -rotational
symmetry. However, we should emphasise that no examples are known for minimal supergravity
-rotational
symmetry. However, we should emphasise that no examples are known for minimal supergravity 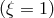 .
Hence there is the possibility that in this case static near-horizon geometries with non-trivial electric and
magnetic fields do not exist, although this has not yet been shown. If this is the case, then the above results
fully classify static near-horizon geometries with
.
Hence there is the possibility that in this case static near-horizon geometries with non-trivial electric and
magnetic fields do not exist, although this has not yet been shown. If this is the case, then the above results
fully classify static near-horizon geometries with 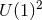 -rotational symmetry. For
-rotational symmetry. For 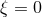 the analysis of
electro-magnetic geometries is analogous to the purely magnetic case above; in fact there exists a dyonic
AdS2 geometry that is a direct product
the analysis of
electro-magnetic geometries is analogous to the purely magnetic case above; in fact there exists a dyonic
AdS2 geometry that is a direct product 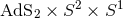 and it is conjectured there are no
others.
and it is conjectured there are no
others.
6.3.2 Homogeneous
The classification of homogeneous near-horizon geometries can be completely solved, even including a
cosmological constant 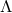 . This does not appear to have been presented explicitly before, so for
completeness we include it here with a brief derivation. We may define a homogeneous near-horizon
geometry as follows. The Riemannian manifold
. This does not appear to have been presented explicitly before, so for
completeness we include it here with a brief derivation. We may define a homogeneous near-horizon
geometry as follows. The Riemannian manifold 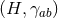 is a homogeneous space, i.e., admits a
transitive isometry group
is a homogeneous space, i.e., admits a
transitive isometry group 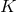 , such that the rest of the near-horizon data
, such that the rest of the near-horizon data 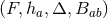 are invariant under
are invariant under 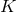 . As discussed in Section (4.4), this is equivalent to the near-horizon
geometry being a homogeneous spacetime with a Maxwell field invariant under its isometry
group.
. As discussed in Section (4.4), this is equivalent to the near-horizon
geometry being a homogeneous spacetime with a Maxwell field invariant under its isometry
group.
An immediate consequence of homogeneity is that an invariant function must be a constant and any
invariant 1-form must be a Killing field. Hence the 1-forms 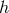 and
and 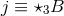 are Killing and the functions
are Killing and the functions
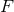 ,
, 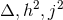 must be constants. Thus, the horizon Einstein equations (17
must be constants. Thus, the horizon Einstein equations (17 ), (18
), (18 ), (113
), (113 ), (114
), (114 ) and
Maxwell equation (115
) and
Maxwell equation (115 ) simplify.
) simplify.
Firstly, note that if 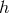 and
and 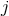 vanish identically then
vanish identically then 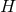 is Einstein
is Einstein 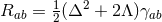 , so
, so 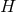 is a constant curvature space
is a constant curvature space 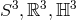 (the latter two can only occur if
(the latter two can only occur if 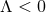 ). This family includes
the static near-horizon geometry
). This family includes
the static near-horizon geometry 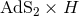 .
.
Now consider the case where at least one of 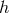 and
and 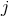 is non-vanishing. By contracting the
Maxwell equation (115
is non-vanishing. By contracting the
Maxwell equation (115 ) with
) with 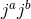 one can show that
one can show that 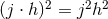 and hence by the
Cauchy–Schwarz inequality
and hence by the
Cauchy–Schwarz inequality 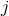 and
and  must be parallel as long as they are both non-zero. Thus, if one
of
must be parallel as long as they are both non-zero. Thus, if one
of 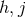 is non-vanishing, we can write
is non-vanishing, we can write 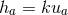 and
and 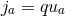 for some constants
for some constants 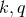 where
where 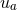 is a unit normalised Killing vector field. The near-horizon equations now reduce to
is a unit normalised Killing vector field. The near-horizon equations now reduce to
Theorem 6.5. Any homogeneous near-horizon geometry in Einstein–Maxwell–CS-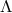 theory for which
one of the constants
theory for which
one of the constants 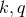 is non-zero, must be locally isometric to
is non-zero, must be locally isometric to
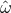 is a
is a 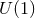 -connection over a 2D base with metric
-connection over a 2D base with metric  satisfying
satisfying 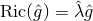 , with
, with
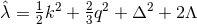 , and
, and 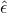 is the volume form of the 2D base. The curvature of the connection is
is the volume form of the 2D base. The curvature of the connection is
![dωˆ = [k2 − 4q2 + Δ2 + 2Λ ]1∕2ˆ𝜖 3](article1351x.gif) and
and 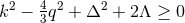 . If
. If 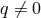 the constants must satisfy
whereas if
the constants must satisfy
whereas if 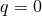 one must have
one must have 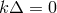 .
.
The proof of this proceeds as in the vacuum case, by reducing the horizon equations to the 2D orbit
space defined by the Killing field 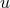 . The above result contains a number of special cases of interest,
which we now elaborate upon. Firstly, note that
. The above result contains a number of special cases of interest,
which we now elaborate upon. Firstly, note that  reduces to the vacuum case, see
Theorem 4.4.
reduces to the vacuum case, see
Theorem 4.4.
Before discussing the general case consider  , so the near-horizon geometry is static, which
connects to Section 6.3.1. The constraint on the parameters is
, so the near-horizon geometry is static, which
connects to Section 6.3.1. The constraint on the parameters is 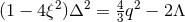 and hence, if
and hence, if
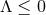 one must have
one must have 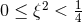 . For
. For 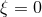 the connection is trivial and hence the near-horizon
geometry is locally isometric to the dyonic
the connection is trivial and hence the near-horizon
geometry is locally isometric to the dyonic 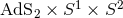 solution. For
solution. For  we get
examples of the geometries in Proposition (6.3), where
we get
examples of the geometries in Proposition (6.3), where 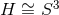 with its standard homogeneous
metric.
with its standard homogeneous
metric.
Now we consider  in generality so at least one of
in generality so at least one of 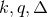 is non-zero, in which case
is non-zero, in which case 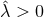 and
so
and
so 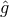 is the round
is the round 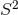 . The horizon is then either
. The horizon is then either 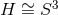 with its homogeneous metric or
with its homogeneous metric or
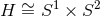 , depending on whether the connection
, depending on whether the connection 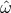 is non-trivial or not, respectively. Notably we
have:
is non-trivial or not, respectively. Notably we
have:
Corollary 6.2. Any homogeneous near-horizon geometry of minimal supergravity is locally isometric
to 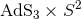 , or the near-horizon limit of either (i) the BMPV black hole (including
, or the near-horizon limit of either (i) the BMPV black hole (including 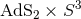 ),
or (ii) an extremal nonsupersymmetric charged black hole with
),
or (ii) an extremal nonsupersymmetric charged black hole with 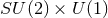 rotational symmetry.
rotational symmetry.
The proof of this follows immediately from Theorem 6.5 with 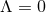 and
and 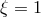 . In this case the
constraint on the parameters factorises to give two branches of possible solutions (a)
. In this case the
constraint on the parameters factorises to give two branches of possible solutions (a) 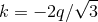 or (b)
or (b)
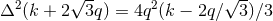 . Case (a) gives two solutions. If
. Case (a) gives two solutions. If 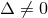 it must have
it must have
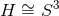 and corresponds to the BMPV solution (i) (for
and corresponds to the BMPV solution (i) (for 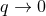 this reduces to
this reduces to 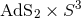 ),
whereas if
),
whereas if 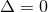 it is the
it is the 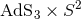 solution with
solution with 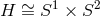 . Case (b) also gives
two solutions. If
. Case (b) also gives
two solutions. If 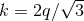 then
then 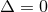 , which gives
, which gives 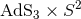 with
with 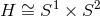 ,
otherwise we get solution (ii) with
,
otherwise we get solution (ii) with 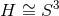 . Note that solution (ii) reduces to the vacuum case as
. Note that solution (ii) reduces to the vacuum case as
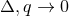 .
.
The black-hole solution (ii) may be constructed as follows. A charged generalisation of the MP black
hole can be generated in minimal supergravity [50]. Generically, the extremal limit depends on 3-parameters
with two independent angular momenta 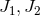 and
and 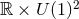 symmetry. Setting
symmetry. Setting 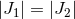 gives two
distinct branches of 2-parameter extremal black-hole solutions with enhanced
gives two
distinct branches of 2-parameter extremal black-hole solutions with enhanced 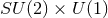 rotational symmetry corresponding to the BMPV solution (i) (which reduces to the RN solution if
rotational symmetry corresponding to the BMPV solution (i) (which reduces to the RN solution if
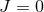 ) or solution (ii) (which reduces to the vacuum extremal MP black hole in the neutral
limit).
) or solution (ii) (which reduces to the vacuum extremal MP black hole in the neutral
limit).
It is interesting to note the analogous result for pure Einstein–Maxwell theory:
Corollary 6.3. Any homogeneous near-horizon geometry of Einstein–Maxwell theory is locally isometric to either (i)
or (ii) This also follows from application of Theorem 6.5 with 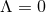 and
and 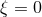 . Solution (i) for
. Solution (i) for 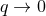 reduces to the vacuum solution of Theorem 4.4, whereas for
reduces to the vacuum solution of Theorem 4.4, whereas for 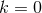 , it gives the static dyonic
, it gives the static dyonic
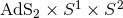 solution. Solution (ii) for
solution. Solution (ii) for 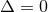 gives
gives 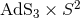 , whereas for
, whereas for 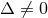 it gives a
near-horizon geometry with
it gives a
near-horizon geometry with 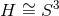 , which for
, which for 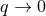 is
is 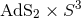 . A charged rotating black-hole
solution to Einstein–Maxwell theory generalising MP is not known explicitly. Hence this corollary could be
of use for constructing such an extremal charged rotating black hole with
. A charged rotating black-hole
solution to Einstein–Maxwell theory generalising MP is not known explicitly. Hence this corollary could be
of use for constructing such an extremal charged rotating black hole with 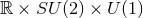 symmetry.
symmetry.
For 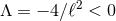 there are even more possibilities, since
there are even more possibilities, since 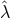 may be positive, zero, or negative. One
then gets near-horizon geometries that generically have
may be positive, zero, or negative. One
then gets near-horizon geometries that generically have 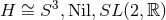 , respectively, equipped
with their standard homogeneous metrics. Each of these may have a degenerate limit with
, respectively, equipped
with their standard homogeneous metrics. Each of these may have a degenerate limit with
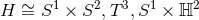 , as occurs in the vacuum and supersymmetric cases. The full space of solutions
interpolates between the vacuum case given in Corollary 4.2, and the supersymmetric near-horizon
geometries of gauged supergravity of Proposition 5.3. For example, the supersymmetric horizons [120
, as occurs in the vacuum and supersymmetric cases. The full space of solutions
interpolates between the vacuum case given in Corollary 4.2, and the supersymmetric near-horizon
geometries of gauged supergravity of Proposition 5.3. For example, the supersymmetric horizons [120 ]
correspond to
]
correspond to 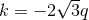 and
and 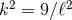 with
with 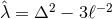 . We will not investigate the full space of
solutions in detail here.
. We will not investigate the full space of
solutions in detail here.
6.3.3 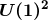 -rotational symmetry
-rotational symmetry
The classification of near-horizon geometries in 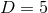 Einstein–Maxwell–CS theory, under the
assumption of
Einstein–Maxwell–CS theory, under the
assumption of 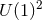 symmetry, turns out to be significantly more complicated than the vacuum case.
This is unsurprising; solutions may carry two independent angular momenta, electric charge and
dipole/magnetic charge (depending on the spacetime asymptotics). As a result, there are several ways for a
black hole to achieve extremality. Furthermore, such horizons may be deformed by background electric
fields [153].
symmetry, turns out to be significantly more complicated than the vacuum case.
This is unsurprising; solutions may carry two independent angular momenta, electric charge and
dipole/magnetic charge (depending on the spacetime asymptotics). As a result, there are several ways for a
black hole to achieve extremality. Furthermore, such horizons may be deformed by background electric
fields [153].
In the special case of minimal supergravity one can show:
Proposition 6.4 ([155 ]). Any near-horizon geometry of minimal supergravity
with
]). Any near-horizon geometry of minimal supergravity
with 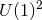 -rotational symmetry takes the form of Eqs. (58
-rotational symmetry takes the form of Eqs. (58 ) and (62
) and (62 ), where the functional form
of
), where the functional form
of 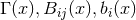 can be fully determined in terms of rational functions of
can be fully determined in terms of rational functions of 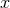 . In particular,
. In particular,
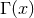 is a quadratic function.
is a quadratic function.
The method of proof is discussed in Section 6.4. Although this solves the problem in principle, it turns out that in practice it is very complicated to disentangle the constraints on the constants that specify the solution. Hence an explicit classification of all possible solutions has not yet been obtained, although in principle it is contained in the above result.
We now summarise all known examples of five dimensional non-static near-horizon geometries with
non-trivial gauge fields, which arise as near-horizon limits of known black hole or black string solutions. All
these examples possess  rotational symmetry and hence fall into the class of solutions covered by
Theorem 3.5, so the near-horizon metric and field strength
rotational symmetry and hence fall into the class of solutions covered by
Theorem 3.5, so the near-horizon metric and field strength  take the form of Eqs. (58
take the form of Eqs. (58 ) and (62
) and (62 )
respectively. We will divide them by horizon topology.
)
respectively. We will divide them by horizon topology.
Spherical topology
Charged Myers–Perry black holes: This asymptotically-flat solution is known explicitly only for minimal
supergravity  (in particular it is not known in pure Einstein–Maxwell
(in particular it is not known in pure Einstein–Maxwell  ), since it can be
constructed by a solution-generating procedure starting with the vacuum MP solution. It depends on four
parameters
), since it can be
constructed by a solution-generating procedure starting with the vacuum MP solution. It depends on four
parameters 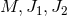 , and
, and 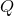 corresponding to the ADM mass, two independent angular momenta and
an electric charge. The extremal limit generically depends on three parameters and gives a near-horizon
geometry with
corresponding to the ADM mass, two independent angular momenta and
an electric charge. The extremal limit generically depends on three parameters and gives a near-horizon
geometry with 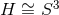 .
.
Charged Kaluza–Klein black holes: The most general known solution to date was found in [204]
(see references therein for a list of previously known solutions) and carries a mass, two
independent angular momenta, a KK monopole charge, an electric charge and a ‘magnetic
charge’20.
The extremal limit will generically depend on five-parameters, however, as for the vacuum case the extremal
locus must have more than one connected component. These solutions give a large family of near-horizon
geometries with 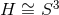 .
.
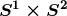 topology
topology
Supersymmetric black rings and strings: The asymptotically-flat supersymmetric black ring [64] and the
supersymmetric Taub–NUT black ring [65] both have a near-horizon geometry that is locally
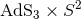 . There are also supersymmetric black string solutions with such near-horizon
geometries [93, 19].
. There are also supersymmetric black string solutions with such near-horizon
geometries [93, 19].
Dipole black rings: The singly-spinning dipole black ring [69 ] is a solution to Einstein–Maxwell–CS for all
] is a solution to Einstein–Maxwell–CS for all
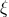 . It is a 3-parameter family with a single angular momentum and dipole charge possessing a 2-parameter
extremal limit. The resulting near-horizon geometry with
. It is a 3-parameter family with a single angular momentum and dipole charge possessing a 2-parameter
extremal limit. The resulting near-horizon geometry with 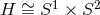 is parameterised by 4-parameters
is parameterised by 4-parameters
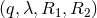 with one constraint relating them. Asymptotic flatness of the full black-hole solution
imposes one further constraint, although from the viewpoint of the near-horizon geometry, this is strictly an
external condition and we will deal with the general case here. The solution is explicitly given by
with one constraint relating them. Asymptotic flatness of the full black-hole solution
imposes one further constraint, although from the viewpoint of the near-horizon geometry, this is strictly an
external condition and we will deal with the general case here. The solution is explicitly given by
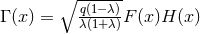 with
with 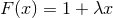 ,
, 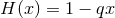 and we have also defined
the length scale
and we have also defined
the length scale 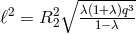 . The parameters satisfy
. The parameters satisfy 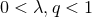 . The local metric
induced on spatial cross sections
. The local metric
induced on spatial cross sections 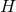 extends smoothly to a metric on
extends smoothly to a metric on 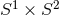 provided
provided
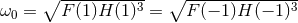 .
.
Charged non-supersymmetric black rings: The dipole ring solution admits a three-parameter charged
generalisation with one independent angular momentum and electric and dipole charges [63] (the removal of
Dirac-Misner string singularities imposes an additional constraint, so this solution has the same number of
parameters as that of [69]). The charged black ring has a two-parameter extremal limit with a
corresponding two-parameter near-horizon geometry. As in the above case, at the level of near-horizon
geometries there is an additional independent parameter corresponding to the arbitrary size of the radius of
the  .
.
Electro-magnetic Kerr black strings: Black string solutions have been constructed carrying five independent
charges: mass  , linear momentum
, linear momentum  along the
along the  of the string, angular momentum
of the string, angular momentum  along
the internal
along
the internal  , as well as electric
, as well as electric  and magnetic charge
and magnetic charge 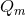 [49]. These solutions
admit a four parameter extremal limit, which in turn give rise to a five-parameter family of
non-static near horizon geometries (the additional parameter is the radius of the
[49]. These solutions
admit a four parameter extremal limit, which in turn give rise to a five-parameter family of
non-static near horizon geometries (the additional parameter is the radius of the 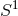 at spatial
infinity) [155
at spatial
infinity) [155 ].
].
For simplicity we will restrict our attention to the solutions with 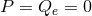 . The resulting
near-horizon solution is parameterised by
. The resulting
near-horizon solution is parameterised by 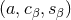 with
with 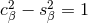 and corresponds to an extremal
string with non-zero magnetic charge and internal angular momentum:
and corresponds to an extremal
string with non-zero magnetic charge and internal angular momentum:
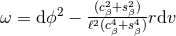 , the function
, the function 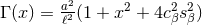 and
the length scale
and
the length scale 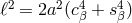 . The induced metric on cross sections of the horizon
. The induced metric on cross sections of the horizon 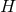 extends
smoothly to a cohomogeneity-1 metric on
extends
smoothly to a cohomogeneity-1 metric on 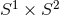 .
.
Although the two solutions (124 ) and (125
) and (125 ) share many features, it is important to emphasise that only
the former is known to correspond to the near-horizon geometry of an asymptotically-flat black ring. It is
conjectured that there exists a general black-ring solution to minimal supergravity that carries mass,
two angular momenta, electric and dipole charges all independently. Hence there should exist
corresponding 4-parameter families of extremal black rings. [155
) share many features, it is important to emphasise that only
the former is known to correspond to the near-horizon geometry of an asymptotically-flat black ring. It is
conjectured that there exists a general black-ring solution to minimal supergravity that carries mass,
two angular momenta, electric and dipole charges all independently. Hence there should exist
corresponding 4-parameter families of extremal black rings. [155 ] discusses the possibility that the
tensionless Kerr-string solution is the near-horizon geometry of these yet-to-be-constructed black
rings.
] discusses the possibility that the
tensionless Kerr-string solution is the near-horizon geometry of these yet-to-be-constructed black
rings.
6.4 Theories with hidden symmetry
Consider 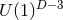 -invariant solutions of a general theory of the form (59
-invariant solutions of a general theory of the form (59 ). One may represent such
solutions by a three-dimensional metric
). One may represent such
solutions by a three-dimensional metric  and a set of scalar fields
and a set of scalar fields 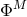 (potentials) all defined on a
three-dimensional manifold, see, e.g., [155
(potentials) all defined on a
three-dimensional manifold, see, e.g., [155 ]. Equivalently, such solutions can be derived from the field
equations of a three-dimensional theory of gravity coupled to a scalar harmonic map whose target manifold
is parameterised by the
]. Equivalently, such solutions can be derived from the field
equations of a three-dimensional theory of gravity coupled to a scalar harmonic map whose target manifold
is parameterised by the 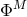 with metric
with metric 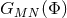 determined by the specific theory. In certain theories
of special interest, the scalar manifold is a symmetric space
determined by the specific theory. In certain theories
of special interest, the scalar manifold is a symmetric space 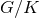 equipped with the bi-invariant metric
equipped with the bi-invariant metric
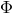 is a coset representative of
is a coset representative of 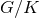 and
and 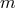 is a normalisation constant dependent on the theory.
Then the theory is equivalent to a three-dimensional theory of gravity coupled to a non-linear sigma model
with target space
is a normalisation constant dependent on the theory.
Then the theory is equivalent to a three-dimensional theory of gravity coupled to a non-linear sigma model
with target space 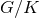 .
.
Consider near-horizon geometries with 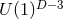 isometry in such theories. It can be shown
[162
isometry in such theories. It can be shown
[162 , 135
, 135 , 155
, 155 ] that the classification problem reduces to an ODE on the orbit space
] that the classification problem reduces to an ODE on the orbit space 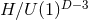 for the
for the
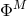 , while
, while 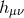 is completely determined. We will assume non-toroidal horizon topology so the orbit
space is an interval, which without loss of generality we take to be
is completely determined. We will assume non-toroidal horizon topology so the orbit
space is an interval, which without loss of generality we take to be ![[− 1,1]](article1483x.gif) parameterised by the
coordinate
parameterised by the
coordinate 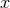 . The ODE is the equation of motion for a non-linear sigma model defined on this interval:
. The ODE is the equation of motion for a non-linear sigma model defined on this interval:
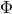 depends only on
depends only on 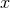 . It is straightforward to integrate this matrix
equation and completely solve for the scalar fields
. It is straightforward to integrate this matrix
equation and completely solve for the scalar fields 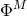 , which can then be used to reconstruct the full
, which can then be used to reconstruct the full
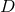 -dimensional solution. Hence in principle one has the full functional form of the solution. However, in
practice, reconstructing the near-horizon data is hindered by the non-linearity of the scalar
metric.
-dimensional solution. Hence in principle one has the full functional form of the solution. However, in
practice, reconstructing the near-horizon data is hindered by the non-linearity of the scalar
metric.
The most notable example which can be treated in the above formalism is vacuum 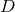 -dimensional
gravity for which
-dimensional
gravity for which 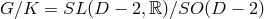 . The classification problem for near-horizon
geometries has been completely solved using this approach [135], as discussed earlier in Section 4.5.1.
. The classification problem for near-horizon
geometries has been completely solved using this approach [135], as discussed earlier in Section 4.5.1.
Four-dimensional Einstein–Maxwell also possesses such a structure where the coset is now
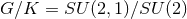 , although the near-horizon classification discussed earlier in Section 6.2 does not
exploit this fact.
, although the near-horizon classification discussed earlier in Section 6.2 does not
exploit this fact.
It turns out that 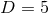 minimal supergravity also has a non-linear sigma model structure with
minimal supergravity also has a non-linear sigma model structure with
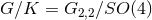 (
(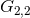 refers to the split real form of the exceptional Lie group
refers to the split real form of the exceptional Lie group 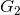 ). The
classification of near-horizon geometries in this case was analysed in [155
). The
classification of near-horizon geometries in this case was analysed in [155 ] using the hidden symmetry and
some partial results were obtained, see Proposition 6.4.
] using the hidden symmetry and
some partial results were obtained, see Proposition 6.4.
It is clear that this method has wider applicability. It would be interesting to use it to classify near-horizon geometries in other theories possessing such hidden symmetry.
We note that near-horizon geometries in this class extremise the energy functional of the harmonic map
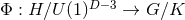 , with boundary conditions chosen such that the corresponding near-horizon data
is smooth. Explicitly, this functional is given by
, with boundary conditions chosen such that the corresponding near-horizon data
is smooth. Explicitly, this functional is given by
 , it
was proved that
, it
was proved that ![E[Φ ] ≥ 0](article1500x.gif) with equality if and only if
with equality if and only if  corresponds to a near-horizon geometry, i.e.,
near-horizon geometries are global minimisers of this functional [1, 132
corresponds to a near-horizon geometry, i.e.,
near-horizon geometries are global minimisers of this functional [1, 132 ]. This result has also been
demonstrated for four dimensional Einstein–Maxwell theory [87
]. This result has also been
demonstrated for four dimensional Einstein–Maxwell theory [87 ] and
] and  Einstein–Maxwell-dilaton
theory [210
Einstein–Maxwell-dilaton
theory [210 , 211
, 211 ]. It would be interesting if this result could be generalised to other theories with hidden
symmetry such as minimal supergravity.
]. It would be interesting if this result could be generalised to other theories with hidden
symmetry such as minimal supergravity.
6.5 Non-Abelian gauge fields
Much less work has been done on classifying extremal black holes and their near-horizon geometries coupled to non-Abelian gauge fields.
The simplest setup for this is four-dimensional Einstein–Yang–Mills theory. As is well known, the standard four dimensional black-hole uniqueness theorems fail in this case (at least in the non-extremal case), for a review, see [205]. Nevertheless, near-horizon geometry uniqueness theorems analogous to the Einstein–Maxwell case have recently been established for this theory.
Static near-horizon geometries in this theory have been completely classified.
Theorem 6.6 ([164 ]). Consider
]). Consider  Einstein–Yang–Mills-
Einstein–Yang–Mills-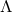 theory with a compact
semi-simple gauge group
theory with a compact
semi-simple gauge group 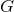 . Any static near-horizon geometry with compact horizon cross section
is given by:
. Any static near-horizon geometry with compact horizon cross section
is given by: 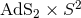 if
if 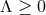 ;
; 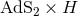 where
where 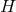 is one of
is one of 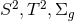 if
if 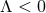 .
.
The proof employs the same method as in Einstein–Maxwell theory. However, it should be noted that
the Yang–Mills field need not be that of the Abelian embedded solution. The horizon gauge field may be
any Yang–Mills connection on 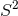 , or on the higher genus surface as appropriate, with a gauge group
, or on the higher genus surface as appropriate, with a gauge group 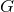 (if there is a non-zero electric field
(if there is a non-zero electric field  the gauge group
the gauge group 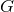 is broken to the centraliser of
is broken to the centraliser of 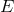 ). The
moduli space of such connections have been previously classified [14]. Hence, unless the gauge group is
). The
moduli space of such connections have been previously classified [14]. Hence, unless the gauge group is
 , one may have genuinely non-Abelian solutions. It should be noted that static near-horizon
geometries have been previously considered in Einstein–Yang–Mills–Higgs under certain restrictive
assumptions [25].
, one may have genuinely non-Abelian solutions. It should be noted that static near-horizon
geometries have been previously considered in Einstein–Yang–Mills–Higgs under certain restrictive
assumptions [25].
Non-static near-horizon geometries have also been classified under the assumption of axisymmetry.
Theorem 6.7 ([164]). Any axisymmetric non-static near-horizon geometry with compact horizon
cross section, in  Einstein–Yang–Mills-
Einstein–Yang–Mills- with a compact semi-simple gauge group, must
be given by the near-horizon geometry of an Abelian embedded extreme Kerr–Newman-
with a compact semi-simple gauge group, must
be given by the near-horizon geometry of an Abelian embedded extreme Kerr–Newman- black hole.
black hole.
The proof of this actually requires new ingredients as compared to the Einstein–Maxwell theory. The
AdS2-symmetry enhancement theorems discussed in Section 3.2 do not apply in the presence of a
non-Abelian gauge field. Nevertheless, assuming the horizon cross sections are of 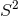 topology allows one
to use a global argument to show the symmetry enhancement phenomenon still occurs. This implies
the solution is effectively Abelian and allows one to avoid finding the general solution to the
ODEs that result from the reduction of the Einstein–Yang–Mills equations. One can also rule
out toroidal horizon cross sections, hence giving a complete classification of horizons with a
topology allows one
to use a global argument to show the symmetry enhancement phenomenon still occurs. This implies
the solution is effectively Abelian and allows one to avoid finding the general solution to the
ODEs that result from the reduction of the Einstein–Yang–Mills equations. One can also rule
out toroidal horizon cross sections, hence giving a complete classification of horizons with a
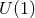 -symmetry.
-symmetry.
Interestingly, Einstein–Yang–Mills theory with a negative cosmological constant is a consistent
truncation of the bosonic sector of 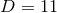 supergravity on a squashed
supergravity on a squashed 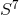 [188]. It would be interesting
if near-horizon classification results could be obtained in more general theories with non-Abelian Yang–Mills
fields, such as the full
[188]. It would be interesting
if near-horizon classification results could be obtained in more general theories with non-Abelian Yang–Mills
fields, such as the full 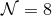 ,
, 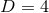 ,
, 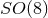 -gauged supergravity that arises as a truncation of
-gauged supergravity that arises as a truncation of
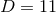 supergravity on
supergravity on 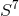 .
.



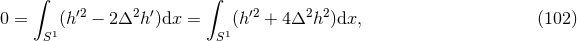


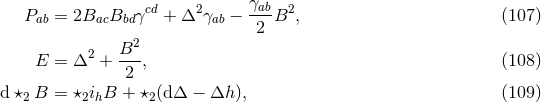
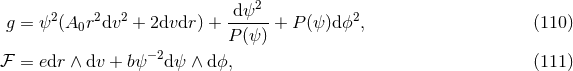

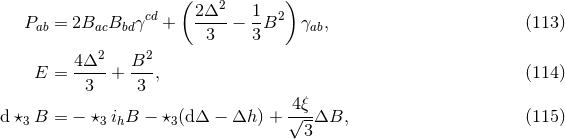
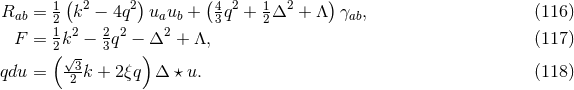
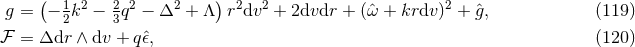
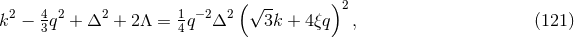
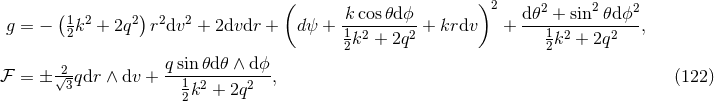
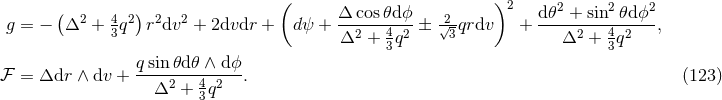
![[ 2 2 ] 2 2 g = Γ (x) − r-dv--+ 2dvdr + ℓΓ-(x)dx-- ℓ2 (1 − x2 ) 2 ( ∘ ------ )2 2 2 2 2 + R1λ(1-+-λ-)H-(x-) dϕ1 + (1-−-λ) 1-−-λrdv + R2q-ω-0(1 −-x-)(dϕ2)2, q(1 − λ)F (x) λR1R2 1 + λ H (x)2 √-- [ ∘ ------ ] -3-- 1-−-q ω0qR2-(1-+-x-) 2 ℱ = 2 d 1 + q H (x ) dϕ , (124 )](article1441x.gif)
![[ 2 2 ] 2 2 4 4 2 2 2 r-dv-- ℓ-Γ (x-)dx- 4a-(cβ-+-sβ)-(1 −-x-) 2 g = Γ (x) − ℓ2 + 2dvdr + (1 − x2) + ℓ2Γ (x ) ω [ 1 23 3 2 2 2 2 ]2 2 Rd-ϕ-- 8a-cβs-β 2a-cβs-β(cβ-+-sβ)(1-−-x-) + a a − ℓ4 rdv + ℓ2Γ (x) ω , √ --3 4 4 [ ] 2--3a-sβcβ-(cβ-+-sβ)- --x-- ℱ = ℓ2 d Γ (x)ω , (125 )](article1462x.gif)
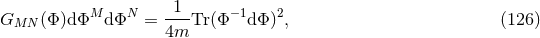
![d [ dΦ ] --- (1 − x2 )Φ −1--- = 0, (127 ) dx dx](article1485x.gif)
![∫ [ ] 1 2 -1-- −1 2 --2---- E [Φ ] = (1 − x )4m Tr(Φ ∂xΦ ) − 1 − x2 dx (128 ) −1](article1498x.gif)