5 Supersymmetric Solutions
By definition, a supersymmetric solution of a supergravity theory is a solution that also admits a Killing spinor, i.e., a spinor field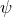 that satisfies
that satisfies 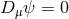 , where
, where 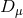 is a spinorial covariant derivative that
depends on the matter fields of the theory. Given such a Killing spinor
is a spinorial covariant derivative that
depends on the matter fields of the theory. Given such a Killing spinor 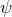 , the bilinear
, the bilinear 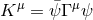 is a
non-spacelike Killing field. By definition, a supersymmetric horizon is invariant under the Killing
field
is a
non-spacelike Killing field. By definition, a supersymmetric horizon is invariant under the Killing
field  and thus
and thus 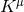 must be tangent to the horizon. Hence
must be tangent to the horizon. Hence 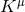 must be null on the
horizon; in other words the horizon is a Killing horizon of
must be null on the
horizon; in other words the horizon is a Killing horizon of 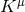 . Furthermore, since
. Furthermore, since 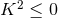 both outside and inside the horizon, it follows that on the horizon
both outside and inside the horizon, it follows that on the horizon 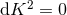 , i.e., it must be
a degenerate horizon. Hence any supersymmetric horizon is necessarily a degenerate Killing
horizon.
, i.e., it must be
a degenerate horizon. Hence any supersymmetric horizon is necessarily a degenerate Killing
horizon.
5.1 Four dimensions
The simplest supergravity theory in four dimensions admitting supersymmetric black holes is minimal
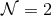 supergravity, whose bosonic sector is simply standard Einstein–Maxwell theory. The general
supersymmetric solution in this theory is given by the Israel–Wilson–Perjés metrics. Using this fact, the
following near-horizon uniqueness theorem has been proved:
supergravity, whose bosonic sector is simply standard Einstein–Maxwell theory. The general
supersymmetric solution in this theory is given by the Israel–Wilson–Perjés metrics. Using this fact, the
following near-horizon uniqueness theorem has been proved:
Theorem 5.1 ([41 ]). Any supersymmetric near-horizon geometry in
]). Any supersymmetric near-horizon geometry in 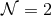 minimal supergravity
is one of the maximally supersymmetric solutions
minimal supergravity
is one of the maximally supersymmetric solutions 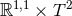 or
or 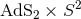 .
.
Notice that staticity here follows from supersymmetry. In Section 7 we will discuss the implications of this result for uniqueness of supersymmetric black holes in four dimensions.
For 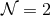 gauged supergravity, whose bosonic sector is Einstein–Maxwell theory with a
negative cosmological constant, an analogous classification of supersymmetric near-horizon
geometries has not been performed. Nevertheless, one may deduce the following result, from a
classification of all near-horizon geometries of this theory under the additional assumption of
axisymmetry:
gauged supergravity, whose bosonic sector is Einstein–Maxwell theory with a
negative cosmological constant, an analogous classification of supersymmetric near-horizon
geometries has not been performed. Nevertheless, one may deduce the following result, from a
classification of all near-horizon geometries of this theory under the additional assumption of
axisymmetry:
Proposition 5.1 ([154 ]). Any supersymmetric, axisymmetric, near-horizon geometry in
]). Any supersymmetric, axisymmetric, near-horizon geometry in 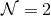 gauged supergravity, is given by the near-horizon limit of the 1-parameter family of supersymmetric
Kerr–Newman-AdS4 black holes [150].
gauged supergravity, is given by the near-horizon limit of the 1-parameter family of supersymmetric
Kerr–Newman-AdS4 black holes [150].
Note that the above near-horizon geometry is non-static. This is related to the fact that supersymmetric AdS black holes must carry angular momentum. It would be interesting to remove the assumption of axisymmetry. Some related work has been done in the context of supersymmetric isolated horizons [29].
Supersymmetric black holes are not expected to exist in 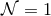 supergravity. For the general
supergravity. For the general 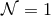 supergravity the following result, supporting this expectation, has been established.
supergravity the following result, supporting this expectation, has been established.
Proposition 5.2 ([115]). A supersymmetric near-horizon geometry of 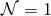 supergravity is
either the trivial solution
supergravity is
either the trivial solution 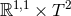 , or
, or 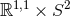 where
where 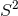 may be a non-round sphere.
may be a non-round sphere.
An example of a supersymmetric near-horizon geometry of the form 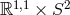 , with a round
, with a round 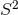 , was
given in [176].
, was
given in [176].
5.2 Five dimensions
The simplest 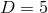 supergravity theory admitting supersymmetric black holes is
supergravity theory admitting supersymmetric black holes is 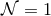 minimal
supergravity. The bosonic sector is Einstein–Maxwell theory with a Chern–Simons term given by Eq. (112
minimal
supergravity. The bosonic sector is Einstein–Maxwell theory with a Chern–Simons term given by Eq. (112 )
with a specific coupling
)
with a specific coupling 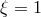 . Supersymmetric solutions to this theory were classified in [93
. Supersymmetric solutions to this theory were classified in [93 ]. This
was used to obtain a complete classification of supersymmetric near-horizon geometries in this
theory.
]. This
was used to obtain a complete classification of supersymmetric near-horizon geometries in this
theory.
Theorem 5.2 ([191 ]). Any supersymmetric near-horizon geometry of minimal supergravity is locally
isometric to one of the following maximally supersymmetric solutions:
]). Any supersymmetric near-horizon geometry of minimal supergravity is locally
isometric to one of the following maximally supersymmetric solutions: 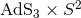 ,
, 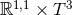 ,
or the near-horizon geometry of the Breckenridge–Myers–Peet–Vafa (BMPV) black hole (of which
,
or the near-horizon geometry of the Breckenridge–Myers–Peet–Vafa (BMPV) black hole (of which
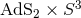 is a special case).
is a special case).
Note that here supersymmetry implies homogeneity. The 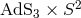 near-horizon geometry has cross
sections
near-horizon geometry has cross
sections 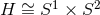 and arises as the near-horizon limit of supersymmetric black rings [64
and arises as the near-horizon limit of supersymmetric black rings [64 , 65
, 65 ] and
supersymmetric black strings [93
] and
supersymmetric black strings [93 , 19
, 19 ]. Analogous results have been obtained in
]. Analogous results have been obtained in 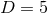 minimal
supergravity coupled to an arbitrary number of vector multiplets [111
minimal
supergravity coupled to an arbitrary number of vector multiplets [111 ]. As discussed in Section 7, the above
theorem can be used to prove a uniqueness theorem for topologically spherical supersymmetric black
holes.
]. As discussed in Section 7, the above
theorem can be used to prove a uniqueness theorem for topologically spherical supersymmetric black
holes.
The corresponding problem for minimal gauged supergravity has proved to be more difficult. The
bosonic sector of this theory is Einstein–Maxwell–Chern–Simons theory with a negative cosmological
constant. The theory admits asymptotically AdS5 black-hole solutions that are relevant in the
context of the AdS/CFT correspondence [120 , 38
, 38 ]. The following partial results have been
shown.
]. The following partial results have been
shown.
Proposition 5.3 ([120 ]). Consider a supersymmetric, homogeneous near-horizon geometry of
minimal gauged supergravity. Cross sections of the horizon must be one of the following: a
homogeneously squashed
]). Consider a supersymmetric, homogeneous near-horizon geometry of
minimal gauged supergravity. Cross sections of the horizon must be one of the following: a
homogeneously squashed 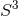 ,
, 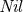 or
or 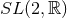 manifold.
manifold.
The near-horizon geometry of the 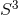 case was used to construct the first example of an asymptotically
AdS5 supersymmetric black hole [120
case was used to construct the first example of an asymptotically
AdS5 supersymmetric black hole [120 ]. Analogous results in gauged supergravity coupled to an arbitrary
number of vector multiplets (this includes
]. Analogous results in gauged supergravity coupled to an arbitrary
number of vector multiplets (this includes 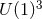 gauged supergravity) were obtained in [151
gauged supergravity) were obtained in [151 ]. Unlike the
ungauged theory, homogeneity is not implied by supersymmetry, and indeed there are more general
solutions.
]. Unlike the
ungauged theory, homogeneity is not implied by supersymmetry, and indeed there are more general
solutions.
Proposition 5.4 ([161 ]). The most general supersymmetric near-horizon geometry in minimal
gauged supergravity, admitting a
]). The most general supersymmetric near-horizon geometry in minimal
gauged supergravity, admitting a 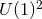 -rotational symmetry and a compact horizon section, is the
near-horizon limit of the topologically-spherical supersymmetric black holes of [38
-rotational symmetry and a compact horizon section, is the
near-horizon limit of the topologically-spherical supersymmetric black holes of [38 ].
].
The motivation for assuming this isometry group is that all known black-hole solutions in five
dimensions possess this. Interestingly, this result implies the non-existence of supersymmetric AdS5 black
rings with 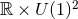 isometry.
isometry.
In fact, recent results allow one to remove all assumptions and obtain a complete classification. Generic
supersymmetric solutions of minimal gauged supergravity preserve 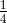 -supersymmetry.
-supersymmetry.
Proposition 5.5 ([121, 106 ]). Any
]). Any 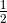 -supersymmetric near-horizon geometry in minimal gauged
supergravity must be invariant under a local
-supersymmetric near-horizon geometry in minimal gauged
supergravity must be invariant under a local 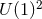 -rotational isometry.
-rotational isometry.
Furthermore, the following has also been shown.
Proposition 5.6 ([105 ]).
Any supersymmetric near-horizon geometry in minimal gauged supergravity with a compact horizon
section must preserve
]).
Any supersymmetric near-horizon geometry in minimal gauged supergravity with a compact horizon
section must preserve 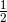 -supersymmetry.
-supersymmetry.
This latter result is proved using a Lichnerowicz type identity to establish a correspondence between Killing spinors and solutions to a horizon Dirac equation, and then applying an index theorem. Therefore, combining the previous three propositions gives a complete classification theorem for near-horizon geometries in minimal gauged supergravity.
Theorem 5.3 ([161, 105, 106]). A supersymmetric near-horizon geometry in minimal gauged
supergravity, with a compact horizon section, must be locally isometric to the near-horizon limit of the
topologically-spherical supersymmetric black holes [38], or the homogeneous near-horizon geometries
with the 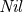 or
or 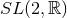 horizons.
horizons.
This theorem establishes a striking corollary for the corresponding black hole classification theorem.
We emphasise that the absence of supersymmetric AdS5 black rings is rather suprising, given
asymptotically-flat counterparts are known to exist [64 ].
].
Parts of the above analysis have been generalised by 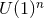 gauged supergravity, although the results
are slightly different.
gauged supergravity, although the results
are slightly different.
Proposition 5.7 ([151 ]). Consider a supersymmetric near-horizon geometry in
]). Consider a supersymmetric near-horizon geometry in 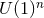 minimal
gauged supergravity with
minimal
gauged supergravity with 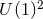 -rotational symmetry and a compact horizon section. It must be
either: (i) the near-horizon limit of the topologically spherical black holes of [160]; or (ii)
-rotational symmetry and a compact horizon section. It must be
either: (i) the near-horizon limit of the topologically spherical black holes of [160]; or (ii) 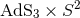 with
with 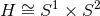 or (iii)
or (iii) 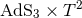 with
with 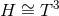 . These latter two cases have constant
scalars and only exist in certain regions of the scalar moduli space (not including the minimal theory).
. These latter two cases have constant
scalars and only exist in certain regions of the scalar moduli space (not including the minimal theory).
Therefore, in this theory one cannot rule out the existence of supersymmetric AdS5 black rings
(although as argued in [151] they would not be connected to the asymptotically-flat black rings [66 ]). It
would be interesting to complete the classification of near-horizon geometries in this more general theory,
along the lines of the minimal theory.
]). It
would be interesting to complete the classification of near-horizon geometries in this more general theory,
along the lines of the minimal theory.
5.3 Six dimensions
The simplest supergravity in six dimensions is minimal supergravity. The bosonic field context of this
theory is a metric and a 2-form potential with self-dual field strength. The classification of supersymmetric
solutions to this theory was given in [112 ]. This was used to work out a complete classification of
supersymmetric near-horizon geometries.
]. This was used to work out a complete classification of
supersymmetric near-horizon geometries.
Theorem 5.4 ([112]). Any supersymmetric near-horizon geometry of 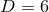 minimal supergravity, with a compact horizon cross section, is either
minimal supergravity, with a compact horizon cross section, is either 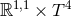 ,
, 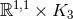 or
locally
or
locally 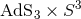 .
.
The 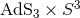 solution has
solution has 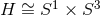 and arises as the near-horizon limit of a supersymmetric
rotating black string.
and arises as the near-horizon limit of a supersymmetric
rotating black string.
Analogous results have been obtained for minimal supergravity coupled to an arbitrary number of scalar and tensor multiplets [3].
5.4 Ten dimensions
Various results have been derived for heterotic supergravity and type IIB supergravity.
The bosonic field content of 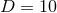 heterotic supergravity consist of the metric, a 2-form gauge
potential and a scalar field (dilaton). The full theory is invariant under 16 supersymmetries. There are two
classes of supersymmetric near-horizon geometries [113
heterotic supergravity consist of the metric, a 2-form gauge
potential and a scalar field (dilaton). The full theory is invariant under 16 supersymmetries. There are two
classes of supersymmetric near-horizon geometries [113 ]. One is the direct product
]. One is the direct product 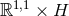 , with
vanishing flux and constant dilaton, where
, with
vanishing flux and constant dilaton, where 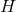 is Spin(7) holonomy manifold, which generically
preserves one supersymmetry (there are solutions in this class which preserve more supersymmetry
provided
is Spin(7) holonomy manifold, which generically
preserves one supersymmetry (there are solutions in this class which preserve more supersymmetry
provided 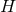 has certain special holonomy). In the second class, the near-horizon geometry is a
fibration of AdS3 over a base
has certain special holonomy). In the second class, the near-horizon geometry is a
fibration of AdS3 over a base 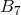 (with a
(with a 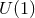 -connection) with a
-connection) with a 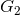 structure, which must
preserve
structure, which must
preserve 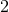 supersymmetries. This class may preserve
supersymmetries. This class may preserve 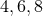 supersymmetries if
supersymmetries if 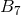 is further
restricted. In particular, an explicit classification for
is further
restricted. In particular, an explicit classification for 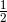 -supersymmetric near-horizon geometries is
possible.
-supersymmetric near-horizon geometries is
possible.
Proposition 5.8 ([113]). Any supersymmetric near-horizon geometry of heterotic supergravity
invariant under 8 supersymmetries, with a compact horizon cross section, must be locally isometric
to one of 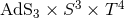 ,
, 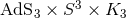 or
or 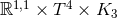 (with constant dilaton).
(with constant dilaton).
A large number of heterotic horizons preserving 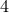 supersymmetries have been constructed, including
explicit examples where
supersymmetries have been constructed, including
explicit examples where 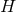 is
is 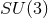 and
and 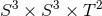 [114].
[114].
The bosonic field content of type IIB supergravity consists of a metric, a complex scalar, a complex
2-form potential and a self-dual 5-form field strength. The theory is invariant under 32 supersymmetries. A
variety of results concerning the classification of supersymmetric near-horizon geometries in this theory have
been derived [102, 101 , 103
, 103 ]. Certain explicit classification results are known for near-horizon
geometries with just a 5-form flux preserving more than two supersymmetries, albeit under certain
restrictive assumptions [101]. More generally, the existence of one supersymmetry places rather
weak geometric constraints on the horizon cross sections
]. Certain explicit classification results are known for near-horizon
geometries with just a 5-form flux preserving more than two supersymmetries, albeit under certain
restrictive assumptions [101]. More generally, the existence of one supersymmetry places rather
weak geometric constraints on the horizon cross sections 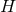 : generically
: generically 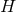 may be any
almost Hermitian spin
may be any
almost Hermitian spin manifold [103]. There are also special cases for which
manifold [103]. There are also special cases for which 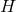 has a
has a
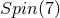 structure and those for which it has an
structure and those for which it has an 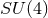 structure (where the Killing spinor is
pure). More recently, it has been shown that any supersymmetric near-horizon geometry in
type IIB supergravity must preserve an even number of supersymmetries, and furthermore, if a
certain horizon Dirac operator has non-trivial kernel the bosonic symmetry group must contain
structure (where the Killing spinor is
pure). More recently, it has been shown that any supersymmetric near-horizon geometry in
type IIB supergravity must preserve an even number of supersymmetries, and furthermore, if a
certain horizon Dirac operator has non-trivial kernel the bosonic symmetry group must contain
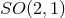 [104].
[104].
5.5 Eleven dimensions
The bosonic field content of 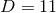 supergravity consists of a metric
supergravity consists of a metric 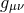 and a 3-form potential
and a 3-form potential
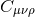 . The near-horizon limit of the 4-form field strength
. The near-horizon limit of the 4-form field strength 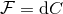 can be written as Eq. (27
can be written as Eq. (27 ), where
), where
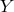 and
and 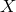 are a 2-form and closed 4-form respectively on the 9-dimensional horizon cross sections
are a 2-form and closed 4-form respectively on the 9-dimensional horizon cross sections 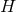 .
The near-horizon Einstein equations are Eqs. (17
.
The near-horizon Einstein equations are Eqs. (17 ) and (18
) and (18 ) with
) with 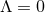 and the matter field terms are
given by [116
and the matter field terms are
given by [116 ]
]
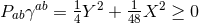 and
and
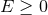 . Therefore, the general results established under these assumptions, discussed in Section 3, are all
valid, including most notably the horizon topology theorem.
. Therefore, the general results established under these assumptions, discussed in Section 3, are all
valid, including most notably the horizon topology theorem.
Various classification results have been derived for supersymmetric near-horizon geometry solutions
under the assumption that cross sections of the horizon are compact. Static supersymmetric near-horizon
geometries are warped products of either 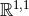 or AdS
2 with
or AdS
2 with 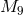 , where
, where 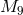 admits a particular
admits a particular
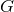 -structure [117]. We note that these warped product forms are guaranteed by the general analysis of
static near-horizon geometries in Section 3.2.1. Supersymmetric near-horizon geometries have been studied
more generally in [116]. Most interestingly, a near-horizon (super)symmetry enhancement theorem has been
established.
-structure [117]. We note that these warped product forms are guaranteed by the general analysis of
static near-horizon geometries in Section 3.2.1. Supersymmetric near-horizon geometries have been studied
more generally in [116]. Most interestingly, a near-horizon (super)symmetry enhancement theorem has been
established.
Theorem 5.5 ([118]). Any supersymmetric near-horizon geometry solution to eleven dimensional
supergravity, with compact horizon cross sections, must preserve an even number of supersymmetries.
Furthermore, the bosonic symmetry group must contain 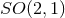 .
.
The proof of this follows by first establishing a Lichnerowicz type identity for certain horizon Dirac operators and then application of an index theorem. As far as bosonic symmetry is concerned, the above result is a direct analogue of the various near-horizon symmetry theorems discussed in Section 3.2, which are instead established under various assumptions of rotational symmetry.