5 Infrared Quantum-Spacetime Phenomenology
Work on Planck-scale quantum-spacetime phenomenology is a rather recent development, with a significant effort taking place over only little more than a decade. But one can already make a distinction between “traditional” and “novel” quantum-spacetime phenomenology approaches. The proposals I have reviewed in the previous two Sections 3 and 4 cover the scope of the “traditional” approach, considering UV effects that could be relevant for observations in astrophysics and/or in controlled-laboratory experiments. I devote this and the next Section 6 to the “novel” idea that Planck-scale quantization of spacetime could have valuable phenomenological implications in some IR regimes and/or that the tests could rely on cosmology.Considering that these “novel” areas of quantum-spacetime phenomenology are in a preliminarily exploratory phase I will adopt a lower standard in the selection of topics, meaning that I will even mention some proposals that have not fully established a link to a definite scheme of spacetime quantization and/or have not fully established the availability of sensitivities that could be compellingly linked with the introduction of spacetime quantization at the Planck scale. I will rather rely on an (inevitably subjective) assessment of whether the relevant proposals provide valuable first steps in the direction of establishing in the not-so-distant future robust Planck-scale quantum-spacetime phenomenology.
5.1 IR quantum-spacetime effects and UV/IR mixing
In the long [508, 475], and so far inconclusive, search for quantum gravity and quantum spacetime the
main strategy was inspired by the discovery paradigm of the 20th century, the “microscope paradigm” with
discovery potential measured in terms of the shortness of the distance scales probed. But recent research has
raised the possibility that by quantizing spacetime at the Planck scale one might have not
only some new phenomena in a far-UV regime, but also some new phenomena in a “dual” IR
regime. Actually, as compellingly stressed in Ref. [176], our present understanding of black-hole
thermodynamics, and particularly the scaling 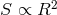 of the entropy of a black hole of radius
of the entropy of a black hole of radius
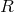 , suggests that such effects of “UV/IR mixing” may be inevitable. It is on the basis of
apparently robust hypotheses concerning the behavior of quantum gravity in the UV (Planckian)
regime that we arrive at this quadratic dependence, which is surprising with respect to what one
might expect in particular in quantum field theory, where cubic scaling (
, suggests that such effects of “UV/IR mixing” may be inevitable. It is on the basis of
apparently robust hypotheses concerning the behavior of quantum gravity in the UV (Planckian)
regime that we arrive at this quadratic dependence, which is surprising with respect to what one
might expect in particular in quantum field theory, where cubic scaling (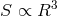 ) naturally
arises. But this feature originating from the UV sector clearly should have its most profound
implications in the large-distance/IR regime since the difference between quadratic dependence on the
radius and cubic dependence on the radius becomes more and more significant as the radius is
increased.35
) naturally
arises. But this feature originating from the UV sector clearly should have its most profound
implications in the large-distance/IR regime since the difference between quadratic dependence on the
radius and cubic dependence on the radius becomes more and more significant as the radius is
increased.35
Another argument in favor of UV/IR mixing is found considering a popular intuition for quantum
spacetime, which relies on the introduction of an uncertainty principle for spacetime itself (in addition to
the Heisenberg one, which acts in phase space). The link with UV/IR mixing can be already seen simply by
considering a principle of the form 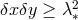 for spatial coordinates, with
for spatial coordinates, with 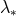 plausibly
on the order of the Planck length. This type of uncertainty relation would evidently imply
that small uncertainty in
plausibly
on the order of the Planck length. This type of uncertainty relation would evidently imply
that small uncertainty in 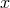 should require large uncertainty in
should require large uncertainty in 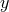 , and this suggests a link
between probing short distance scales (small
, and this suggests a link
between probing short distance scales (small 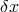 ) and probing large distance scales (large
) and probing large distance scales (large
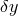 ).
).
For this last point we have more than general arguments: computations in a noncommutative spacetime
compatible with this sort of uncertainty relations, the “canonical spacetime”, with noncommutativity of
coordinates governed by ![[xμ, xν] = i𝜃μν](article854x.gif) , have found explicit manifestations of UV/IR mixing. This is
particularly evident when analyzing mass renormalization within the most popular formalization of
quantum field theories in such canonical noncommutative spacetimes. At one loop one finds
terms in mass renormalization of the form [213
, have found explicit manifestations of UV/IR mixing. This is
particularly evident when analyzing mass renormalization within the most popular formalization of
quantum field theories in such canonical noncommutative spacetimes. At one loop one finds
terms in mass renormalization of the form [213 , 516
, 516 , 397
, 397 ] (for a “
] (for a “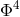 scalar field theory”)
scalar field theory”)
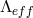 is a peculiar cutoff that can be expressed in terms of a standard UV cutoff
is a peculiar cutoff that can be expressed in terms of a standard UV cutoff 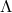 , the
“noncommutativity matrix”
, the
“noncommutativity matrix” 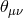 and the momentum
and the momentum 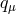 of the particle as follows
Removing the cutoff
of the particle as follows
Removing the cutoff 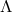 (
(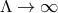 ) one is left with
) one is left with ![Λ2eff = 1∕[qρ(𝜃2)ρσqσ]](article864x.gif) , so that
These power-law and logarithmic IR features are the result of the UV implications of noncommutativity,
which manifest themselves in a rearrangement of the renormalization procedure [213, 516, 397
, so that
These power-law and logarithmic IR features are the result of the UV implications of noncommutativity,
which manifest themselves in a rearrangement of the renormalization procedure [213, 516, 397 ]. In general,
the presence of such sharp features in the IR may be of some concern, since they have not (yet) been
observed. And these concerns are more serious in the cases where these features are sharpest. However, it
should be noticed that different choices of the matrix
]. In general,
the presence of such sharp features in the IR may be of some concern, since they have not (yet) been
observed. And these concerns are more serious in the cases where these features are sharpest. However, it
should be noticed that different choices of the matrix 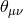 produce very different types of IR behavior, and
it is well established that in the presence (at least in the UV sector) of supersymmetry only the
logarithmic IR features survive (the power-law corrections are removed by one of the standard
supersymmetry-induced cancellation mechanisms). The least virulent IR scenario is obtained by assuming
the presence of UV supersymmetry and choosing a “light-like” noncommutativity matrix [19
produce very different types of IR behavior, and
it is well established that in the presence (at least in the UV sector) of supersymmetry only the
logarithmic IR features survive (the power-law corrections are removed by one of the standard
supersymmetry-induced cancellation mechanisms). The least virulent IR scenario is obtained by assuming
the presence of UV supersymmetry and choosing a “light-like” noncommutativity matrix [19 , 90]
(
, 90]
(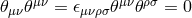 ), so that the main IR feature is a modification of the on-shell relation of the
form
The unit vector
), so that the main IR feature is a modification of the on-shell relation of the
form
The unit vector 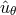 describes a preferential direction [19] determined by the matrix
describes a preferential direction [19] determined by the matrix 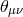 , while the
dimensionless parameter
, while the
dimensionless parameter 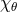 also allows for an expected [397] dependence of the magnitude of the effect
on the specific particle under study: since the IR feature is found in the renormalization procedure, and
this in turn has an obvious dependence on the interactions of a given field with other fields
in the theory, the coefficient of the logarithmic IR correction has different value for different
fields.
also allows for an expected [397] dependence of the magnitude of the effect
on the specific particle under study: since the IR feature is found in the renormalization procedure, and
this in turn has an obvious dependence on the interactions of a given field with other fields
in the theory, the coefficient of the logarithmic IR correction has different value for different
fields.
Note that in the IR regime (small 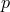 ) one can rewrite (81
) one can rewrite (81 ) as follows
) as follows
Interestingly, canonical noncommutativity is not the only quantum-spacetime proposal that can motivate the
study of UV/IR mixing. This is suggested by the perspective on the semi-classical limit of LQG that
provided motivation for the quantum-spacetime model of Refs. [33 , 34
, 34 ], that also inspired the models
considered in Refs. [154
], that also inspired the models
considered in Refs. [154 , 155
, 155 , 69
, 69 ]. In this LQG-inspired scenario one finds [33
]. In this LQG-inspired scenario one finds [33 , 34
, 34 ] modifications of the
dispersion relation that are linear in momentum in the IR regime, and this has motivated a phenomenology
based36
on the IR dispersion relation [154
] modifications of the
dispersion relation that are linear in momentum in the IR regime, and this has motivated a phenomenology
based36
on the IR dispersion relation [154 , 155
, 155 , 69
, 69 ]
]
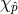 is a phenomenological
parameter37
analogous to
is a phenomenological
parameter37
analogous to 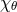 .
.
5.2 A simple model with soft UV/IR mixing and precision Lamb-shift measurements
The long-wavelength behavior of the two scenarios for “soft UV/IR mixing” summarized here in Eq. (82 )
and Eq. (83
)
and Eq. (83 ) evidently differ only because of the fact that invariance under spatial rotations (lost in
Eq. (82
) evidently differ only because of the fact that invariance under spatial rotations (lost in
Eq. (82 )) is preserved by the scenario described in Eq. (83
)) is preserved by the scenario described in Eq. (83 ). Therefore, one could simultaneously consider
the two scenarios, by observing that the characterization of Eq. (82
). Therefore, one could simultaneously consider
the two scenarios, by observing that the characterization of Eq. (82 ) in terms of
) in terms of 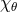 and
and 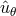 is applicable
to the scenario of Eq. (83
is applicable
to the scenario of Eq. (83 ) by replacing
) by replacing 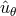 with
with 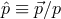 and replacing
and replacing 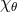 with
with 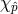 . However, in
light of the limited scope of my review of results on “soft UV/IR mixing”, I shall be satisfied with a
simplified description, assuming space-rotation invariance and limiting my focus to the effects of dispersion
relations of the form
. However, in
light of the limited scope of my review of results on “soft UV/IR mixing”, I shall be satisfied with a
simplified description, assuming space-rotation invariance and limiting my focus to the effects of dispersion
relations of the form
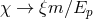 , which provides no loss
of generality if
, which provides no loss
of generality if 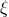 is allowed to be particle dependent).
is allowed to be particle dependent).
The phenomenology of models such as this requires a complete change of strategy with respect to the
phenomenology of quantum-spacetime UV effects that I discussed in previous Sections 3 and 4 of this
review. Whereas the typical search for those UV effects relied on low-precision high-energy data, for the
type of IR effects that I am now considering the best options come from high-precision low-energy data. A
first example of this was given in Ref. [155 ], most notably with a (however brief) discussion of how a
dispersion relation of type (84
], most notably with a (however brief) discussion of how a
dispersion relation of type (84 ) could be relevant for Lamb-shift measurements. Indeed, assuming
Eq. (84
) could be relevant for Lamb-shift measurements. Indeed, assuming
Eq. (84 ) holds for the electron, then one should have a modification of the energy levels of the
hydrogen atom. And in light of the high precision of certain Lamb shift measurements (which
Ref. [155] assesses as being better than one part in
) holds for the electron, then one should have a modification of the energy levels of the
hydrogen atom. And in light of the high precision of certain Lamb shift measurements (which
Ref. [155] assesses as being better than one part in 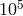 , see also, e.g., Refs. [328, 546]) one
can use this observation to place valuable limits on parameters such as
, see also, e.g., Refs. [328, 546]) one
can use this observation to place valuable limits on parameters such as 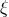 (and
(and 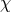 ) for the
electron.
) for the
electron.
5.3 Soft UV/IR mixing and atom-recoil experiments
Evidently the ansatz (84 ) is such that if particles of different mass had the same
value38
of
) is such that if particles of different mass had the same
value38
of 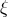 then the effect would be seen more easily for heavier (more massive) types of particles.
then the effect would be seen more easily for heavier (more massive) types of particles.
I find particularly striking the case of measurements of the recoil of cesium (and rubidium) atoms. For
cesium one would assume, following Eq. (84 ), that
), that
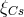 is the
is the  parameter for the case of cesium atoms.
parameter for the case of cesium atoms.
The measurement strategy we proposed in Ref. [69 ] for testing Eq. (85
] for testing Eq. (85 ) with atoms is applicable to
measurements of the “recoil frequency” of atoms with experimental setups involving one or more
“two-photon Raman transitions” [548
) with atoms is applicable to
measurements of the “recoil frequency” of atoms with experimental setups involving one or more
“two-photon Raman transitions” [548 ]. The strategy of the analysis is best described by setting aside
initially the possibility of Planck-scale effects, and looking at the recoil of an atom in a two-photon Raman
transition from the perspective adopted in Ref. [548
]. The strategy of the analysis is best described by setting aside
initially the possibility of Planck-scale effects, and looking at the recoil of an atom in a two-photon Raman
transition from the perspective adopted in Ref. [548 ], which provides a convenient starting point for the
Planck-scale generalization that is of interest here. One can impart momentum to an atom through a
process involving absorption of a photon of frequency
], which provides a convenient starting point for the
Planck-scale generalization that is of interest here. One can impart momentum to an atom through a
process involving absorption of a photon of frequency 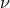 and (stimulated) emission, in the opposite
direction, of a photon of frequency
and (stimulated) emission, in the opposite
direction, of a photon of frequency 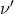 . The frequency
. The frequency 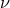 is computed taking into account a resonance
frequency
is computed taking into account a resonance
frequency 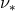 of the atom and the momentum the atom acquires, recoiling upon absorption of
the photon:
of the atom and the momentum the atom acquires, recoiling upon absorption of
the photon: 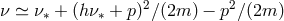 , where
, where 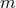 is the mass of the atom (e.g.,
is the mass of the atom (e.g.,
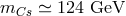 for cesium), and
for cesium), and 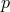 is its initial momentum. The emission of the photon of
frequency
is its initial momentum. The emission of the photon of
frequency 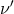 must be such as to de-excite the atom and impart to it additional momentum:
must be such as to de-excite the atom and impart to it additional momentum:
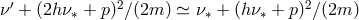 . Through this analysis one establishes that by measuring
. Through this analysis one establishes that by measuring
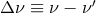 , in cases in which
, in cases in which 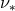 and
and 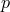 can be accurately determined, one actually measures
can be accurately determined, one actually measures 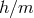 for the atoms:
for the atoms:
![Δ ν ∕[ν∗(ν∗ + p∕h)]](article908x.gif) and of
measurements [277
and of
measurements [277 ] of
] of 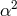 , the square of the fine structure constant.
, the square of the fine structure constant.  can be expressed in terms of the
mass
can be expressed in terms of the
mass 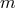 of any given particle [548
of any given particle [548 ] through the Rydberg constant,
] through the Rydberg constant, 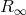 , and the mass of the electron,
, and the mass of the electron,
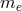 , in the following way [548
, in the following way [548 ]:
]: 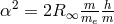 . Therefore, according to Eq. (86
. Therefore, according to Eq. (86 ) one should have
where
) one should have
where 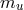 is the atomic mass unit and
is the atomic mass unit and 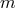 is the mass of the atoms used in measuring
is the mass of the atoms used in measuring ![Δ ν∕[ν (ν + p∕h )] ∗ ∗](article918x.gif) .
The outcomes of atom-recoil measurements, such as the ones with cesium reported in Ref. [548
.
The outcomes of atom-recoil measurements, such as the ones with cesium reported in Ref. [548 ], are
consistent with Eq. (87
], are
consistent with Eq. (87 ) to an accuracy of a few parts in
) to an accuracy of a few parts in  . The fact that Eq. (86
. The fact that Eq. (86 ) has been verified to
such a high degree of accuracy proves to be very valuable, since it turns out [69
) has been verified to
such a high degree of accuracy proves to be very valuable, since it turns out [69 ] that modifications of the
dispersion relation of type (85
] that modifications of the
dispersion relation of type (85 ) require a modification of Eq. (86
) require a modification of Eq. (86 ). Following Ref. [69
). Following Ref. [69 ] one easily finds
and in turn in place of Eq. (87
] one easily finds
and in turn in place of Eq. (87 ) one has
) one has
![[ ( )] Δ ν ( m ) m α2 me mu -------------- 1 − ξCs ----- -------- = -------- --- . 2ν∗(ν∗ + p∕h) 2MP hν ∗ + p 2R ∞ mu m](article921x.gif)
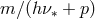 , which in typical experiments of the type here of interest can be [548
, which in typical experiments of the type here of interest can be [548 ] of order
] of order
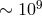 .
.
This turns out to be just enough to provide the desired “Planck-scale sensitivity”: one easily finds that
combining the measurements on cesium reported in Ref. [548] and the determination of 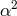 reported in
Ref. [277], one can establish [69] that
reported in
Ref. [277], one can establish [69] that 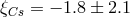 .
.
It is interesting that, besides tests of IR modifications of the dispersion relation, these atom-recoil studies can also be used to investigate possible IR modifications of the law of conservation of momentum. An example of such an analysis is given in Ref. [89].
5.4 Opportunities for Bose–Einstein condensates
The use of atoms in quantum-spacetime phenomenology immediately confronts us with issues that are presently beyond the reach of available theoretical results. A legitimate expectation is that quantum-spacetime effects for atoms could be weaker than for the particles that compose atoms, as a result of the sort of “average-out effects” that one is often expected in the quantum-spacetime literature. This would have to be modeled by introducing an extra suppression factor (a sort of “compositeness factor”) in addition to the Planck-scale suppression that is standard in quantum-spacetime phenomenology. Analyses not making room for such an additional suppression might overestimate the Planck-scale-sensitivity reach of the relevant experiments. On the other hand we are at present not sure whether such compositeness-suppression factors are truly needed, or at least if they are needed in all contexts and in all quantum-spacetime models. For example, it is not unreasonable to imagine that in appropriate quantum-spacetime models, when we achieve the ability to analyze them in detail, we might find that as long as a particle is to be handled as a quantum state (far from its classical limit) then it might be irrelevant for the magnitude of quantum-spacetime effects whether the particle is composite or “fundamental”.
This issue of compositeness will surely gradually take an important role in quantum-spacetime research, but at present it is at a very preliminary stage of investigation, and I shall therefore set it aside. However, do note that if particles composed of a very large number of constituent particles experience Planck-scale effects unsuppressed by their compositeness, then not only atoms but also (and perhaps more powerfully) Bose–Einstein condensates could prove to be a very valuable opportunity for quantum-spacetime phenomenology.
And it is noteworthy that in the recent quantum-spacetime-phenomenology literature there has already
been a surge of interest in the possibilities offered by Bose–Einstein condensates, as seen in
Refs. [542, 472, 139 , 138
, 138 ]. In particular, Refs. [139, 138] study Bose–Einstein condensates adopting a
perspective on soft UV/IR mixing that is closely related to the one discussed for atoms in the previous
Section 5.3.
]. In particular, Refs. [139, 138] study Bose–Einstein condensates adopting a
perspective on soft UV/IR mixing that is closely related to the one discussed for atoms in the previous
Section 5.3.
5.5 Soft UV/IR mixing and the end point of tritium beta decay
Perhaps the most tempting opportunity for the phenomenology of UV/IR mixing comes from studies of the
low-energy beta decay spectrum of tritium,  , which have produced so far some rather
puzzling results [545
, which have produced so far some rather
puzzling results [545 , 370
, 370 ]. It is well understood (see, e.g., Refs. [121, 174]) that these puzzles could be
addressed by introducing deformed rules of kinematics. And it is intriguing that studies conducted near the
endpoint of tritium beta decay are the only known way to accurately investigate the properties
of neutrinos in a non-relativistic (non-ultrarelativistic) regime, where their momenta could
be comparable to their (tiny) masses. So, it would seem to be a very natural opportunity for
advocating UV/IR mixing as a possible explanation. However, the evidence available so far is not
very encouraging for the hope of attributing the magnitude of the reported anomalies to IR
effects induced by the Planck scale. Still, it is noteworthy that specifically the simple model for
soft UV/IR mixing that I described in the previous Sections 5.2 and 5.3 has just the right
structure for producing the sort of anomalies that are being reported, as was first stressed in
Ref. [154
]. It is well understood (see, e.g., Refs. [121, 174]) that these puzzles could be
addressed by introducing deformed rules of kinematics. And it is intriguing that studies conducted near the
endpoint of tritium beta decay are the only known way to accurately investigate the properties
of neutrinos in a non-relativistic (non-ultrarelativistic) regime, where their momenta could
be comparable to their (tiny) masses. So, it would seem to be a very natural opportunity for
advocating UV/IR mixing as a possible explanation. However, the evidence available so far is not
very encouraging for the hope of attributing the magnitude of the reported anomalies to IR
effects induced by the Planck scale. Still, it is noteworthy that specifically the simple model for
soft UV/IR mixing that I described in the previous Sections 5.2 and 5.3 has just the right
structure for producing the sort of anomalies that are being reported, as was first stressed in
Ref. [154 ].
].
The main point of Ref. [154 ] is centered on the properties of the function
] is centered on the properties of the function 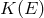 conventionally used
to characterize the Kurie plot of tritium beta decay:
conventionally used
to characterize the Kurie plot of tritium beta decay:
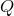 is the difference between initial and final masses of the process,
is the difference between initial and final masses of the process, 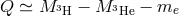 (and,
therefore,
(and,
therefore, 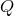 is the sum of the neutrino energy,
is the sum of the neutrino energy, 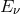 , and the kinetic energy of the electron,
, and the kinetic energy of the electron,
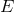 ).
).
Using standard dispersion relations one finds
which does not fit well with the available data near the endpoint [545, 370]. It was observed in Ref. [154 ]
that instead using a modified dispersion relation of type (84
]
that instead using a modified dispersion relation of type (84 ), for negative
), for negative 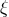 , one obtains better
agreement, but this requires that
, one obtains better
agreement, but this requires that 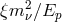 have a value of a few eV. In turn this implies a
value39
of
have a value of a few eV. In turn this implies a
value39
of 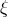 that is extremely large with respect to the natural quantum-spacetime estimate
that is extremely large with respect to the natural quantum-spacetime estimate 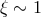 , and as a
result the case for a quantum-spacetime interpretation is rather weak at present. Still, this exciting
experimental situation deserves to be further pursued: perhaps we are modeling soft UV/IR mixing
correctly but we have developed the wrong intuition about the role the Planck scale should play, or perhaps
one should look at alternative ways to model UV/IR mixing.
, and as a
result the case for a quantum-spacetime interpretation is rather weak at present. Still, this exciting
experimental situation deserves to be further pursued: perhaps we are modeling soft UV/IR mixing
correctly but we have developed the wrong intuition about the role the Planck scale should play, or perhaps
one should look at alternative ways to model UV/IR mixing.
5.6 Non-Keplerian rotation curves from quantum-gravity effects
In addition to precision measurements on particles of peculiarly low momentum, another very clear opportunity for UV-IR mixing is provided by data on the behavior of gravity on very large distance scales. And in that context speculating about new-physics phenomena is fully justified by the observed non-Keplerian features of the rotation curves of galaxies or clusters [183]. These non-Keplerian features are usually interpreted as motivation for introducing dark matter (or other non-quantum-gravity new physics, such as MOND [416]), but, in light of the recent awareness of the possibility of UV/IR mixing, it is legitimate to speculate that they may be at least in part due to quantum-spacetime effects.
The perspective one might adopt in trying to profit from this opportunity is similar to when one works within standard quantum field theories and derives an “effective potential” (usually obtained through the calculation of loop contributions) that corrects the tree-level classical potential.
Interestingly, the type of modifications of dispersion relations that have been motivated by
quantum-spacetime research do automatically suggest that the Newtonian potential should receive some
corresponding corrections. In fact, the Newtonian potential is produced by a static point source when the
field that mediates the force described by the potential has energy-momentum space (inverse)
propagator 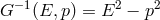 . In general, if the field that mediates the force has a different
propagator,
. In general, if the field that mediates the force has a different
propagator, 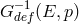 , the Newtonian potential produced at the spatial point
, the Newtonian potential produced at the spatial point 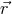 by a point-like
mass
by a point-like
mass 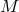 , located at the origin, is replaced by the potential obtained by computing [283]
, located at the origin, is replaced by the potential obtained by computing [283]
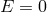 .
.
A more articulated argument for modifications of the Newton potential at large distances from a
quantum-spacetime perspective has been put forward as part of the mentioned research program on
“asymptotic safety”. This is done in Ref. [469 ], which indeed adopts as a working assumption the
availability of a quantum field theory of gravity whose underlying degrees of freedom are those of the
spacetime metric, defined nonperturbatively as a fundamental, “asymptotically-safe” theory. Obtaining
definite predictions for the rotation curves of galaxies or clusters within this formalism is presently well
beyond our technical capabilities. However, preliminary studies of the renormalization-group behavior
provide encouragement for a certain level of analogy between this theory and non-Abelian Yang–Mills
theories, and, relying in part on this analogy, Ref. [469] argued that one could obtain non-Keplerian
features from renormalization.
], which indeed adopts as a working assumption the
availability of a quantum field theory of gravity whose underlying degrees of freedom are those of the
spacetime metric, defined nonperturbatively as a fundamental, “asymptotically-safe” theory. Obtaining
definite predictions for the rotation curves of galaxies or clusters within this formalism is presently well
beyond our technical capabilities. However, preliminary studies of the renormalization-group behavior
provide encouragement for a certain level of analogy between this theory and non-Abelian Yang–Mills
theories, and, relying in part on this analogy, Ref. [469] argued that one could obtain non-Keplerian
features from renormalization.
5.7 An aside on gravitational quantum wells
Another opportunity for studies of UV/IR mixing is provided by measurements performed on
neutron quantum states in the gravity field of the Earth, such as the striking ones reported in
Refs. [428 , 429
, 429 ]. I have nothing to report on this that would fit the main focus of this review,
concerning Planck-scale quantum pictures of spacetime, but it seemed worth mentioning this
nonetheless, especially in light of the fact that this class of low-energy studies (candidates for
the investigation of UV/IR mixing) have already been analyzed from the perspective of some
quantum spacetimes, even though so far all such studies have introduced spacetime quantization at
scales that are very far from the Planck scale (much lower energy scales, much greater distance
scales).
]. I have nothing to report on this that would fit the main focus of this review,
concerning Planck-scale quantum pictures of spacetime, but it seemed worth mentioning this
nonetheless, especially in light of the fact that this class of low-energy studies (candidates for
the investigation of UV/IR mixing) have already been analyzed from the perspective of some
quantum spacetimes, even though so far all such studies have introduced spacetime quantization at
scales that are very far from the Planck scale (much lower energy scales, much greater distance
scales).
Since I am already here diverting from the main theme of the review, I shall be satisfied confining the
discussion of quantum-spacetime studies of the gravitational quantum well to the particularly interesting
points made in Refs. [118 , 102
, 102 , 483
, 483 , 137
, 137 ]. The studies in Refs. [118
]. The studies in Refs. [118 , 102
, 102 , 483
, 483 ] all assumed “canonical
noncommutativity” of spacetime coordinates:
] all assumed “canonical
noncommutativity” of spacetime coordinates:
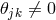 ) from the space/time noncommutativity
(
) from the space/time noncommutativity
(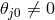 ).
).
And Refs. [118 , 102
, 102 , 483
, 483 ] agree on the fact that pure space/space noncommutativity (
] agree on the fact that pure space/space noncommutativity (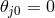 ) has no
significant implications for the gravitational quantum well. However, Ref. [483] notices that with
space/time noncommutativity (
) has no
significant implications for the gravitational quantum well. However, Ref. [483] notices that with
space/time noncommutativity (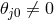 ) there are tangible consequences for the gravitational quantum
well so that in turn one can use the measurement results of Refs. [428
) there are tangible consequences for the gravitational quantum
well so that in turn one can use the measurement results of Refs. [428 , 429
, 429 ] to put bounds on space/time
noncommutativity,40
although only at the level
] to put bounds on space/time
noncommutativity,40
although only at the level 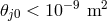 (whereas interest from the Planck-scale-quantum-spacetime side
would focus in the neighborhood of
(whereas interest from the Planck-scale-quantum-spacetime side
would focus in the neighborhood of 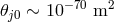 ).
).
Refs. [118, 102] make the choice of combining space/space noncommutativity with a noncommutativity of momentum space:
It then turns out that this noncommutativity of momentum space does tangibly affect the analysis of the gravitational quantum well. So that in turn one can use the measurement results of Refs. [428 , 429
, 429 ] to
place bounds at the level
] to
place bounds at the level 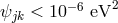 .
.
Ref. [137 ] is an example of analysis of the gravitational quantum well not from the viewpoint of
spacetime noncommutativity, but rather from the viewpoint of the scheme of spacetime quantization
introduced in Refs. [323, 322], which is centered on a modification of the Heisenberg principle
] is an example of analysis of the gravitational quantum well not from the viewpoint of
spacetime noncommutativity, but rather from the viewpoint of the scheme of spacetime quantization
introduced in Refs. [323, 322], which is centered on a modification of the Heisenberg principle
 does turn out [137] to affect the analysis of the gravitational quantum
well, and using the measurement results of Refs. [428, 429] one can place bounds at the level
does turn out [137] to affect the analysis of the gravitational quantum
well, and using the measurement results of Refs. [428, 429] one can place bounds at the level
 .
.

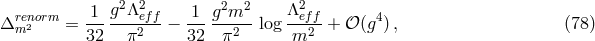

![2 Δren2orm ∼ ----g----- + g2m2 log [m2q (𝜃2)ρσq ]. (80 ) m qρ(𝜃2)ρσqσ ρ σ](article865x.gif)
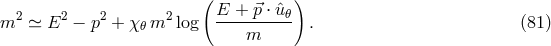






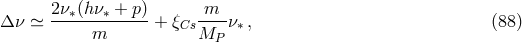
![[∫ ]1∕2 K (E ) = dp ν p2ν δ (Q − E − Eν) , (89 )](article928x.gif)
![[ ∘ --------2----2-]1∕2 K (E ) = (Q − E ) (Q − E ) − m ν) , (90 )](article934x.gif)
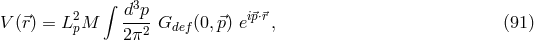
![[xj,xk ] = i𝜃jk , [xj,x0] = i𝜃j0, (92 )](article949x.gif)
![[pj,pk] = iψjk. (93 )](article956x.gif)
![[x ,p ] = iδ (1 + βp2 ). (94 ) j k jk](article958x.gif)