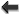12 Selected Astrophysical Applications
12.1 Measurements of black-hole mass and spin
Astrophysical black holes are not charged, and thus are characterized only by their mass and spin.
Measurements of black hole mass are generally straightforward, requiring only the observation of an
orbital companion and an application of Kepler’s laws. Current mass estimates for stellar-mass
black holes (in particular microquasars) are reviewed by McClintock & Remillard [184 ] and for
supermassive black holes by Kormendy & Richstone [159] (see also [196] for somewhat more recent
data).
] and for
supermassive black holes by Kormendy & Richstone [159] (see also [196] for somewhat more recent
data).
Nevertheless, there remain several fundamental questions connected with the mass of black holes, and
some of them are directly connected to accretion disk theory. One of them is the question of ultra-luminous
X-ray sources (ULXs) [61, 181]. ULXs are powerful X-ray sources located outside of galactic nuclei, which
have luminosities in excess of the Eddington limit for 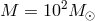 , assuming isotropic emission. The huge
luminosities of ULXs lead some to conclude that they are accreting intermediate-mass black holes, with
masses
, assuming isotropic emission. The huge
luminosities of ULXs lead some to conclude that they are accreting intermediate-mass black holes, with
masses 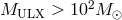 (e.g., [165, 198]). Others think that they are stellar mass black holes with
(e.g., [165, 198]). Others think that they are stellar mass black holes with
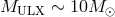 , either exhibiting beamed emission [108] or surrounded by disks that are somehow able
to produce highly super-Eddington luminosities (e.g., [147, 36]). At this time, at least one
ULX (ESO 243-49/HLX-1) has been convincingly demonstrated to have an intermediate mass
(
, either exhibiting beamed emission [108] or surrounded by disks that are somehow able
to produce highly super-Eddington luminosities (e.g., [147, 36]). At this time, at least one
ULX (ESO 243-49/HLX-1) has been convincingly demonstrated to have an intermediate mass
( ) [90, 67].
) [90, 67].
As difficult as it is to nail down the mass on some of these systems, it is even more difficult to measure
black hole spin, even though it plays a direct, and important, role in accretion disk physics. One obvious
example of the role of spin is the dependence of 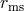 , the coordinate radius of the ISCO, on spin. In the
symmetry plane of the black hole
, the coordinate radius of the ISCO, on spin. In the
symmetry plane of the black hole 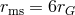 for
for 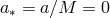 (non-rotating),
(non-rotating), 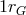 for
for
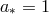 (maximal prograde rotation), and
(maximal prograde rotation), and 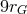 for
for 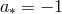 (maximal retrograde rotation)
(Section 2.5). It is believed that the inner edge of the accretion disk will be similarly affected.
In addition, for rapidly rotating black holes, the Blandford–Znajek mechanism, and similar
processes which depend on spin, may account for a fair share of the global energetics, comparable
to that of accretion itself (see, e.g., [155, 189] and references therein). Therefore, measuring
the spin of accreting black holes is integrally tied up in understanding black hole accretion
generally.
(maximal retrograde rotation)
(Section 2.5). It is believed that the inner edge of the accretion disk will be similarly affected.
In addition, for rapidly rotating black holes, the Blandford–Znajek mechanism, and similar
processes which depend on spin, may account for a fair share of the global energetics, comparable
to that of accretion itself (see, e.g., [155, 189] and references therein). Therefore, measuring
the spin of accreting black holes is integrally tied up in understanding black hole accretion
generally.
Four methods for determining black hole spin have been proposed in the literature. With references to
some of the earliest results, they are: 1) fitting the continuum spectra of microquasars observed in the
thermally dominant state using disk emission models [65, 185, 197, 277]; 2) fitting observed relativistically
broadened iron line profiles with theoretical models [142, 315, 201, 202, 260]; 3) matching observed QPO
frequencies to those predicted by theoretical models [62, 11 , 259, 306]; and 4) analyzing the “shadow” a
black hole makes on the surface of an accretion disk [301]. The first three methods have been the most
commonly applied to date. Although there have been some glaring discrepancies in the spin estimates
published to date (e.g., one group claiming that Cyg X-1 has a near-zero spin,
, 259, 306]; and 4) analyzing the “shadow” a
black hole makes on the surface of an accretion disk [301]. The first three methods have been the most
commonly applied to date. Although there have been some glaring discrepancies in the spin estimates
published to date (e.g., one group claiming that Cyg X-1 has a near-zero spin, 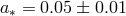 [200], and
then later claiming it has a near-maximal spin,
[200], and
then later claiming it has a near-maximal spin, 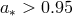 [89]), there appears to be a settling of values in
recent years and a growing confidence in the methods, particularly the continuum fitting. There are
still some concerns, however. For the continuum fitting method, the main issue is that the
inclination of the X-ray emitting region must be measured by some independent means. This is
because the effect of the inclination on the spectrum is degenerate with the effect of spin [175], so
both can not be accounted for within the continuum fitting method. In cases where such an
independent measure is available (e.g. [292]), the continuum fitting method appears robust. For the
relativistically-broadened iron line method, there are difficulties in properly estimating the extent of the
“red” wing, which is most directly related to the spin of the black hole, and in modeling the
hard X-ray source photons and the disk ionization, both of which strongly affect the reflection
spectrum. The reviews by Remillard, McClintock, and collaborators [258, 186] give more complete
introductions to the topic of measuring black hole spin, with emphasis mainly on the continuum fitting
method.
[89]), there appears to be a settling of values in
recent years and a growing confidence in the methods, particularly the continuum fitting. There are
still some concerns, however. For the continuum fitting method, the main issue is that the
inclination of the X-ray emitting region must be measured by some independent means. This is
because the effect of the inclination on the spectrum is degenerate with the effect of spin [175], so
both can not be accounted for within the continuum fitting method. In cases where such an
independent measure is available (e.g. [292]), the continuum fitting method appears robust. For the
relativistically-broadened iron line method, there are difficulties in properly estimating the extent of the
“red” wing, which is most directly related to the spin of the black hole, and in modeling the
hard X-ray source photons and the disk ionization, both of which strongly affect the reflection
spectrum. The reviews by Remillard, McClintock, and collaborators [258, 186] give more complete
introductions to the topic of measuring black hole spin, with emphasis mainly on the continuum fitting
method.
12.2 Black hole vs. neutron star accretion disks
Little of what we have said so far has depended on whether the central compact object is a black hole or
neutron star, provided only that the neutron star is compact enough to lie inside the inner radius of the disk
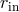 . In this case, its presence will not be noticed by the disk except through its gravity, which will be
practically the same as for a black hole (an exception would be if the neutron star is strongly
magnetized [113]). However, this does not mean that accreting black hole and neutron star
sources will be indistinguishable, as we have not yet fully addressed the question of what happens
to energy advected past
. In this case, its presence will not be noticed by the disk except through its gravity, which will be
practically the same as for a black hole (an exception would be if the neutron star is strongly
magnetized [113]). However, this does not mean that accreting black hole and neutron star
sources will be indistinguishable, as we have not yet fully addressed the question of what happens
to energy advected past 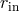 . For optically thick, geometrically thin Shakura–Sunyaev disks
(Section 5.3), a significant fraction of the gravitational energy liberated by advection is radiated by the
gas prior to it passing through
. For optically thick, geometrically thin Shakura–Sunyaev disks
(Section 5.3), a significant fraction of the gravitational energy liberated by advection is radiated by the
gas prior to it passing through 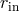 . Thus, the total luminosity of thin disks will not depend
sensitively on the nature of the central object. However, this is not the case for the ADAF solution
(Section 7), for which much of the thermal energy gained by the gas from accretion is carried
all the way in to the central object. Narayan and his collaborators [225
. Thus, the total luminosity of thin disks will not depend
sensitively on the nature of the central object. However, this is not the case for the ADAF solution
(Section 7), for which much of the thermal energy gained by the gas from accretion is carried
all the way in to the central object. Narayan and his collaborators [225 , 215
, 215 , 195
, 195 , 107
, 107 ] have
convincingly argued that this may allow observers to distinguish between black hole and neutron star
sources.
] have
convincingly argued that this may allow observers to distinguish between black hole and neutron star
sources.
The key is that, for black hole sources, advection through the event horizon allows the excess
thermal energy to be effectively absorbed without ever radiating. For neutron star sources, on
the other hand, the presence of a hard surface ensures that the excess energy of accretion is
released upon impact and must be radiated to infinity. This implies that for systems in the
ADAF state, a black hole source should be significantly less luminous that a neutron star one
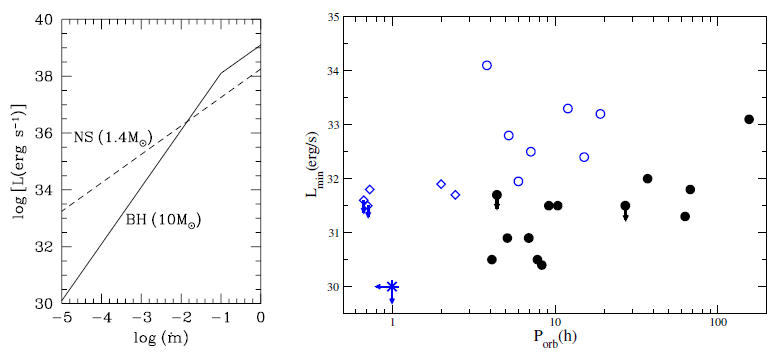
with the same mass accretion rate [225]. Perhaps a more important point is that the range of
luminosities should be wider for a black hole source than for a neutron star one [215 ]. This is
because, while the luminosity goes as
]. This is
because, while the luminosity goes as 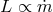 for all neutron star states and for black holes
in a high accretion state, it goes as
for all neutron star states and for black holes
in a high accretion state, it goes as 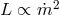 for black holes in the ADAF state for which
for black holes in the ADAF state for which
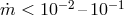 . Furthermore, since the luminosity is also proportional to the mass of the central object
. Furthermore, since the luminosity is also proportional to the mass of the central object
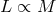 , at the highest accretion rates a black hole source should be more luminous than a
neutron star one due to its higher mass (Figure 23
, at the highest accretion rates a black hole source should be more luminous than a
neutron star one due to its higher mass (Figure 23 ). Another key point to this argument is that
neutron stars can independently and reliably be confirmed if they display type I bursts, which are
thermonuclear flashes occurring in material accumulated on the surface of the neutron star [141].
Thus one can compare known neutron star sources against suspected black hole sources. This
has now been done in a number of otherwise similar sources and Narayan’s expectations have
indeed been confirmed [215
). Another key point to this argument is that
neutron stars can independently and reliably be confirmed if they display type I bursts, which are
thermonuclear flashes occurring in material accumulated on the surface of the neutron star [141].
Thus one can compare known neutron star sources against suspected black hole sources. This
has now been done in a number of otherwise similar sources and Narayan’s expectations have
indeed been confirmed [215 , 195, 107] (a recent example is shown in Figure 23
, 195, 107] (a recent example is shown in Figure 23 ). This provides
compelling observational evidence for the existence of black hole event horizons, although this falls
short of being a proof [12]. This topic is discussed further in the review article by Narayan and
McClintock [219].
). This provides
compelling observational evidence for the existence of black hole event horizons, although this falls
short of being a proof [12]. This topic is discussed further in the review article by Narayan and
McClintock [219].
12.3 Black-hole accretion disk spectral states
Black hole accretion disks, particularly in X-ray binaries, exhibit complex spectra composed of both thermal and nonthermal components. During outbursts, the relative strengths of these components change frequently in concert with changes in luminosity and the characteristics of the radio features (i.e., jets). Astronomers have developed a set of empirical spectral classification states to broadly characterize these observations. These spectral states likely reveal important information about the underlying physical state of the system; therefore it is worth summarizing the states and their observed properties here.
Probably the easiest state to connect with a theoretical model is the “High/Soft” (HS) or “Thermally
Dominant” state. As the name implies, the spectrum in this state is dominated by the thermal component.
This state is best explained as 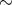 1 keV thermal emission from a multitemperature accretion
disk, as predicted by the Shakura–Sunyaev (thin) disk model (Section 5.3). However, most
sources spend the majority of their lifetimes in the “Low/Hard” (LH) or even “quiescent” state.
The quiescent state is characterized by exceptionally low luminosity and a hard, non-thermal
spectrum (photon index
1 keV thermal emission from a multitemperature accretion
disk, as predicted by the Shakura–Sunyaev (thin) disk model (Section 5.3). However, most
sources spend the majority of their lifetimes in the “Low/Hard” (LH) or even “quiescent” state.
The quiescent state is characterized by exceptionally low luminosity and a hard, non-thermal
spectrum (photon index 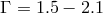 ). As the luminosity increases the sources usually enters the
Hard state. Here the 2 – 10 keV intensity is still comparatively low and the spectrum is still
nonthermal. This spectrum is best fit with a powerlaw of photon index
). As the luminosity increases the sources usually enters the
Hard state. Here the 2 – 10 keV intensity is still comparatively low and the spectrum is still
nonthermal. This spectrum is best fit with a powerlaw of photon index 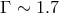 (220 keV). In
this state, the thermal component is either not detected or appears much cooler, indicating
the thin disk may truncate further out than in the Thermally Dominant state, although see
[199] for claims that the thin disk extends all the way to the ISCO even in the Hard state.
Observations suggest the region interior to the thin disk may be filled with a hot (presumably thick),
optically-thin plasma, which accounts for the nonthermal part of the spectrum. This is the picture
suggested by the “truncated disk model” [85, 86, 84], which pictures the Hard state as a truncated
thin (Shakura–Sunyaev) disk adjoined with an inner thick (ADAF-like) flow. This model has
shown tremendous phenomenological success [79], although other models for the Hard state still
abound [323, 101, 136]. The Hard state is also linked with observations of a persistent radio jet that is not
seen in other states (see Section 10). The final spectral state, which is referred to as the “Very
High” (VH) or “Steep Power Law” state, is characterized by the appearance of high-frequency
QPOs (Section 12.4) and the presence of both disk and powerlaw components, each of which
contributes substantial luminosity. In this state, the powerlaw component is observed to be steep
(
(220 keV). In
this state, the thermal component is either not detected or appears much cooler, indicating
the thin disk may truncate further out than in the Thermally Dominant state, although see
[199] for claims that the thin disk extends all the way to the ISCO even in the Hard state.
Observations suggest the region interior to the thin disk may be filled with a hot (presumably thick),
optically-thin plasma, which accounts for the nonthermal part of the spectrum. This is the picture
suggested by the “truncated disk model” [85, 86, 84], which pictures the Hard state as a truncated
thin (Shakura–Sunyaev) disk adjoined with an inner thick (ADAF-like) flow. This model has
shown tremendous phenomenological success [79], although other models for the Hard state still
abound [323, 101, 136]. The Hard state is also linked with observations of a persistent radio jet that is not
seen in other states (see Section 10). The final spectral state, which is referred to as the “Very
High” (VH) or “Steep Power Law” state, is characterized by the appearance of high-frequency
QPOs (Section 12.4) and the presence of both disk and powerlaw components, each of which
contributes substantial luminosity. In this state, the powerlaw component is observed to be steep
(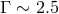 ), giving the state its name. This state is sometimes associated with intermittent
jets.
), giving the state its name. This state is sometimes associated with intermittent
jets.
These states, their distinguishing observational properties, and a sampling of observations from various black hole X-ray binaries is presented in the review by McClintock and Remillard [184].
12.4 Quasi-Periodic Oscillations (QPOs)
Einstein’s general theory of gravity has never been tested in its strong field limit, characteristic of the region very near black holes (or neutron stars), i.e., a few gravitational radii away from these sources. Soon VLBI measurements may be able to resolve these scales for the supermassive black hole at the center of our galaxy. However, for most sources, resolution in time seems to be a more practical approach. To zeroth order, the light curves from accreting black holes vary in a chaotic manner, resembling a loud noise. However, Fourier analysis of the light curve reveals stinkingly regular patterns buried in the noise. For Galactic black hole and neutron star sources, these quasi-periodic oscillations (QPOs) have frequencies of a few hundred Hz.
Frequencies in the range 100 – 1000 Hz formally correspond to orbital frequencies a few gravitational
radii away from a stellar-mass object. The focus on orbital frequencies is further motivated by the stability
of observed QPO frequencies over very long periods of time. For example, QPOs of 300 Hz and 450 Hz
were observed from the microquasar GRO J1655–40 during its 1996 outburst and again almost nine years
later during its 2005 outburst. This strongly suggests that the frequencies cannot depend on quantities such
as magnetic field, density, temperature, or accretion rate, as these all vary greatly in time. The only
parameters of a black hole accretion system that do not vary over a nine year period are the mass and the
spin of the central black hole. Thus, the oscillation frequencies must only depend on these two parameters,
and only frequencies connected to orbital motion have the property that they depend only on
mass and spin. Thus, the possible frequencies are: the Keplerian frequency, the two epicyclic
frequencies (as originally suggested by Kluźniak and Abramowicz [11 ]), the Lense–Thirring
frequency (as originally suggested by Stella and Vietri [294]), and their combinations (e.g.,
[145, 143]).
]), the Lense–Thirring
frequency (as originally suggested by Stella and Vietri [294]), and their combinations (e.g.,
[145, 143]).
In several microquasars the detected high-frequency QPOs come not as single oscillations, but as part of a pair. Furthermore, Abramowicz and Kluźniak [11] noticed that they are commensurable, being most often in a 2/3 ratio, as shown in Table 2. This suggests that a resonance may be at work. Twin peak QPOs in the kilohertz range have been also detected from binaries containing accreting neutron stars. These neutron star QPOs show a similar, though less obvious, 2/3 ratio.
| Microquasar | Frequency ratio |
| GRO J1655–40 | 300/450 = 0.66 |
| XTE 1550–564 | 184/276 = 0.66 |
| H 1743–322 | 166/240 = 0.69 |
| GRS 1915+105 | 113/168 = 0.67 |
It was realized [150] that the behavior of the observed QPO frequencies and amplitudes in both neutron star binaries and microquasars is typical for a certain type of non-linear resonance. Indeed, it may be observed when a properly tuned spring is attached to a pendulum with a properly chosen length. Such a system oscillates in two “modes”: the pendulum mode and the spring mode.15 Mostly due to efforts of Rebusco and Horák [256, 130, 131, 132], a mathematical resonance model was developed to describe an arbitrary system oscillating in two modes near a 2/3 non-linear resonance. The model’s predictions for the frequency and amplitude behaviors are strikingly similar to the ones observed in X-ray binary QPOs, suggesting that they may indeed be explained as a non-linear resonance of two modes of oscillation. The model does not, however, explain what these modes are, how they are excited, nor what energy reservoir they tap. Only when these questions are answered satisfactorily could one say that the QPO puzzle is solved.16
As a final note, a crucial discovery by Barret and collaborators [32, 33, 34] concerning the behavior of the quality factor of twin peak QPOs proves that they are disk oscillations and cannot be explained by kinematic (Doppler) effects due to the presence “hot spots” on the accretion disk surface. These effects are, however, important in modulating the QPO’s signal [55]. It is not clear, though, whether the oscillations are explained by the discoseismic modes discussed in Section 9.2 (see, e.g., [313] for references).
12.5 The case of Sgr A*
As already mentioned in Section 2.1.1, there is a very good chance that the first direct evidence for a black hole event horizon will come from Sgr A*, the compact, supermassive object at the center of the Milky Way. There have already been a number of strong, indirect arguments in favor of the black hole nature of Sgr A* [54, 53], but no direct evidence yet. Sgr A* is also of interest because it represents a unique case of black hole accretion, having by far the lowest (scaled) mass accretion rate and radiative efficiency of any known source. We anticipate greatly expanding this section in the near future as new results become available.