1 Introduction
Close binaries consisting of two compact stellar remnants – white dwarfs (WDs), neutron stars (NSs) or black holes (BHs) are considered primary targets of the forthcoming field of gravitational wave (GW) astronomy (see, for a review, [758*, 252*, 673*, 672]), since their orbital evolution is entirely controlled by the emission of gravitational waves and leads to ultimate coalescence (merger) and possible explosive disruption of the components. Emission of gravitational waves accompanies the latest stages of evolution of stars and manifests instabilities in relativistic objects [10, 13]. Close compact binaries can thus serve as testbeds for theories of gravity [221]. The NS(BH) binary mergers that release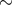 1052 erg as GWs [113, 114] should be the brightest GW events in the
10 – 1000 Hz frequency band of the existing or future ground-based GW detectors like LIGO [23],
VIRGO [6], GEO600 [635], KAGRA(LCGT) [728] (see also [642] for a review of the current
state of existing and 2nd- and 3rd-generation ground-based detectors). Mergers of NS(BH)
binaries can be accompanied by the release of a huge amount of electromagnetic energy in a burst
and manifest themselves as short gamma-ray bursts (GRBs). A lot of observational support
for NS-NS/NS-BH mergers as sources of short GRBs have been obtained (see, e.g., studies of
short GRB locations in the host galaxies [204*, 751*] and references therein). As well, relativistic
jets, associated with GRB of any nature may be sources of GW in the ground-based detectors
range [50].
1052 erg as GWs [113, 114] should be the brightest GW events in the
10 – 1000 Hz frequency band of the existing or future ground-based GW detectors like LIGO [23],
VIRGO [6], GEO600 [635], KAGRA(LCGT) [728] (see also [642] for a review of the current
state of existing and 2nd- and 3rd-generation ground-based detectors). Mergers of NS(BH)
binaries can be accompanied by the release of a huge amount of electromagnetic energy in a burst
and manifest themselves as short gamma-ray bursts (GRBs). A lot of observational support
for NS-NS/NS-BH mergers as sources of short GRBs have been obtained (see, e.g., studies of
short GRB locations in the host galaxies [204*, 751*] and references therein). As well, relativistic
jets, associated with GRB of any nature may be sources of GW in the ground-based detectors
range [50].
WD binaries, especially those observed as AM CVn-stars and ultracompact X-ray binaries (UCXB), are potential GW sources within the frequency band (10–4 – 1) Hz of the space GW interferometers like (the currently cancelled) LISA [187*],1 NGO (eLISA) [10, 11], DEGIGO [857] and other proposed or planned low-frequency GW detectors [122, 40]. At the moment, eLISA is selected for the third large-class mission in ESA’s Cosmic Vision science program (L3). Its first step should be the launch of ESA’s LISA Pathfinder (LPF) mission in 2015; the launch of eLISA itself is currently planned for 2034.2
WD binary mergers are among the primary candidate mechanisms for type Ia supernovae (SNe Ia)
explosions. The NIR magnitudes of the latter are considered as the best “standard candles” [26] and, in
this guise, are crucial in modern cosmological studies [638, 578]. Further improvements in the
precision of standardization of SNe Ia fluxes is possible, e.g., by account of their environmental
dependence [640, 641, 820]. On the other hand, usually, as an event beneath the “standard candle”, an
explosion of a non-rotating WD with the Chandrasekhar mass (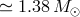 ) is considered. Rotation
of progenitors may increase the critical mass and make SNe Ia less reliable for cosmological
use [154, 155].
) is considered. Rotation
of progenitors may increase the critical mass and make SNe Ia less reliable for cosmological
use [154, 155].
SNe Ia are suggested to be responsible for the production of about 50% of all heavy elements in the
Universe [761]. Mergers of WD binaries are, probably, one of the main mechanisms of the formation of
massive (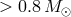 ) WDs and WDs with strong magnetic fields (see, e.g., [322*, 392] for recent studies and
review of earlier work).
) WDs and WDs with strong magnetic fields (see, e.g., [322*, 392] for recent studies and
review of earlier work).
A comparison of SN Ia rates (for the different models of their progenitors) with observations may, in principle, shed light on both the star formation history and on the nature of the progenitors (see, e.g., [663, 873*, 453, 874, 871, 250, 457, 249, 458*]).
Compact binaries are the end products of the evolution of stellar binaries, and the main purpose of the present review is to describe the astrophysical knowledge of their formation and evolution. We shall discuss the present situation with the main parameters determining their evolution and the rates of coalescence of NS/BH binaries and WDs.
The problem is to evaluate as accurately as possible (i) the physical parameters of the coalescing binaries (masses of the components and, if possible, their spins, magnetic fields, etc.) and (ii) the occurrence rate of mergers in the Galaxy and in the local Universe. Masses of NSs in binaries are known with a rather good accuracy of 10% or better from, e.g., pulsar studies [760, 367]; see also [410, 550*] for recent summaries of NS mass measurements.
The case is not so good with the rate of coalescence of relativistic stellar binaries. Unfortunately, there is no way to derive it from first principles – neither the formation rate of the progenitors for compact binaries nor stellar evolution are known well enough. However, the situation is not completely hopeless, especially in the case of NS binary systems. The natural appearance of rotating NSs with magnetic fields as radio pulsars allows one to search for binary pulsars with a secondary compact companion using powerful methods of modern radio astronomy (for example, in dedicated pulsar surveys, such as the Parkes multi-beam pulsar survey [455, 192]).
Based on observational statistics of Galactic pulsar binaries with NS companions, one can evaluate the
Galactic rate of NS binary formation and merging [586*, 505*]. On the other hand, a direct simulation of the
evolution of population of binaries in the Galaxy (the population synthesis method) can also predict the
formation and merger rates of close compact binaries as a function of (numerous) parameters of stellar
formation and evolution. Both kinds of estimates are plagued by badly constrained parameters or selection
effects, but it is, nevertheless, encouraging that most likely Galactic rates of events obtained in two
ways currently differ by a factor of 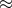 3 only: 80/Myr from observations and 30/Myr from
population synthesis; see [456] for a recent review of observational and theoretical estimates, also
Section 6.
3 only: 80/Myr from observations and 30/Myr from
population synthesis; see [456] for a recent review of observational and theoretical estimates, also
Section 6.
No BH or NS + BH binary systems have been found so far, so merger rates of compact binaries with BHs have been evaluated as yet only from population synthesis studies.
1.1 Formation of stars and end products of their evolution
Let us briefly remind the key facts about star formation and evolution. Approximately 6% of the baryonic
matter in the Universe is confined to stars [217]. Recent observational data suggests that, first, long thin
filaments form inside molecular clouds and, next, these filaments fragment into protostellar cores due to
gravitational instability, if their mass-per-unit length exceeds a certain threshold [14]. An object
may be called a “star” if it is able to generate energy by nuclear fusion at a level sufficient to
halt the contraction [394]. For solar chemical composition, 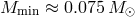 and
and 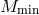 increases if stellar metallicity is lower than solar [81]. Currently, among observed stars, the lowest
dynamically determined mass has component C of a triple system AB Dor:
increases if stellar metallicity is lower than solar [81]. Currently, among observed stars, the lowest
dynamically determined mass has component C of a triple system AB Dor: 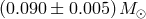 [115].
[115].
The maximum mass of the star is set by the proximity of the luminosity to the Eddington
limit and pulsational instability. For solar chemical composition, this limit is close to
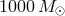 [38, 880].3
But conditions of stellar formation, apparently, define a much lower mass limit. Currently, components of a
[38, 880].3
But conditions of stellar formation, apparently, define a much lower mass limit. Currently, components of a
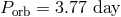 eclipsing binary NGC3603-A1 have maximum dynamically-measured masses:
eclipsing binary NGC3603-A1 have maximum dynamically-measured masses:
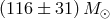 and
and 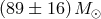 [683]. Since both stars are slightly-evolved main-sequence
objects (WN6h), subject to severe–stellar-wind mass loss, their inferred initial masses could be
higher:
[683]. Since both stars are slightly-evolved main-sequence
objects (WN6h), subject to severe–stellar-wind mass loss, their inferred initial masses could be
higher:  and
and 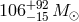 [125]. Indirect evidence, based on photometry and spectral
analysis suggests the possible existence of
[125]. Indirect evidence, based on photometry and spectral
analysis suggests the possible existence of  stars; see [813] for references and
discussion.
stars; see [813] for references and
discussion.
For the metal-free stars that formed first in the Universe (the so-called Population III stars), the upper
mass limit is rather uncertain. For example, it can be below 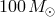 [4, 745], because of the competition
of accretion onto first formed compact core and nuclear burning and influence of UV-irradiation from
nearby protostars. On the other hand, masses of Population III stars could be much higher due to the
absence of effective coolants in the primordial gas. Then, their masses could be limited by pulsations,
though, the upper mass limit remains undefined [703] and masses up to
[4, 745], because of the competition
of accretion onto first formed compact core and nuclear burning and influence of UV-irradiation from
nearby protostars. On the other hand, masses of Population III stars could be much higher due to the
absence of effective coolants in the primordial gas. Then, their masses could be limited by pulsations,
though, the upper mass limit remains undefined [703] and masses up to 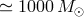 are often inferred in
theoretical studies.
are often inferred in
theoretical studies.
The initial mass function (IMF) of main-sequence stars can be approximated by a power-law
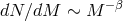 [669] and most simply may be presented taking
[669] and most simply may be presented taking 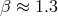 for
for 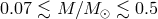 and
and
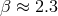 for
for 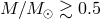 [830]. Note, there are also claims that for the most massive stars
(
[830]. Note, there are also claims that for the most massive stars
(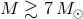 ) the IMF is much steeper – up to
) the IMF is much steeper – up to 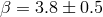 , see [403] and references therein. Estimates
of the current star-formation rate (SFR) in the Galaxy differ depending on the method, but most at
present converge to
, see [403] and references therein. Estimates
of the current star-formation rate (SFR) in the Galaxy differ depending on the method, but most at
present converge to 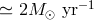 [108, 353, 537]. This implies that in the past the Galactic
SFR was much higher. For the most recent review of observations and models pertinent to
the star-formation process, the origin of the initial mass function, and the clustering of stars,
see [389].
[108, 353, 537]. This implies that in the past the Galactic
SFR was much higher. For the most recent review of observations and models pertinent to
the star-formation process, the origin of the initial mass function, and the clustering of stars,
see [389].
The evolution of a single star and the nature of its compact remnant are determined by the main-sequence
mass 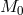 and chemical composition. If
and chemical composition. If 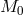 is lower than the minimum mass of stars that ignite carbon
in the core,
is lower than the minimum mass of stars that ignite carbon
in the core, 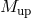 , after exhausting the hydrogen and helium in its core, a carbon-oxygen WD forms. If
, after exhausting the hydrogen and helium in its core, a carbon-oxygen WD forms. If
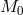 exceeds a certain limiting
exceeds a certain limiting 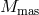 , the star proceeds to form an iron core, which collapses into a NS
or a BH. In the stars of intermediate range between
, the star proceeds to form an iron core, which collapses into a NS
or a BH. In the stars of intermediate range between 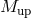 and
and 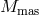 (called “super-AGB
stars”4
) carbon ignites in a partially-degenerate core and converts its matter (completely or partially) into an
oxygen-neon mixture. As conjectured by Paczyński and Ziolkowski [557], the TP-AGB stage of the evolution of
stars5
terminates when shells with positive specific binding energy
(called “super-AGB
stars”4
) carbon ignites in a partially-degenerate core and converts its matter (completely or partially) into an
oxygen-neon mixture. As conjectured by Paczyński and Ziolkowski [557], the TP-AGB stage of the evolution of
stars5
terminates when shells with positive specific binding energy 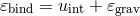 appear in the envelope of
the star. In the latter expression, the internal energy term
appear in the envelope of
the star. In the latter expression, the internal energy term 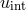 accounts for ideal gas, radiation,
ionisation, dissociation and electron degeneracy, as suggested by Han et al. [266]. The upper limit on
masses of model precursors of CO white dwarfs depends primarily on stellar metallicity, on the treatment of
mixing in stellar interiors, on accepted rates of mass loss during the AGB and on more subtle details of the
models, see, e.g., [278, 279, 338]. Modern studies suggest that for solar metallicity models it does not
exceed
accounts for ideal gas, radiation,
ionisation, dissociation and electron degeneracy, as suggested by Han et al. [266]. The upper limit on
masses of model precursors of CO white dwarfs depends primarily on stellar metallicity, on the treatment of
mixing in stellar interiors, on accepted rates of mass loss during the AGB and on more subtle details of the
models, see, e.g., [278, 279, 338]. Modern studies suggest that for solar metallicity models it does not
exceed 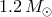 [412].
[412].
As concerns more massive stars, if, due to He-burning in the shell, the mass of the core
reaches 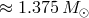 , electron captures by 24Mg and 20Ne ensue and the core collapses to a
neutron star producing an “electron-capture supernova” (ECSN) [488]). Otherwise, an ONe WD
forms.6
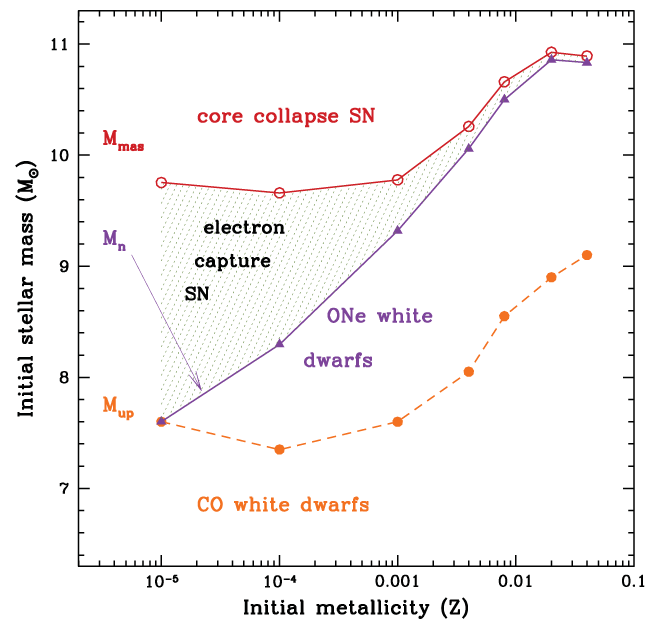
Figure 1* shows, as an example, the endpoints of stellar evolution for intermediate mass stars, computed by
Siess [707, 708].7
Note, results of computations very strongly depend on still uncertain rates of nuclear burning, the
treatment of convection, the rate of assumed stellar-wind mass loss, which is poorly known from
observations for the stars in this transition mass range, as well as on other subtle details of the
stellar models (see discussion in [599, 325, 98, 749]). For instance, zero-age main sequence
(ZAMS) masses of solar-composition progenitors of ECSN found by Poelarends et al. [599] are
, electron captures by 24Mg and 20Ne ensue and the core collapses to a
neutron star producing an “electron-capture supernova” (ECSN) [488]). Otherwise, an ONe WD
forms.6
Figure 1* shows, as an example, the endpoints of stellar evolution for intermediate mass stars, computed by
Siess [707, 708].7
Note, results of computations very strongly depend on still uncertain rates of nuclear burning, the
treatment of convection, the rate of assumed stellar-wind mass loss, which is poorly known from
observations for the stars in this transition mass range, as well as on other subtle details of the
stellar models (see discussion in [599, 325, 98, 749]). For instance, zero-age main sequence
(ZAMS) masses of solar-composition progenitors of ECSN found by Poelarends et al. [599] are
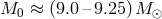 .8
In [295], the lower mass limit for core-collapse SN at solar metallicity is found to be equal to
.8
In [295], the lower mass limit for core-collapse SN at solar metallicity is found to be equal to 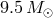 .
According to Jones et al. [326], an
.
According to Jones et al. [326], an 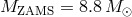 star experiences ECSN, while a
star experiences ECSN, while a 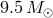 star
evolves to Fe-core collapse. Takahashi et al. [749] find that a
star
evolves to Fe-core collapse. Takahashi et al. [749] find that a 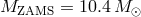 solar metallicity star
explodes as ECSN, while an
solar metallicity star
explodes as ECSN, while an 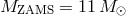 star experiences Fe-core collapse, consistent with Siess’
results.
star experiences Fe-core collapse, consistent with Siess’
results.
ECSN eject only a small amount of Ni (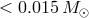 ), and it was suggested that they may be identified
with some subluminous type II-P supernovae [366*]. Tominaga et al. [764] were able to simulate ECSN
starting from first principles and to reproduce their multicolor light curves. They have shown
that observed features of SN 1054 (see [741]) share the predicted characteristics of
ECSN.
), and it was suggested that they may be identified
with some subluminous type II-P supernovae [366*]. Tominaga et al. [764] were able to simulate ECSN
starting from first principles and to reproduce their multicolor light curves. They have shown
that observed features of SN 1054 (see [741]) share the predicted characteristics of
ECSN.
We should enter a caveat that in [412] it is claimed that opacity-related instability at the base of the
convective envelope of 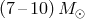 stars may result in ejection of the envelope and, consequently, prevent
ECSN.
stars may result in ejection of the envelope and, consequently, prevent
ECSN.
Electron-capture SNe, if they really happen, presumably produce NS with short spin periods and, if in binaries, systems with short orbital periods and low eccentricities. Observational evidence for ECSN is provided by Be/X-ray binaries, which harbor two subpopulations, typical for post-core-collapse objects and for post-ECSN ones [369]. Low kicks implied for NSs formed via ECSN [597*] may explain the dichotomous nature of Be/X-ray binaries. As well, thanks to low kicks, NS produced via ECSN may be more easily retained in globular clusters (e.g., [397]) or in regions of recent star formation.
The existence of the ONe-variety of WDs, predicted theoretically [24, 488, 301*] and later confirmed by observations [774], is important in the general context of compact-star binary evolution, since their accretion-induced collapse (AIC) may result in the formation of low-mass neutron stars (almost) without natal kicks and may thus be relevant to the formation of NS binaries and low-mass X-ray binaries (see [21, 146*, 798] and references therein). But since for the purpose of detection of gravitational waves they are not different from much more numerous CO WDs, we will, as a rule, not consider them below as a special class.
If 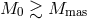 (or
(or 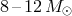 ), thermonuclear evolution proceeds until iron-peak
elements are produced in the core. Iron cores are subject to instabilities (neutronization,
nuclei photo-disintegration), that lead to gravitational collapse. The core collapse of
massive stars results in the formation of a neutron star or, for very massive stars, a black
hole9
and is associated with the brightest astronomical phenomena such as supernova explosions (of type II, Ib,
or Ib/c, according to the astronomical classification based on the spectra and light curves properties). If the
pre-collapsing core retains significant rotation, powerful gamma-ray bursts lasting up to hundreds of seconds
may be produced [850].
), thermonuclear evolution proceeds until iron-peak
elements are produced in the core. Iron cores are subject to instabilities (neutronization,
nuclei photo-disintegration), that lead to gravitational collapse. The core collapse of
massive stars results in the formation of a neutron star or, for very massive stars, a black
hole9
and is associated with the brightest astronomical phenomena such as supernova explosions (of type II, Ib,
or Ib/c, according to the astronomical classification based on the spectra and light curves properties). If the
pre-collapsing core retains significant rotation, powerful gamma-ray bursts lasting up to hundreds of seconds
may be produced [850].
The boundaries between the masses of the progenitors of WDs or NSs and NSs or BHs are fairly uncertain (especially for BHs). Usually accepted typical masses of stellar remnants for single non-rotating solar–chemical-composition stars are summarized in Table 1.
Initial mass [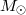 ] ] |
remnant type | typical remnant mass [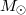 ] ] |
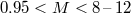 |
WD | 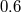 |
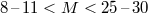 |
NS | 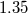 |
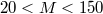 |
BH | 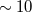 |
Note that the above-described mass ranges for different outcomes of stellar evolution were obtained by computing 1D-models of non-rotating stars. However, all star are rotating and some of them possess magnetic fields, thus, the problem is 2D (or 3D, if magnetic-field effects are considered). For a physical description of effects of rotation on stellar evolution, see [406, 561]. Realistic models with rotation should account for deviation from spherical symmetry, modification of gravity due to centrifugal force, variation of radiative flux with local effective gravity, transfer of angular momentum and transport of chemical species. Up to now, as a rule, models with rotation are computed in a 1D-approximation which, typically, makes use of the fact that in rotating stars mass is constant within isobar surfaces [174]. Regretfully, for low and intermediate-mass stars, model sequences of rotating stars covering evolution to advanced phases of AGB-evolution are absent. However, as noted by Domínguez et al. [156], rotation must strongly influence evolution at core helium exhaustion and the formation of the CO-core stage, when the latter experiences very strong contraction. With an increase of angular velocity, lower pressure is necessary to balance the gravity, hence the temperature of He-burning shells of rotating stars should be lower than in non-rotating stars of the same mass. This extends AGB-lifetime and results in an increase of the mass of CO-cores, i.e., the C-ignition limit may be shifted to lower masses compared to non-rotating stars. Most recently, grids of 1-D models of rotating stars were published, e.g., in [69, 234, 236].
The algorithm and first results of self-consistent calculations of rapidly-rotating 2D stellar models of stars in the early stages of evolution are described, e.g., in [639, 178]. We may note that these models are very important for deriving physical parameters of the stars from astro-seismological data.
For a more detailed introduction to the physics and evolution of stars, the reader is referred to the classical fundamental textbook [121] and to several more modern ones [612, 51, 53, 171, 297, 298]. Formation and physics of compact objects is described in more detail in the monographs [691, 53]. For recent studies and reviews of the evolution of massive stars and the mechanisms of core-collapse supernovae we refer to [406, 79, 320, 881, 212, 721].
1.2 Binary stars
A fundamental property of stars is their multiplicity. Among stars that complete their nuclear evolution in
the Hubble time, the estimated binary fraction varies from 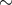 (40 – 60)% for
(40 – 60)% for 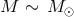 stars [168, 621]
to almost 100% for more massive A/B and O-stars, e.g., [468, 382, 370, 469, 106, 671], (but, e.g.,
in [454, 670], a substantially lower binary fraction for massive stars is claimed).
stars [168, 621]
to almost 100% for more massive A/B and O-stars, e.g., [468, 382, 370, 469, 106, 671], (but, e.g.,
in [454, 670], a substantially lower binary fraction for massive stars is claimed).
Based on the summary of data on binary fraction 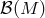 provided in [381, 385, 671], van Haaften
et al. [804] suggested an approximate formula
provided in [381, 385, 671], van Haaften
et al. [804] suggested an approximate formula
The most crucial parameters of binaries include the component separation  and mass ratio
and mass ratio 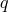 , since
for close binaries they define the outcome of the Roche lobe overflow (in fact, the fate of the system).
The most recent estimates for M-dwarfs and solar-type stars confirm earlier findings that the
, since
for close binaries they define the outcome of the Roche lobe overflow (in fact, the fate of the system).
The most recent estimates for M-dwarfs and solar-type stars confirm earlier findings that the
 -distribution does not strongly deviate from a flat one:
-distribution does not strongly deviate from a flat one: 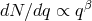 , with
, with 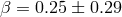 [632]. This distribution is defined by the star-formation process and dynamical evolution in
stellar clusters; see, e.g., [28, 569]. Distribution over
[632]. This distribution is defined by the star-formation process and dynamical evolution in
stellar clusters; see, e.g., [28, 569]. Distribution over  is flat in log between contact and
is flat in log between contact and
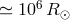 [604*].
[604*].
We note, cautionarily, that all estimates of the binary fraction, mass-ratios of components and distributions over separations of components are plagued by numerous selection effects (see [380] for a thorough simulation of observations and modeling observational bias). A detailed summary of studies of multiplicity rates, distributions over orbital periods and mass ratios of components for different groups of stars may be found in [167].
In binary stars with sufficiently-large orbital separations (“wide binaries”) the presence of the secondary component does not influence significantly the evolution of the components. In “close binaries” the evolutionary expansion of stars leads to the overflow of the critical (Roche) lobe and mass exchange between the components RLOF. Consequently, the formation of compact remnants in close binaries differs from single stars (see Section 3 for more details).
As discussed above, the lower mass limit of NS progenitors is uncertain by several 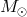 . This limit is
even more uncertain for the BH progenitors. For example, the presence of a magnetar (neutron star with an
extremely large magnetic field) in the open cluster Westerlund 1 means that it descends from a star that is
more massive than the currently-observed most-massive main-sequence cluster stars (because for
a massive star the duration of the main-sequence stage is inversely proportional to its mass
squared). The most massive main-sequence stars in this stellar cluster are found to have masses as
high as
. This limit is
even more uncertain for the BH progenitors. For example, the presence of a magnetar (neutron star with an
extremely large magnetic field) in the open cluster Westerlund 1 means that it descends from a star that is
more massive than the currently-observed most-massive main-sequence cluster stars (because for
a massive star the duration of the main-sequence stage is inversely proportional to its mass
squared). The most massive main-sequence stars in this stellar cluster are found to have masses as
high as 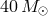 , suggesting
, suggesting 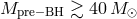 [643]. On the other hand, it was speculated,
based on the properties of X-ray sources, that in the initial mass range
[643]. On the other hand, it was speculated,
based on the properties of X-ray sources, that in the initial mass range 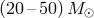 the mass
of pre-BHs may vary depending on such poorly-known parameters as rotation or magnetic
fields [176].
the mass
of pre-BHs may vary depending on such poorly-known parameters as rotation or magnetic
fields [176].
Binaries with compact remnants are primary potentially-detectable GW sources (see Figure 2*). This
figure plots the sensitivity of ground-based interferometers, LIGO, as well as the space laser
interferometers LISA and eLISA, in the terms of dimensionless GW strain  measured over one
year.10
The strongest Galactic sources at all frequencies are the most compact NS binaries, WD binaries, and (still
hypothetically) BHs. NS/BH binary systems are formed from initially massive binaries, while WD binaries
descend from low-mass binaries.
measured over one
year.10
The strongest Galactic sources at all frequencies are the most compact NS binaries, WD binaries, and (still
hypothetically) BHs. NS/BH binary systems are formed from initially massive binaries, while WD binaries
descend from low-mass binaries.
In this review we shall concentrate on the formation and evolution of compact star binaries most relevant to GW studies. The article is organized as follows. We start in Section 2 with a review of the main observational data on NS binaries, especially measurements of masses of NSs and BHs, which are most important for the estimate of the amplitude of the expected GW signal. We briefly discuss empirical methods to determine the NS binary coalescence rate. The basic principles of stellar binary evolution are discussed in Section 3. Then, in Section 4 we describe the evolution of massive stellar binaries. Next, we discuss the Galactic rate of formation of binaries with NSs and BHs in Section 5. Theoretical estimates of detection rates for mergers of relativistic stellar binaries are discussed in Section 6. Further, we proceed to the analysis of the formation of short-period binaries with WD components in Section 7, and consider observational data on WD binaries in Section 8. A model for the evolution of interacting double-degenerate systems is presented in Section 9. In Section 10 we describe gravitational waves from compact binaries with white dwarf components. Section 11 is devoted to the modeling of optical and X-ray emissions of AM CVn-stars. Our conclusions follow in Section 12.

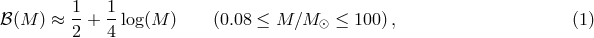
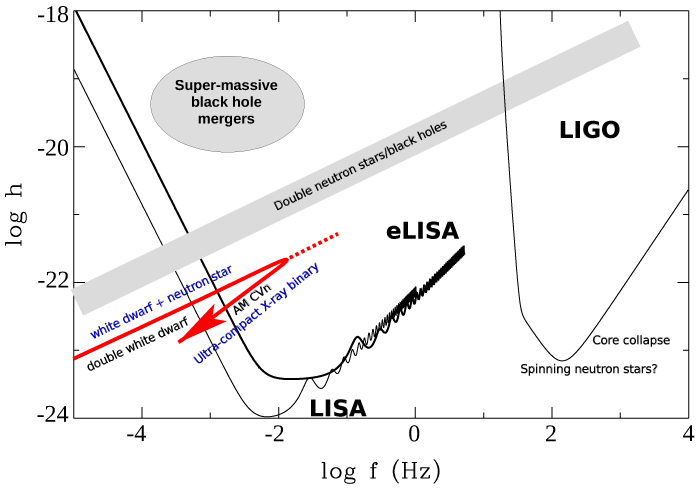
 –
– diagram occupied by
some potential GW sources. (Courtesy G. Nelemans).
diagram occupied by
some potential GW sources. (Courtesy G. Nelemans).