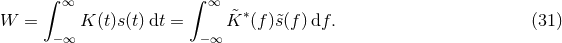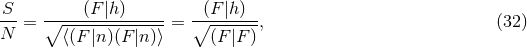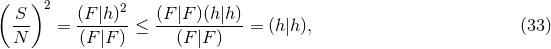4 Summary of Low-Frequency Gravitational-Wave Sources
The low-frequency GW band, which we define as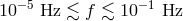 , is astrophysically appealing
because it is populated by a large number of sources, which are relevant to understanding stellar evolution
and stellar populations, to probing the growth and evolution massive black holes (MBH) and their links to
galaxies, and possibly to observing the primordial universe. Space-based interferometric detectors such as
LISA and eLISA are sensitive over this entire band. Figure 2
, is astrophysically appealing
because it is populated by a large number of sources, which are relevant to understanding stellar evolution
and stellar populations, to probing the growth and evolution massive black holes (MBH) and their links to
galaxies, and possibly to observing the primordial universe. Space-based interferometric detectors such as
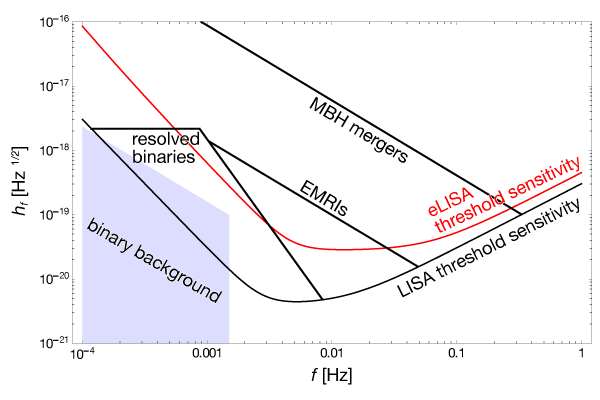
LISA and eLISA are sensitive over this entire band. Figure 2 illustrates the GW strength, as a function of
GW frequency, for a variety of interesting sources classes. The LISA and eLISA baseline sensitivities are
overlaid, illustrating the potential reach of interferometric detectors. The area above the baseline curve is
generically called “discovery space;” the height of a source above the curve provides a rough
estimate of the expected signal-to-noise ratio (SNR) with which the source could be detected using
matched-filtering techniques. SNR and matched filtering are important concepts that underlie
much of what will be discussed elsewhere in this review, so we explain them briefly in this
section.
illustrates the GW strength, as a function of
GW frequency, for a variety of interesting sources classes. The LISA and eLISA baseline sensitivities are
overlaid, illustrating the potential reach of interferometric detectors. The area above the baseline curve is
generically called “discovery space;” the height of a source above the curve provides a rough
estimate of the expected signal-to-noise ratio (SNR) with which the source could be detected using
matched-filtering techniques. SNR and matched filtering are important concepts that underlie
much of what will be discussed elsewhere in this review, so we explain them briefly in this
section.
In a particular GW search based on the evaluation of a detection “statistic” 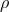 , the detection SNR of a
GW signal is defined as the ratio of the expectation value
, the detection SNR of a
GW signal is defined as the ratio of the expectation value ![E[ρ]](article211x.gif) when the signal is present to the
root-mean-square average of
when the signal is present to the
root-mean-square average of 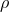 when the signal is absent. Typically the output
when the signal is absent. Typically the output  of the detector is
modeled as the sum of a signal
of the detector is
modeled as the sum of a signal 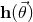 , depending on parameters
, depending on parameters 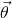 , and of instrumental noise
, and of instrumental noise 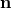 . It is
normally assumed that the noise is stationary and Gaussian, and that it has uncorrelated frequency
components
. It is
normally assumed that the noise is stationary and Gaussian, and that it has uncorrelated frequency
components 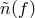 . Under these assumptions, the statistical fluctuations of noise are completely
determined by the one-sided power spectral density (PSD)
. Under these assumptions, the statistical fluctuations of noise are completely
determined by the one-sided power spectral density (PSD) 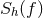 , which is defined by the equation
, which is defined by the equation
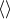 denotes the expectation value over realizations of the noise (also known as the ensemble
average), and the asterisk denotes complex conjugation. This gives rise to a natural definition of an inner
product on the space of possible waveform templates,
In particular, the (sampling) probability of a given realization of noise
denotes the expectation value over realizations of the noise (also known as the ensemble
average), and the asterisk denotes complex conjugation. This gives rise to a natural definition of an inner
product on the space of possible waveform templates,
In particular, the (sampling) probability of a given realization of noise 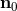 is just
Searching the data for GW signals usually involves applying a linear filter
is just
Searching the data for GW signals usually involves applying a linear filter 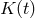 to compute the statistic
Redefining the filter by setting
to compute the statistic
Redefining the filter by setting 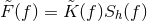 yields the overlap
yields the overlap 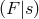 . The corresponding SNR is
and from the Cauchy–Schwarz inequality we have
with equality when
. The corresponding SNR is
and from the Cauchy–Schwarz inequality we have
with equality when 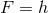 . This shows that the matched filter obtained when
. This shows that the matched filter obtained when 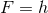 is the optimal
linear statistic to search for the signal
is the optimal
linear statistic to search for the signal 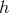 in noise characterized by the PSD
in noise characterized by the PSD 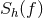 . Furthermore, the
optimal matched-filtering SNR is just
. Furthermore, the
optimal matched-filtering SNR is just 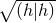 . Future references to SNR in this review will always refer to
this mathematical object.
. Future references to SNR in this review will always refer to
this mathematical object.
 . The discovery space is delineated by the LISA
threshold sensitivity curve [277
. The discovery space is delineated by the LISA
threshold sensitivity curve [277 ] in black, and by the eLISA sensitivity curve in red [21] (the
curves were produced using the online sensitivity curve and source plotting website [321]). This
region is populated by a wealth of strong sources, often in large numbers, including mergers of
MBHs, EMRIs of stellar-scale compact objects into MBHs, and millions of close-orbiting binary
systems in the galaxy. Thousands of the strongest signals from these galactic binary systems should
be individually resolvable, while the combined signals of millions of them produce a stochastic
background at low frequencies. These systems provide ample opportunities for astrophysical tests
of GR for gravitational-field strengths that are not well characterized and studied in conventional
astronomy.
] in black, and by the eLISA sensitivity curve in red [21] (the
curves were produced using the online sensitivity curve and source plotting website [321]). This
region is populated by a wealth of strong sources, often in large numbers, including mergers of
MBHs, EMRIs of stellar-scale compact objects into MBHs, and millions of close-orbiting binary
systems in the galaxy. Thousands of the strongest signals from these galactic binary systems should
be individually resolvable, while the combined signals of millions of them produce a stochastic
background at low frequencies. These systems provide ample opportunities for astrophysical tests
of GR for gravitational-field strengths that are not well characterized and studied in conventional
astronomy.The three main types of GW sources in the low-frequency band are massive black hole (MBH) binaries, extreme mass-ratio inspirals (EMRIs), and galactic binaries.
MBH binaries comprise two supermassive and nearly equal-mass black holes. These systems typically
form following the merger of two galaxies, when the MBHs originally in the centers of the two
pre-merger galaxies reach the center of the merged galaxy and form a binary. These binaries
generate very strong GW emission and can be detected by space-based GW detectors out to
cosmological distances. The signals will be observed from the time they enter the detector band,
at  , until the signal cuts off after the objects have merged. The systems evolve
through an inspiral, as the objects orbit one another on a nearly circular orbit of gradually
shrinking radius, followed by the merger, and then the ringdown. This last phase of the signal is
generated as the black-hole merger product, which is initially perturbed in a highly asymmetric
state, settles down to a stationary and axisymmetric configuration. During the inspiral phase
the field strength is moderate and the velocity is low compared to the speed of light, so the
system can be modeled using post-Newtonian theory [289, 477, 263, 478, 84
, until the signal cuts off after the objects have merged. The systems evolve
through an inspiral, as the objects orbit one another on a nearly circular orbit of gradually
shrinking radius, followed by the merger, and then the ringdown. This last phase of the signal is
generated as the black-hole merger product, which is initially perturbed in a highly asymmetric
state, settles down to a stationary and axisymmetric configuration. During the inspiral phase
the field strength is moderate and the velocity is low compared to the speed of light, so the
system can be modeled using post-Newtonian theory [289, 477, 263, 478, 84 ]. During the
merger the system is highly dynamical and requires full nonlinear modeling using numerical
relativity [367, 102, 42, 398]. The final ringdown emission can then be computed using black-hole
perturbation theory, since the perturbations of the object away from stationarity and axisymmetry are
small.
]. During the
merger the system is highly dynamical and requires full nonlinear modeling using numerical
relativity [367, 102, 42, 398]. The final ringdown emission can then be computed using black-hole
perturbation theory, since the perturbations of the object away from stationarity and axisymmetry are
small.
There have been several attempts to develop models that include all three phases of the emission in a single framework. The effective one-body (EOB) model was initially developed analytically, and is based on the idea of modeling the dynamics of a binary by describing the relative motion of binary components as the motion of a test particle in an external spacetime metric (the metric of the “effective” single body) [99, 100, 141]. The EOB model includes a smooth transition to plunge and merger, and then a sharp transition to ringdown. The model incorporates a number of free parameters that have now been estimated by comparison to numerical-relativity simulations [101, 144]. The initial “effective-one-body numerical-relativity” (EOBNR) model described non-spinning black holes, but the formalism has now been extended to include the effects of non-precessing spins [345, 51, 438, 44]. The other model that includes all phases of the waveform is the phenomenological inspiral-merger-ringdown (pIMR) developed by Ajith et al. [4, 5]. This model was constructed by directly fitting an ansatz for the frequency-domain waveform, which was motivated by analytic and numerical results, to the output of numerical relativity simulations. This model has also now been extended to include the effect of non-precessing spins [393].
EMRIs consist of stellar-mass (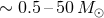 ) compact objects, either white dwarfs, neutron stars, or
black holes, that orbit MBHs. These are expected to occur in the centers of quiescent galaxies, in which a
central MBH is surrounded by a cluster of stars. Interactions between these stars can put compact objects
onto orbits that come very close to the MBH, leading to gravitational capture. EMRIs are
not as strong GW emitters as MBH binaries, but are expected to be observable (if sufficiently
close to us) for the final few years before the smaller object merges with the central MBH,
when the emitted GWs are in the most sensitive part of the frequency range of space-based
detectors. EMRIs will also undergo inspiral, merger, and ringdown, but the signals from the
latter two phases are likely to be too weak to be detected. During the inspiral, EMRIs are also
expected to be on eccentric and inclined orbits, which colors the emitted GWs with multiple
frequency components. During an EMRI, the smaller object spends many thousands of orbits
in the strong-field regime, where its velocity is a significant fraction of the speed of light, so
post-Newtonian waveforms are inapplicable. However, the extreme mass ratio means that black-hole
perturbation theory can be used to compute waveforms, using the mass ratio as an expansion
parameter [362
) compact objects, either white dwarfs, neutron stars, or
black holes, that orbit MBHs. These are expected to occur in the centers of quiescent galaxies, in which a
central MBH is surrounded by a cluster of stars. Interactions between these stars can put compact objects
onto orbits that come very close to the MBH, leading to gravitational capture. EMRIs are
not as strong GW emitters as MBH binaries, but are expected to be observable (if sufficiently
close to us) for the final few years before the smaller object merges with the central MBH,
when the emitted GWs are in the most sensitive part of the frequency range of space-based
detectors. EMRIs will also undergo inspiral, merger, and ringdown, but the signals from the
latter two phases are likely to be too weak to be detected. During the inspiral, EMRIs are also
expected to be on eccentric and inclined orbits, which colors the emitted GWs with multiple
frequency components. During an EMRI, the smaller object spends many thousands of orbits
in the strong-field regime, where its velocity is a significant fraction of the speed of light, so
post-Newtonian waveforms are inapplicable. However, the extreme mass ratio means that black-hole
perturbation theory can be used to compute waveforms, using the mass ratio as an expansion
parameter [362 , 394].
, 394].
Stellar binaries in our own galaxy with two compact object components will also be sources for
space-based detectors if the binary period is appropriate (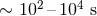 ). The binary components must be
compact to ensure that the binary can reach such periods without undergoing mass transfer.
Theoretical models and observational evidence suggest that there may be many millions of
such binaries that are potential sources. These systems are not expected to evolve significantly
over the typical lifetime of a space mission, and will therefore produce continuous and mostly
monochromatic GW sources in the band of space-based detectors. For a small number of systems it
will be possible to observe a linear drift in frequency over the observation time, due to either
GW-driven inspiral or mass transfer. These systems remain in the weak-field regime through their
observation, and their emission can be represented accurately using the quadrupole formula [353, 354].
In addition to what they will teach us about astrophysics, all these sources are prospective
laboratories for testing gravitation. The different character of sources in each class provides different
opportunities. MBH binaries are strong-field systems that yield GW signals with large SNRs, making
signal detection and characterization less ambiguous than for weaker sources. This will allow
detailed explorations of waveform deviations from the predictions of GR. EMRIs provide detailed
probes of MBH spacetimes, thanks to the large number of strong-field waveform cycles that will
be observable from these systems. Individually-resolvable compact galactic binaries can also
be used to test GR, because their waveforms and evolution are well understood and easily
described using model templates. In addition, many such binaries may be detectable with both GW
and EM observatories, providing opportunities to test the propagation of GWs relative to EM
signals.
). The binary components must be
compact to ensure that the binary can reach such periods without undergoing mass transfer.
Theoretical models and observational evidence suggest that there may be many millions of
such binaries that are potential sources. These systems are not expected to evolve significantly
over the typical lifetime of a space mission, and will therefore produce continuous and mostly
monochromatic GW sources in the band of space-based detectors. For a small number of systems it
will be possible to observe a linear drift in frequency over the observation time, due to either
GW-driven inspiral or mass transfer. These systems remain in the weak-field regime through their
observation, and their emission can be represented accurately using the quadrupole formula [353, 354].
In addition to what they will teach us about astrophysics, all these sources are prospective
laboratories for testing gravitation. The different character of sources in each class provides different
opportunities. MBH binaries are strong-field systems that yield GW signals with large SNRs, making
signal detection and characterization less ambiguous than for weaker sources. This will allow
detailed explorations of waveform deviations from the predictions of GR. EMRIs provide detailed
probes of MBH spacetimes, thanks to the large number of strong-field waveform cycles that will
be observable from these systems. Individually-resolvable compact galactic binaries can also
be used to test GR, because their waveforms and evolution are well understood and easily
described using model templates. In addition, many such binaries may be detectable with both GW
and EM observatories, providing opportunities to test the propagation of GWs relative to EM
signals.
Many approaches to constraining alternative theories are proposed as null experiments, where the assumption is that GW observations will validate the predictions of GR to the level of the detector’s instrumental noise. The size of residual deviations from GR is then constrained by the size of the errors in the GW measurement. However, in order to perform these null experiments, it is necessary to have accurate models for the GWs predicted in GR. The appropriate modeling scheme, assuming GR and a system in vacuum, depends on the system under consideration, as described above. Additional modeling complications arise from astrophysical phenomena, since tidal coupling, extended body effects, and mass transfer can all leave an impact on source evolution, complicating the interpretation of the observed GWs.
The very capabilities of space-based detectors also introduce complications: in contrast to
the rare appearance of GW signals in the output of ground-based interferometers, the data
from space-based missions will contain the superposition of millions of individual continuous
signals. We expect that we will be able to resolve individually thousands of the strongest sources.
Thus, we must deal with problems of confusion (where the presence of many interfering sources
complicates their individual detection), subtraction (where strong signals must be carefully
modeled and removed from the data before weaker sources, overlapping in frequency, become
visible), and global fit (where the parameters of overlapping sources have correlated errors that
must be varied together in searches and parameter-estimation studies). These issues have not
escaped the attention of LISA scientists, and have been tackled both theoretically [38], and in
a practical program of mock data challenges [37, 39 , 450]. All these issues in data analysis
and modeling will impact the prospects for tests of GR using the observations. Little work
has been done to date to estimate these impacts, but we will discuss relevant studies where
appropriate.
, 450]. All these issues in data analysis
and modeling will impact the prospects for tests of GR using the observations. Little work
has been done to date to estimate these impacts, but we will discuss relevant studies where
appropriate.
In the remainder of this section we will briefly describe the three principal source classes expected in the low-frequency band. For each source type, we will discuss the astrophysics of the systems, the estimated event rates for (e)LISA observations, their potential astrophysical implications, and their applications to testing GR, with pointers to later sections that describe the tests in more detail. We focus on the three types of source that we introduced above and that are most likely, from an astrophysical point of view, to be observed by LISA-like observatories: MBH coalescences, EMRIs, and galactic binaries.
It is possible that a space-based detector like LISA or eLISA could detect other sources that could be
used for tests of relativity. For instance, if intermediate-mass black holes with mass between 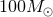 and
and
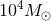 do exist, they could be observed as intermediate-mass-ratio inspirals (IMRIs) when they inspiral
into the MBHs in the centers of galaxies [18
do exist, they could be observed as intermediate-mass-ratio inspirals (IMRIs) when they inspiral
into the MBHs in the centers of galaxies [18 ]. These systems would have the potential for the
same kind of tests of fundamental physics as EMRIs, but with considerably larger SNR at
the same distance and hence would be observable to much greater distances. Cosmological
GW backgrounds might also be observed, generated by processes occurring at the TeV scale in
the early universe, which would provide constraints on the physics of the early universe and
inflation. We refer the reader to [21, 20] for discussions of these sources. We regard them as
somewhat more speculative than the other three source types and we do not consider them further
here.
]. These systems would have the potential for the
same kind of tests of fundamental physics as EMRIs, but with considerably larger SNR at
the same distance and hence would be observable to much greater distances. Cosmological
GW backgrounds might also be observed, generated by processes occurring at the TeV scale in
the early universe, which would provide constraints on the physics of the early universe and
inflation. We refer the reader to [21, 20] for discussions of these sources. We regard them as
somewhat more speculative than the other three source types and we do not consider them further
here.
4.1 Massive black-hole coalescences
Most (if not all) galactic nuclei come to harbor a MBH during their evolution [296, 377], and individual galaxies are expected to undergo multiple mergers over their lifetime. It follows that the formation of MBH binaries following galaxy mergers is an expected outcome. The mergers of such binaries will be among the strongest sources of low-frequency GWs. The rate at which MBH binaries merge in the universe is uncertain at best, but these events will be detectable by LISA-like detectors to extremely large distances, probing an enormous volume of the visible universe. The detection of any MBH mergers, even at a low rate, would produce interesting astrophysical results.
For a circular binary, the mass of a system that merges at a frequency 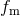 is roughly
is roughly
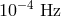 to a few
to a few
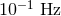 , which sets the sensitive mass range to
, which sets the sensitive mass range to 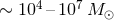 .
.
MBH mergers as low-frequency GW sources.
Predictions for the observable population of MBH mergers are based on merger-tree structure-formation models. The overall merger rate depends on the detailed mechanisms of evolutionary growth of the MBH population. The energy budgets of active galactic nuclei suggest that MBHs could grow by efficient accretion processes [490], while other considerations suggest that mergers could contribute significantly to their early growth [247]. Studies of early cosmic structure [298] indicate that MBHs form from the coalescence of many smaller seed black holes. Many models based on this idea have been developed and simulated numerically [216, 460, 437, 60]. The number of events detectable by LISA was estimated to be in the range 3 – 300 per year [406], with a spectrum of masses in the range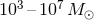 . The predicted event rate is not much different for eLISA [20], although some of the
marginally detectable events involving lighter black holes at high redshift will no longer be
observable.
Figure 3
. The predicted event rate is not much different for eLISA [20], although some of the
marginally detectable events involving lighter black holes at high redshift will no longer be
observable.
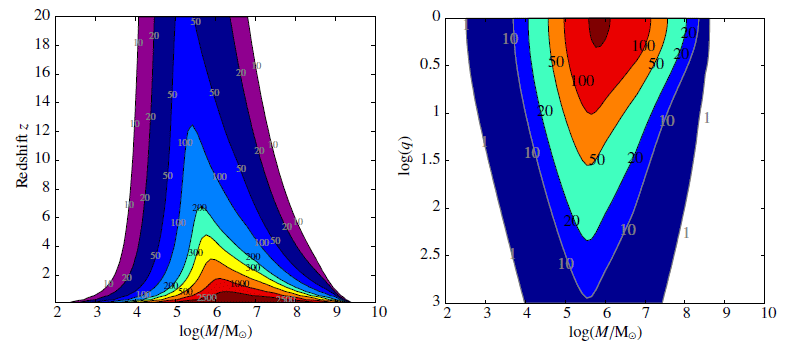
Figure 3 shows contours of constant SNR (as seen by eLISA) in the redshift–total-mass plane for
equal-mass, nonspinning MBH mergers (left panel) and in the total-mass–mass-ratio plane for MBH
mergers at a fixed redshift of
shows contours of constant SNR (as seen by eLISA) in the redshift–total-mass plane for
equal-mass, nonspinning MBH mergers (left panel) and in the total-mass–mass-ratio plane for MBH
mergers at a fixed redshift of 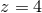 (right panel). For typical events at
(right panel). For typical events at 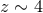 , the SNRs of eLISA
observations are expected to exceed 100 for total masses
, the SNRs of eLISA
observations are expected to exceed 100 for total masses 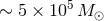 . For comparable-mass mergers at
lower redshifts, eLISA observations could exceed SNRs of 1000. SNRs for LISA are typically factors of 2 – 3
higher. For total mass
. For comparable-mass mergers at
lower redshifts, eLISA observations could exceed SNRs of 1000. SNRs for LISA are typically factors of 2 – 3
higher. For total mass 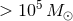 , a significant fraction of this SNR comes from the final merger and
ringdown phase.
, a significant fraction of this SNR comes from the final merger and
ringdown phase.
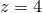 . Image reproduced
by permission from [21].
. Image reproduced
by permission from [21]. The practical detection of MBH systems will have to rely on careful data analysis. Several detection
algorithms for MBH binaries have been studied by a number of different groups, encouraged in part by the
Mock LISA Data Challenges [39 ]. These include Markov Chain Monte Carlo techniques [132],
time-frequency analysis [96], particle-swarm optimization [196], nested sampling [172], and genetic
algorithms [356]. The most recent mock-data challenge included multiple spinning MBH binary signals in
the same dataset, and multiple groups demonstrated their ability to recover these binaries from the LISA
data stream [39
]. These include Markov Chain Monte Carlo techniques [132],
time-frequency analysis [96], particle-swarm optimization [196], nested sampling [172], and genetic
algorithms [356]. The most recent mock-data challenge included multiple spinning MBH binary signals in
the same dataset, and multiple groups demonstrated their ability to recover these binaries from the LISA
data stream [39 ].
].
Astrophysics using MBH mergers.
GW observations will directly probe the character of the black holes on scales comparable to the size of the event horizons. How the information about the black holes (such as their spin and mass) is encoded in the waveform is a topic of much focused research (see, e.g., [275, 411, 117, 421] for reviews). According to GR, any astrophysical black hole is fully described by just ten numbers, encoding its mass, position, momentum, and spin. Correspondingly, a binary is fully described by 20 parameters, three of which, related to its center-of-mass momentum, cannot be measured from the GW signal. Einstein’s theory of gravity thus predicts a 17-dimensional parameter space of possible signals. The precision with which GW observations will be able to extract the parameters characterizing a MBH binary system has been the subject of extensive investigation. The first comprehensive study [137] considered the LISA determination of the sky location, luminosity distance, and mass of MBH systems, using a simple model of LISA’s orbital motion around the sun. Later studies [322] considered the angular resolution of detectors in precessing-plane configurations like LISA’s, as well as ecliptic-plane interferometers that lie flat in the ecliptic as they orbit the sun. More recent studies have considered more complete MBH-binary waveforms with spin effects, higher harmonics, and with merger and ringdown signals, and have looked at the effect of these corrections on LISA’s parameter-estimation ability [455, 29, 442, 365, 33, 266, 307]. These papers indicate that observations with a LISA-like detector will be able to determine the masses of the two binary components to 0.01 – 0.1%, the spins to 0.001 – 0.1%, the sky position of the binary to 1 – 10 deg2, and the luminosity distance to 0.1 – 10%. For eLISA, the corresponding results are 0.1 – 1%, 1 – 10%, 10 – 100 deg2 and 10 – 100%. Such precise measurements will provide unprecedented information about MBHs in the universe.These parameter-estimation studies witness the quality of the information that will be available to astrophysicists for the phenomenological exploration of the astrophysical character of MBH systems. Of particular interest is the evolution of black-hole spins and the final spin of merger remnants [80, 242], which GW observations should be able to shed some light on. In addition, the set of detected MBH merger systems will provide constraints on the formation history of MBHs [360, 359, 241, 308, 355]. In [405, 198] it was shown that a detector like LISA would be able to tell with high confidence the difference between ten different models for the growth of structure in the universe. For many of the models, the difference would already be apparent after as little as three months of data collection. A similar differentiation among models would also be possible with eLISA [21], and both detectors will also be able to constrain “mixed models” combining different MBH population models. MBH binaries can also be used as standard candles to probe cosmological evolution [234].
Testing relativity using MBH mergers.
MBH mergers are good laboratories for testing relativity because of the high SNR expected for the signals and the correspondingly large volume within which they can be observed. This makes them good systems for constraining GW polarizations (see Section 5.1.1) since relatively weak alternative-polarization signals could in principle be detected. They are also excellent systems for testing GW-propagation effects, such as subluminal propagation speeds and the presence of parity violations, since these effects accumulate with distance and MBH binaries can be seen to very high redshifts (see Section 5.1.2). MBH binaries are also well suited for generic tests of GR based on measuring the evolution of inspiral phasing and checking it for consistency with general-relativistic predictions, since the high SNR allows a large number of phasing parameters to be measured (see Section 5.2). MBH binaries are also the only systems for which the quasinormal ringdown radiation, generated as the highly-distorted post-merger black hole settles down into a quiescent state, will be detectable. Although the amount of energy deposited into different ringdown modes is not well understood, the frequencies and decay rates of the ringdown modes are predicted precisely in GR from perturbation theory. The mass and spin of the final black hole can be measured from a single ringdown mode; if multiple modes are detected, the system can be used to check for consistency with the Kerr metric, and therefore provides a probe of black-hole structure. For a comprehensive review of black-hole ringdowns, we refer the reader to [270 , 337
, 337 , 76]. A more
detailed discussion of the use of ringdown radiation to probe black-hole structure may be found in
Section 6.3.
, 76]. A more
detailed discussion of the use of ringdown radiation to probe black-hole structure may be found in
Section 6.3.
Last, the space-based GW observation of MBH binaries offers an unprecedented opportunity to probe the fully-dynamic strong-field regime of GR by comparison of the observed merger signals to the predictions of numerical relativity. This is an area that has not yet been explored in any detail and so we will not discuss it further in this review. However, it is an important subject that should be carefully explored before a space-based GW detector finally becomes a reality.
4.2 Extreme-mass-ratio inspirals
An EMRI is the GW-emitting inspiral of a stellar-mass compact object, either a black hole, neutron star or
white dwarf, into a MBH in the center of a galaxy. A main-sequence star with mean density
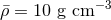 will be tidally disrupted by a black hole of mass
will be tidally disrupted by a black hole of mass  at a distance of
at a distance of 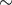 25
Schwarzschild radii [187
25
Schwarzschild radii [187 ]. At such separations an extra-galactic EMRI system will not be generating
detectable amounts of gravitational radiation in the low-frequency band. The tidal-disruption radius
increases with decreasing stellar density and decreasing central–black-hole mass, and the reference values
used above are at the upper end of suitable values for main-sequence stars and mHz GW sources.
Therefore, it is not expected that the inspiral of a main-sequence star will be a candidate for an
EMRI, with the possible exception of such sources in the galactic center, which is sufficiently
nearby that radiation from an object orbiting at several tens of Schwarzschild radii might be
detectable [187].
]. At such separations an extra-galactic EMRI system will not be generating
detectable amounts of gravitational radiation in the low-frequency band. The tidal-disruption radius
increases with decreasing stellar density and decreasing central–black-hole mass, and the reference values
used above are at the upper end of suitable values for main-sequence stars and mHz GW sources.
Therefore, it is not expected that the inspiral of a main-sequence star will be a candidate for an
EMRI, with the possible exception of such sources in the galactic center, which is sufficiently
nearby that radiation from an object orbiting at several tens of Schwarzschild radii might be
detectable [187].
EMRIs occur in the dense stellar clusters that are found surrounding the black holes in the cores of galaxies, and are triggered by a variety of processes:
- Direct capture: This is the “standard” scenario, in which two-body encounters in the stellar cluster gradually perturb the compact-object orbits, changing their angular momentum. This can put a compact object onto an orbit that passes very close to the central black hole. The orbit loses energy and angular momentum in bursts of gravitational radiation emitted near periapse, and if sufficient energy is radiated, the object can be left on an orbit that is bound to the central black hole. It will then gradually inspiral into the central black hole as it loses orbital energy and angular momentum to GW emission.
- Tidal splitting of binaries: Galactic-center stellar clusters will also contain binaries, which can similarly be displaced onto orbits that pass close to the central MBH. If this happens, the binary will typically be disrupted, with one object remaining bound to the MBH and the other becoming unbound and being flung out with high velocity. If this happens to a binary containing one or two compact objects, the captured compact object will be left on a fairly tightly bound orbit a few hundred astronomical units from the MBH, and will become an EMRI on a nearly circular orbit [312].
- Tidal stripping of giant stars: Giant stars typically have a compact core surrounded by a diffuse hydrogen envelope. If such a star passes close to a MBH, the envelope can be partially or completely stripped by the tidal interaction with the MBH. This will deposit the dense core, which is essentially a white dwarf, on a close orbit about the MBH; the system will eventually become an EMRI [145, 150],
- In-situ formation: If a MBH has a massive accretion disc, the disc can become unstable to star formation. Stars formed in such a way are biased toward higher masses and will therefore tend to form black-hole remnants. These remnants will be in circular, equatorial orbits around the central MBH, and will inspiral as EMRIs under GW emission.
More details on all of these processes and further references can be found in [18 , 16
, 16 ].
].
EMRIs as low-frequency GW sources.
The intrinsic rate at which EMRIs occur depends on a lot of astrophysics that is rather poorly understood. The rate is strongly influenced by several processes, including:- Mass segregation: Stellar encounters tend on average to lead to equipartition of energy. This
means that after a two-body interaction the heavier object tends to be moving slower, and
the lighter object tends to be moving faster. If this occurs for two objects around a MBH,
the heavier object sinks in the potential of the MBH and the lighter object moves further out.
Components of the stellar distribution thus become segregated according to their mass. The
more massive objects, which tend to be the black holes, end up closer to the MBH and are
captured preferentially [18
 , 188, 313].
, 188, 313].
- Triaxiality: The centers of galaxies tend to be approximately spherically symmetric, but on larger scales galactic nuclei can be triaxial. Orbits in triaxial potentials tend to be centrophilic: they have a tendency to pass close to the center. This process can funnel stars that are further away from the MBH toward the center and hence increase the inspiral rate [233, 364].
- Resonant relaxation: In the standard relaxation picture, each encounter is random and uncorrelated, so stars undergo a random walk. The process is driven by the diffusion of energy which then leads to angular-momentum transfer. However, in a stellar cluster around a MBH, each star will be on a Keplerian orbit, which is a fixed ellipse in space. The orbits of two nearby stars will thus exert correlated torques on one another, which can lead to a direct resonant evolution of the angular momentum [375, 376]. This process can lead to a much more rapid diffusion of orbits into the regime where they can become EMRIs.
- The “Schwarzschild barrier”: Resonant relaxation relies on the orbits having commensurate radial and azimuthal frequencies, so they remain in fixed planes over multiple orbits. In the strong-field potential of a massive object, orbits are no longer Keplerian but undergo significant perihelion precession. Resonant relaxation is only efficient in the regime where precession is negligible. The “Schwarzschild barrier” refers to the boundary between orbits with and without significant precession. Inside this point resonant relaxation is strongly quenched, potentially reducing inspiral rates [310].
A full description of these processes and their relative importance can be found in [18 , 16
, 16 ]. The range of EMRI
rates per galaxy reported in the literature is
]. The range of EMRI
rates per galaxy reported in the literature is 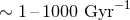 for black holes, and
for black holes, and 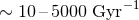 for white dwarfs, with most recent best-guess estimates of
for white dwarfs, with most recent best-guess estimates of 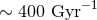 /
/ 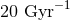 /
/ 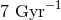 for
black holes / white dwarfs / neutron stars [235
for
black holes / white dwarfs / neutron stars [235 , 19].
, 19].
Uncertainties in the event rate come not only from the intrinsic rate of capture, but also from
uncertainties in the number density of black holes of suitable mass. At present, only three objects in the
range 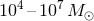 are known from kinematic measurements [173]. Using galaxy luminosity functions, the
luminosity–velocity-dispersion relation and the MBH-mass–velocity dispersion relation, one infers the
number density of MBHs in the LISA mass range to be roughly constant per logarithmic mass unit, and
equal to a few
are known from kinematic measurements [173]. Using galaxy luminosity functions, the
luminosity–velocity-dispersion relation and the MBH-mass–velocity dispersion relation, one infers the
number density of MBHs in the LISA mass range to be roughly constant per logarithmic mass unit, and
equal to a few 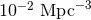 . However, when black holes merge, the asymmetric radiation
of linear momentum over the final few orbits imparts linear momentum (a “merger kick”) to
the remnant black hole. These kicks can be large enough to eject the black hole from its host
galaxy. If these black-hole merger kicks are significant for black holes merging in the centers
of galaxies, the number of black holes with
. However, when black holes merge, the asymmetric radiation
of linear momentum over the final few orbits imparts linear momentum (a “merger kick”) to
the remnant black hole. These kicks can be large enough to eject the black hole from its host
galaxy. If these black-hole merger kicks are significant for black holes merging in the centers
of galaxies, the number of black holes with 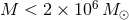 may therefore be significantly
depleted [147].
may therefore be significantly
depleted [147].
EMRI waveforms are complex, since the orbits are expected to be both eccentric and inclined with
respect to the equatorial plane of the central black hole. Various algorithms have been suggested for EMRI
detection, including hierarchical matched filtering starting with short data segments [193], time-frequency
analysis [467 , 200
, 200 , 194
, 194 ], searches with phenomenological templates [464], and Markov Chain
Monte Carlo searches [429, 35
], searches with phenomenological templates [464], and Markov Chain
Monte Carlo searches [429, 35 , 130]. Indeed, EMRIs have proven to be the most difficult source
to detect in the Mock LISA Data Challenges, but in the third challenge round two groups
correctly identified the three EMRIs with the highest-mass central black holes [39]. Several
challenging aspects remain unsolved, such as dealing with source confusion and detecting the EMRIs
with lower-mass central black holes, which are expected to dominate the data; recent progress
encourages confidence that these problems will be solved by the time a space-based detector is
launched.
, 130]. Indeed, EMRIs have proven to be the most difficult source
to detect in the Mock LISA Data Challenges, but in the third challenge round two groups
correctly identified the three EMRIs with the highest-mass central black holes [39]. Several
challenging aspects remain unsolved, such as dealing with source confusion and detecting the EMRIs
with lower-mass central black holes, which are expected to dominate the data; recent progress
encourages confidence that these problems will be solved by the time a space-based detector is
launched.
Assuming that a matched-filtering SNR of 30 is required for EMRI detection, and using the
previously-quoted EMRI rates per black hole [235], it was estimated that LISA could see several hundred
events [192] out to a redshift 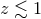 . The intrinsic rate uncertainties mean that the actual
number could be anywhere from a few tens to a few thousands. Most of the events will be
black-hole inspirals into MBHs of mass
. The intrinsic rate uncertainties mean that the actual
number could be anywhere from a few tens to a few thousands. Most of the events will be
black-hole inspirals into MBHs of mass 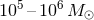 . The estimate for eLISA is a few tens of
events [197, 20, 21], although this is based on a lower SNR detection threshold of 20. (The
lower threshold is justified by the results of the Mock LISA Data Challenges, which successfully
demonstrated detection of EMRI events with SNR of
. The estimate for eLISA is a few tens of
events [197, 20, 21], although this is based on a lower SNR detection threshold of 20. (The
lower threshold is justified by the results of the Mock LISA Data Challenges, which successfully
demonstrated detection of EMRI events with SNR of 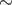 15, albeit with less-than-realistic signal
confusion.)
15, albeit with less-than-realistic signal
confusion.)
Astrophysics using EMRIs.
EMRI observations will tell us about the properties of MBHs at low redshift and about the physics of nuclear stellar clusters. Each EMRI observation can determine many of the parameters of the system, including the mass and spin of the central black hole, to accuracies of a fraction of a percent [46 , 238]. With as few as ten EMRI observations, LISA would be able to constrain the slope of the
MBH mass function at low redshift to
, 238]. With as few as ten EMRI observations, LISA would be able to constrain the slope of the
MBH mass function at low redshift to 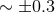 , which is the level of the current observational
uncertainty [199]. eLISA would have the same capability given the same number of detections. If LISA
observed as many EMRIs as current estimates predict, the constraints on the slope would improve by an
order of magnitude, although the corresponding improvement for eLISA would be only a factor of two.
EMRIs will also provide information on the distribution of black-hole spins. The masses of the compact
objects observed in EMRIs will provide information about mass-segregation processes, while the
overall number of events encodes information about the complicated physics that determines
the event rate. Finally, the eccentricities and inclinations of the observed EMRIs will provide
information on the relative efficiencies of the various channels through which EMRIs could be
formed [18
, which is the level of the current observational
uncertainty [199]. eLISA would have the same capability given the same number of detections. If LISA
observed as many EMRIs as current estimates predict, the constraints on the slope would improve by an
order of magnitude, although the corresponding improvement for eLISA would be only a factor of two.
EMRIs will also provide information on the distribution of black-hole spins. The masses of the compact
objects observed in EMRIs will provide information about mass-segregation processes, while the
overall number of events encodes information about the complicated physics that determines
the event rate. Finally, the eccentricities and inclinations of the observed EMRIs will provide
information on the relative efficiencies of the various channels through which EMRIs could be
formed [18 , 16
, 16 ].
].
Testing relativity using EMRIs.
EMRIs are well suited for testing relativity for several reasons: the systems are expected to be clean since the small object is likely to be a black hole with no internal structure, and the influence of any gaseous material in the spacetime is expected to be negligible (but see Section 6); the waveforms can be computed very accurately using black-hole perturbation theory [362 , 48],
allowing very small deviations from the model to be identified; the inspiral is slow, meaning that
many waveform cycles are generated in the strong-field regime, and can be used to test the
spacetime structure [179]; and the waveform structure is complex, due to the expected eccentricity
and inclination of the orbits, and to the intrinsic richness of dynamics in Kerr (or Kerr-like)
spacetimes [18
, 48],
allowing very small deviations from the model to be identified; the inspiral is slow, meaning that
many waveform cycles are generated in the strong-field regime, and can be used to test the
spacetime structure [179]; and the waveform structure is complex, due to the expected eccentricity
and inclination of the orbits, and to the intrinsic richness of dynamics in Kerr (or Kerr-like)
spacetimes [18 , 154
, 154 ].
The primary fundamental-physics application of EMRI observations is probing black-hole structure,
since the EMRI waveforms encode a map of the spacetime geometry close to the central black hole. This
can be used to test whether the central object is indeed described by the Kerr metric, as we expect, or is
some other kind of object. A discrepancy could indicate an astrophysical perturbation in the system, a
violation of the cosmic-censorship hypothesis [352] or of the energy conditions of GR, or even evidence for
the existence of an exotic massive compact object such as a boson star. All of this will be discussed in detail
in Section 6. EMRIs can also be used for tests of GW polarization (Section 5.1.1), and to
constrain alternative theories of gravity through detection of modifications to the inspiral rate (see
Section 5.1.3).
].
The primary fundamental-physics application of EMRI observations is probing black-hole structure,
since the EMRI waveforms encode a map of the spacetime geometry close to the central black hole. This
can be used to test whether the central object is indeed described by the Kerr metric, as we expect, or is
some other kind of object. A discrepancy could indicate an astrophysical perturbation in the system, a
violation of the cosmic-censorship hypothesis [352] or of the energy conditions of GR, or even evidence for
the existence of an exotic massive compact object such as a boson star. All of this will be discussed in detail
in Section 6. EMRIs can also be used for tests of GW polarization (Section 5.1.1), and to
constrain alternative theories of gravity through detection of modifications to the inspiral rate (see
Section 5.1.3).
4.3 Galactic binaries
The most numerous source class in the low-frequency GW band are the ultra-compact binaries of two
stellar-mass compact objects: white dwarfs, neutron stars, or black holes. Most of these will be
in the Milky Way. Numerical population synthesis [330, 328] and theoretical studies of the
highly-evolved stellar population in the galaxy [245, 246] predict that there will be 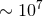 ultra-compact binaries generating GWs in the mHz band visible to space-based detectors like LISA or
eLISA.
ultra-compact binaries generating GWs in the mHz band visible to space-based detectors like LISA or
eLISA.
Ultra-compact binaries as low-frequency GW sources.
For an instrument with sensitivity similar to the “classic” LISA design, the complete population of compact galactic binaries would separate into two distinct observational classes: confused binaries and resolvable binaries. Confused binaries are those with orbital frequencies that are close enough to other binaries in the population that they cannot be readily distinguished from one another in the data [63, 231, 131 ]; their signals are
overlapping and cannot be disentangled from one another, unless one source happens to be
much closer and stronger than the others. For LISA, the confused binaries make up a stochastic
foreground signal from the galaxy, which would be present in the data for all frequencies below the
confusion limit
]; their signals are
overlapping and cannot be disentangled from one another, unless one source happens to be
much closer and stronger than the others. For LISA, the confused binaries make up a stochastic
foreground signal from the galaxy, which would be present in the data for all frequencies below the
confusion limit 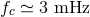 . Various attempts have been made to estimate the spectrum of
this foreground [329
. Various attempts have been made to estimate the spectrum of
this foreground [329 , 439, 162
, 439, 162 , 161, 386, 335], but the final profile would also depend on
details of the algorithms used to analyze the LISA data [278, 131, 431, 136, 90, 290]. For an
instrument with a sensitivity comparable to eLISA, which is approximately a factor of two worse in
the relevant range, this confusion foreground would be much less prominent, and perhaps not
detectable [20, 21].
Resolvable binaries are found both above and below the
, 161, 386, 335], but the final profile would also depend on
details of the algorithms used to analyze the LISA data [278, 131, 431, 136, 90, 290]. For an
instrument with a sensitivity comparable to eLISA, which is approximately a factor of two worse in
the relevant range, this confusion foreground would be much less prominent, and perhaps not
detectable [20, 21].
Resolvable binaries are found both above and below the 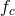 confusion limit. Sources below
confusion limit. Sources below 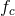 are
binaries that happen to be nearby, and so dominate the emission near their central frequency. For sources
above
are
binaries that happen to be nearby, and so dominate the emission near their central frequency. For sources
above 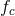 , the characteristic orbital frequencies are separated by a few frequency bin widths
, the characteristic orbital frequencies are separated by a few frequency bin widths
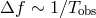 , where
, where 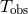 is the length of observation. An instrument like LISA would be expected to
resolve as many as twenty thousand individual binaries [329, 335], while for eLISA the number is expected
to be a few thousand [20, 21, 335].
is the length of observation. An instrument like LISA would be expected to
resolve as many as twenty thousand individual binaries [329, 335], while for eLISA the number is expected
to be a few thousand [20, 21, 335].
Among the resolvable binaries there are several verification binaries (see [430]; for a current list see [327]). These are binaries that are expected to be resolvable in the LISA/eLISA data, but have already been detected and characterized using electromagnetic telescopes. These known binaries will provide early verification that the mission is operating correctly at the nominal sensitivity.
Astrophysics using ultra-compact binaries.
The large number of compact binaries detectable throughout the entire galaxy makes these sources an exceptional resource to address questions about the stellar evolutionary history of the galaxy and the astrophysical dynamics of strong-field interactions in compact systems. If the confusion foreground is observed, it will be modulated by detector motion, a fact that can be used to estimate the multipole moments of the spatial distribution of binaries in the galaxy [407, 162]. A detector like LISA might also be able to detect the extra-galactic population of ultra-compact binaries, providing a valuable probe of its characteristics [171, 127, 129]. However, an extra-galactic population is unlikely to be observed by eLISA. Detections of galactic binaries will be dominated by double–white-dwarf binaries, which are scarce in the galaxy, so (e)LISA will be in a unique position to illuminate their physics. This will shed light on their formation and evolution, including the poorly-understood phase of common-envelope evolution. This is the phase during the evolution of a binary that follows unstable mass transfer. In the frame that co-rotates with the binary, the region of space around a star where orbiting material is bound to that star is known as the Roche lobe. As the orbit of a binary shrinks during inspiral, the Roche lobes also shrink, and one of them can eventually become smaller than the corresponding star. At that point the outer layers of the star are no longer gravitationally bound to the star so they are lost, and this mass is transferred to the other star in the binary. The process can be stable if, when the material is transferred, the orbital radius increases, the Roche lobe increases, and the star therefore no longer overflows the Roche lobe. Typically as the star loses mass it also becomes bigger, so stable mass transfer requires the Roche lobe to increase in size more quickly than the star. Mass transfer can also occur unstably if, when the material is lost, the orbit shrinks, leading to further shrinking of the Roche lobe and more mass transfer (or if the rate at which the star increases in size due to mass loss is faster than the rate at which the Roche lobe increases). After an unstable mass-transfer event, the companion star and the core of the donor star will end up as a binary contained entirely within the material that was transferred from the donor – the “common envelope.” Subsequently, energy is transferred from the orbit to the envelope, leading to shrinking of the orbit until the objects merge or, in the case of interest for ultra-compact binaries, the envelope has been completely expelled. The physics of this phase of the evolution is very poorly understood, but it is normally characterized in terms of the amount of energy transferred between the binary and the envelope. The distribution of orbital periods and masses for ultra-compact galactic binaries will encode important clues about this process.(e)LISA is also expected to observe interacting binaries where mass transfer is ongoing. GW observations can shed light on the fraction of mass-transferring systems; these systems may allow coincident electromagnetic observations, making them even more precise probes of astrophysical processes such as mass transfer and tidal coupling [403, 475, 404, 474].
Testing relativity using ultra-compact binaries.
Resolvable compact binaries offer a good laboratory for testing GR because their waveforms and evolution are well understood and easily described using model templates, and because a reasonable fraction of them will be visible both to (e)LISA and to electromagnetic telescopes. Ultra-compact binaries will provide constraints on massive-graviton theories, either by comparison of GW-propagation delays to the observable electromagnetic signals from the binary, or by the dispersion of the GW as the binary slowly evolves. This is discussed in Section 5.1.2. The ultra-compact binaries also provide laboratories for testing waveform evolution, and for GW polarization and dispersion studies, precisely because they evolve slowly and are easily described by post-Newtonian analysis. This is discussed in Sections 5.1.1 and 5.2.

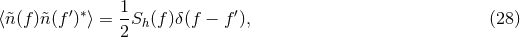
![[ ] ∫ ∞ h&tidle;∗1&tidle;h2 + &tidle;h1&tidle;h∗2 (h1|h2) = 2 ------------ df. (29 ) 0 Sh(f)](article221x.gif)
![[ 1 ] p(n = n0) ∝ exp − --(n0|n0) . (30 ) 2](article223x.gif)