List of Footnotes
| 1 | Some have argued that measurement of cosmological dark energy effects may be explained by long-range modifications of Einstein’s equations. While space-based GW observations may potentially help to refine redshift-distance measurements, we generally take the possibility of cosmological scale GR alternatives as falling outside the scope of this review. | |
| 2 | In this context, laser ranging constrains the Nordtvedt effect (the dependence of free fall for massive bodies on
gravitational self-energy), which is also a violation of SEP. The 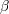 constraint given in [476] assumes the Cassini result [81 constraint given in [476] assumes the Cassini result [81 ] for ] for
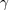 . . |
|
| 3 | In field theory, topological currents are those whose conservation follows not from the equations of motion, but from their very geometric construction. | |
| 4 | Because 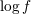 varies weakly in the relevant frequency ranges, it is considered constant in [28], and the varies weakly in the relevant frequency ranges, it is considered constant in [28], and the 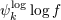 combinations are absorbed in the
combinations are absorbed in the 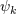 . . |
|
| 5 | The term bothrodesy was coined by Sterl Phinney in 2001, arising from the use of the Greek word 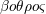 (meaning
“sacrificial pit”) to describe black holes. In modern Greek, (meaning
“sacrificial pit”) to describe black holes. In modern Greek, 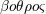 has come to mean “sewage pit”, so holiodesy is a suitable
replacement that was first suggested by Marc Favata [125 has come to mean “sewage pit”, so holiodesy is a suitable
replacement that was first suggested by Marc Favata [125 ]. ]. |
|
| 6 | Bondi–Hoyle–Lyttleton accretion is the process that occurs when an object is moving through a stationary distribution of material. The material is gravitationally focused behind the object and counter-rotating streams collide, which dissipates their angular momentum. The material then falls onto the back side of the object [92, 91]. | |
| 7 | The Petrov type of a metric describes the algebraic properties of the Weyl tensor, the vacuum part of the Riemann tensor. The Petrov classification is based on the multiplicities of the null eigenvectors (principal null directions) of the Weyl tensor. A metric of Petrov type D is algebraically special, having two repeated (double) principal null vectors, while metrics of type I have no algebraic symmetry, possessing four simple principal null directions. | |
| 8 | “Peeling” refers to the fact that different parts of the metric (specifically the Weyl tensor) fall off at different rates with distance. The dominant monopole component is effectively the mass of the central object, while the next-order component, the current dipole, is effectively the spin or angular momentum of the central black hole. The “peeling” constraints imposed in [458] ensured that the mass and spin were not changed by the perturbation. Such changes are already contained within the Kerr metric family and do not represent modifications to GR. | |
| 9 | The difference between the “adiabatic” and “self-consistent” evolutions is in the treatment of the past history of the particle, which is what determines the self-force. In the “adiabatic” approximation the past history is assumed to be geodesic, so the self-force is computed for objects on geodesics and then the final trajectory is evolved through a sequence of “osculating” geodesics using this geodesic self-force. The “self-consistent” approach uses the actual trajectory to describe the past history of the particle. The two approaches differ at second order in the mass ratio, which is the same order as other corrections that have been ignored in both approaches. |

