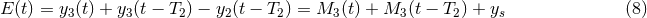7 Improving Doppler Tracking Sensitivity
What would be required to improve broadband12 burst sensitivity ten-fold, to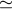 2 × 10–16 (thus, in a 40 day observation, have sensitivity to periodic
waves of
2 × 10–16 (thus, in a 40 day observation, have sensitivity to periodic
waves of 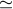 10–17)? Assuming that there is not some unexpected systematic effect entering between
10–15 and 10–16 and that the noises are independent (thus variances add and each component
must be brought to
10–17)? Assuming that there is not some unexpected systematic effect entering between
10–15 and 10–16 and that the noises are independent (thus variances add and each component
must be brought to 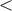 10–16), Table 4 shows the required improvements in the principal
subsystems.
10–16), Table 4 shows the required improvements in the principal
subsystems.
| Noise source |
Comment ( |
Required |
|
|
improvement | |
| Frequency standard |
currently FTS + distribution
|
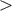 8X 8X |
| Ground electronics |
currently |
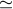 2X 2X |
| Tropospheric scintillation |
currently |
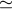 10X 10X |
| Plasma scintillation |
Cassini-class radio system probably
adequate for calibration to |
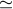 1X 1X |
| Spacecraft motion |
currently |
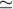 2X 2X |
| Antenna mechanical |
currently |
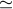 20X 20X |
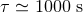 has
been demonstrated [62]. (If high-stability flyable frequency standards become available in the future, they
would allow simultaneous multiple one- and two-way Doppler measurements. These multiple observations
would give excellent diagnostics of many instrumental noises and provide further rejection of systematic
effects [125, 8, 117]). Better frequency standards would require better frequency distribution. Prototypes
for frequency distribution within the Atacama Large Millimeter Array achieve stability 10–16 or better for
time scales of about 1000 seconds [39].
has
been demonstrated [62]. (If high-stability flyable frequency standards become available in the future, they
would allow simultaneous multiple one- and two-way Doppler measurements. These multiple observations
would give excellent diagnostics of many instrumental noises and provide further rejection of systematic
effects [125, 8, 117]). Better frequency standards would require better frequency distribution. Prototypes
for frequency distribution within the Atacama Large Millimeter Array achieve stability 10–16 or better for
time scales of about 1000 seconds [39].
Improving ground electronics noise by 2X is probably possible by even more careful design. Reducing
tropospheric scintillation by 10X will require either an antenna at very high altitude, improvements in AMC
technology (e.g., exactly coincident beams, better water vapor radiometry technology), or perhaps an
interesting idea (suggested independently by Estabrook [48] and Hellings [59]) whereby a second ground
station (listen-only, at high altitude) could be employed to synthesize a Doppler observable which has the
(presumably much lower) tropospheric phase scintillation noise of the higher-altitude receive-only station.
Plasma scintillation correction technology is already adequate to reach an Allan deviation of 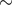 10–16.
Cassini spacecraft unmodeled motion was measured to be within a factor of about 2 of 10–16 (see
Section 4.8); it is not clear what actually limited the Cassini motion measurement so additional
analysis/design might be required to assure that this component entered at the 10–16 level or
lower.
10–16.
Cassini spacecraft unmodeled motion was measured to be within a factor of about 2 of 10–16 (see
Section 4.8); it is not clear what actually limited the Cassini motion measurement so additional
analysis/design might be required to assure that this component entered at the 10–16 level or
lower.
The largest required improvement is in antenna mechanical noise. It is impractical to build a large, steel,
earth-based, moving structure (such as a 34-m antenna) which has intrinsic 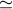 10–16 mechanical stability;
performance at this level will probably require a separate calibration/removal of mechanical
noise. One suggestion is to exploit the differing transfer function of antenna mechanical noise to
two- and three-way observations [15*]. Suppose that a stiffer (that is, smaller mechanical noise)
ancillary antenna is co-located with the two-way tracking antenna. The ancillary antenna takes
data in the “listen-only” (three-way) mode. The desired Doppler signal, ys, and mechanical
noises of the two antennas enter the time series of fractional Doppler fluctuation according
to
10–16 mechanical stability;
performance at this level will probably require a separate calibration/removal of mechanical
noise. One suggestion is to exploit the differing transfer function of antenna mechanical noise to
two- and three-way observations [15*]. Suppose that a stiffer (that is, smaller mechanical noise)
ancillary antenna is co-located with the two-way tracking antenna. The ancillary antenna takes
data in the “listen-only” (three-way) mode. The desired Doppler signal, ys, and mechanical
noises of the two antennas enter the time series of fractional Doppler fluctuation according
to
as if the ancillary antenna were both transmitting and receiving. If the ancillary antenna is sufficiently stiff (i.e., if the magnitude of M3 is small compared with the magnitude of M2) then mechanical noise in the observation can in principle be reduced substantially.
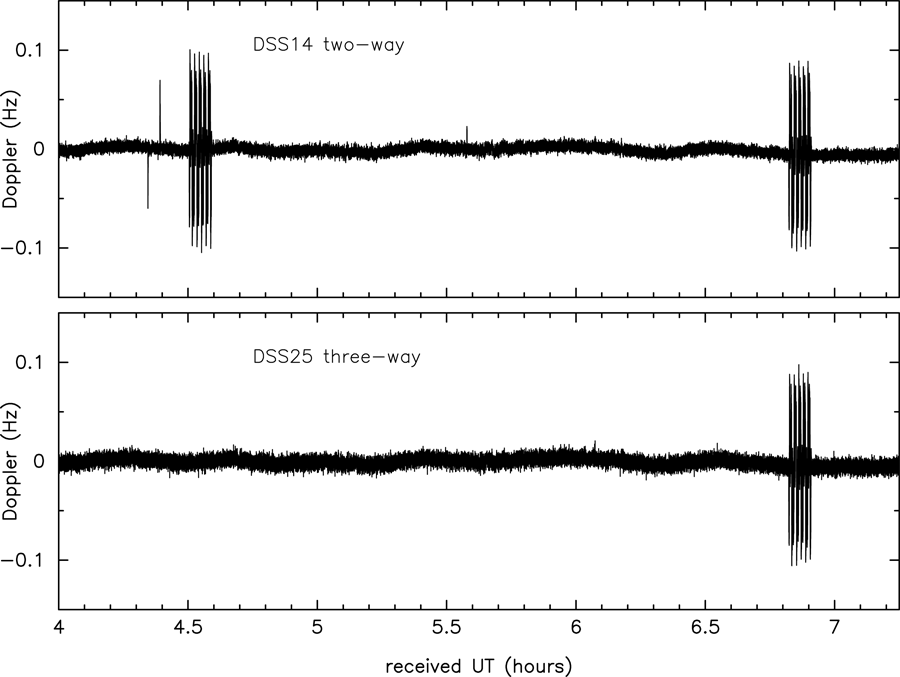
This idea was tested during an otherwise-routine Cassini observation [15*]. The Cassini spacecraft was tracked in the conventional two-way mode using NASA’s DSS14 70-m station while simultaneous three-way data were taken at a nearby antenna (DSS 25). During the track DSS 14’s subreflector was deliberately articulated to introduce a large, artificial “antenna mechanical variation” (the signal path within the antenna was described in Section 4). Figure 25* shows the two- and three-way Doppler time series during the test. The upper panel shows the “two-pulse” signature of antenna mechanical variation in the two-way data (Eq. (6*) and Figure 7*). The lower panel shows the effect of the deliberate subreflector motion in the three-way Doppler [Eq. (7*)].
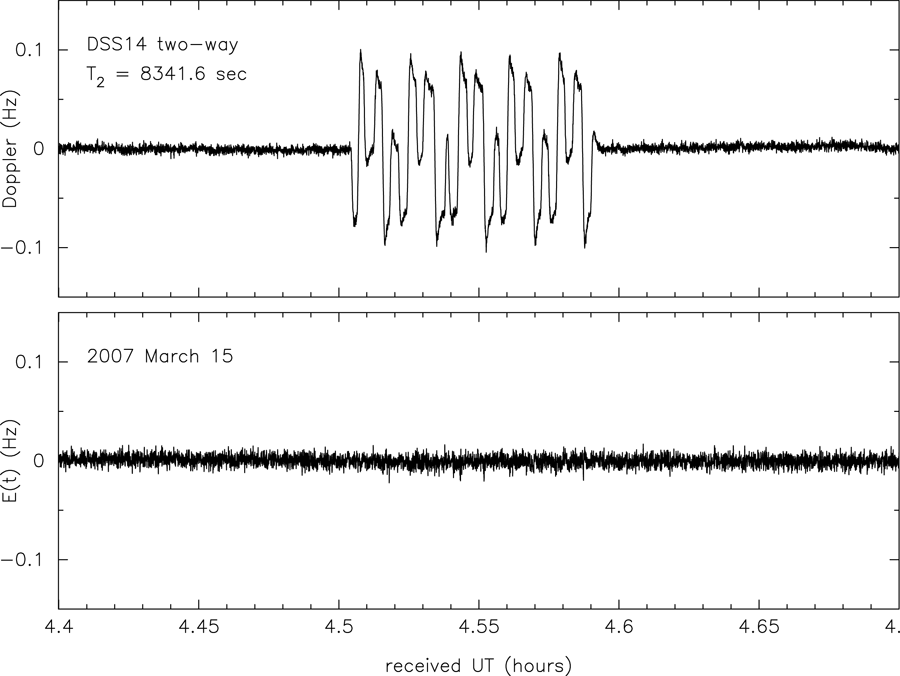
Figure 26* shows a blowup of the time series of the two-way Doppler during the subreflector motion event (upper panel) and the data combination E(t) formed using the two- and three-way data. The two-way mechanical variability cancels to the level of other noises.
Suitably stiff antennas (i.e., antennas with mechanical stability at least an order of magnitude better
than that of DSS 25) have been built for radio astronomy applications [103]. These or comparable antennas
could be used to reduce the antenna mechanical noise in Doppler gravitational wave tracks to 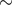 10–16 or
lower for
10–16 or
lower for 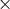 = 1000 s. Of course one would not use this technique except in situations where the antenna
mechanical noise dominates. Some considerations for a practical implementation of this method are
discussed in [14].
= 1000 s. Of course one would not use this technique except in situations where the antenna
mechanical noise dominates. Some considerations for a practical implementation of this method are
discussed in [14].
There is no currently-planned mission that requires Doppler stability at the 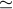 10–16 level. Indeed
unless such stability can be achieved inexpensively that level of Doppler performance might have to be
justified by a mission dedicated to precision radio science. As outlined above, however,
10–16 level. Indeed
unless such stability can be achieved inexpensively that level of Doppler performance might have to be
justified by a mission dedicated to precision radio science. As outlined above, however, 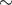 10–16 burst
sensitivity may be possible with extensions of current technologies. To do several orders of magnitude better
than 10–16, however – e.g., to achieve sensitivity adequate to detect the very weak GWs from known
Galactic binaries – would almost certainly require a different utilization of electromagnetic tracking [24*],
discussed briefly in Section 8.
10–16 burst
sensitivity may be possible with extensions of current technologies. To do several orders of magnitude better
than 10–16, however – e.g., to achieve sensitivity adequate to detect the very weak GWs from known
Galactic binaries – would almost certainly require a different utilization of electromagnetic tracking [24*],
discussed briefly in Section 8.

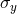 at
at 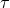 = 1000 s)
= 1000 s)  8
8 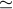 2
2 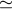 10
10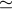 10
10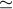 2
2  2
2