1 Introduction
Radio communications systems on deep space probes are used for both command and control of the spacecraft (via transmissions from the earth to the spacecraft, the uplink) and for returning telemetry to the ground (via transmission from the spacecraft to the earth, the downlink). These communications systems typically serve two additional purposes: navigation (use of radio ranging and earth-spacecraft Doppler to determine the position and velocity of the probe) and radio science (use of measured radiowave properties – amplitude, frequency, polarization, etc. – explicitly for mission science). Radio science can address several scientific topics including estimation of planetary masses and mass distributions, measurements of planetary ionospheres/atmospheres/rings, studies of planetary shapes and surfaces, observations of the solar wind, and tests of relativistic gravity. This article describes a radio science application: the use of precision Doppler tracking of deep space probes as a detector
of low-frequency1
gravitational waves (GWs). Precision Doppler experiments were pioneered by Vessot, whose
GP-A suborbital experiment measured the general relativistic redshift in the earth’s static
gravitational field [126*]. In the deep space GW observations discussed here, the earth and a distant
spacecraft are free test masses with the ground-based Doppler tracking system continuously
measuring the earth-spacecraft fractional velocity (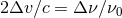 , with
, with  being the
Doppler shift and
being the
Doppler shift and 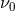 being the radio link’s carrier frequency). A gravitational wave with
strain amplitude h causes perturbations of order h in
being the radio link’s carrier frequency). A gravitational wave with
strain amplitude h causes perturbations of order h in 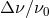 . Unlike other GW detectors, the
. Unlike other GW detectors, the
 1 – 10 AU earth-spacecraft separation makes the detector large compared with millihertz-band
gravitational wavelengths. Consequently times-of-flight of the GWs and radio waves through the
apparatus are important and impose characteristic signatures of GWs in the observed Doppler time
series.
1 – 10 AU earth-spacecraft separation makes the detector large compared with millihertz-band
gravitational wavelengths. Consequently times-of-flight of the GWs and radio waves through the
apparatus are important and impose characteristic signatures of GWs in the observed Doppler time
series.
The theory of the (two-way) Doppler GW detector was built up by generalizing the
response of so-called one-way Doppler measurements. In one-way tracking, each of two test
masses has its own frequency standard. Equipment on one test mass transmits a wave
referenced to its frequency standard and a receiver on the other mass estimates the Doppler
shift by comparing the frequency of the wave it receives with the frequency of its local
standard.2
In 1970, Kaufmann [74] calculated the fractional frequency fluctuation caused by GWs on one-way Doppler
in the context of proposed earth-based GW detectors using the Mössbauer effect. In 1971, Anderson [2]
commented on  100 s fluctuations in Mariner 6’s Doppler time series with the suggestion
that these might be related to resonant-bar events reported at roughly the same time. In 1974,
Davies [42] surveyed the prospects for GW detection with deep space probes. He carefully noted the
sensitivity advantages of Doppler (as contrasted with ranging), identified several competing error
sources, and presented the GW response for two-way Doppler in the special case of GWs incident
normal to the earth-spacecraft line. In 1975, Estabrook and Wahlquist [52*] derived the general
GW response for arbitrary angle-of-arrival and for a detector large compared with the GW
wavelength (see Section 3) and derived the spectral distribution of Doppler fluctuations due to an
isotropic GW background. With colleagues they considered signal and noise transfer functions, the
sensitivity of Doppler tracking to GWs (including the prospects for improving it), and the utility of
simultaneous tracking of several spacecraft [52*, 128*, 46*, 50, 47*]. In 1976, Thorne and Braginsky [109]
estimated event rates for low-frequency GW bursts and discussed the prospects for observing these
bursts with spacecraft Doppler tracking. The first systematic GW observations with deep-space
Doppler tracking were made in the 1980s; those observations – and technical developments in
the following two decades resulting in thousand-fold improved GW sensitivity – are discussed
below.
Update*
100 s fluctuations in Mariner 6’s Doppler time series with the suggestion
that these might be related to resonant-bar events reported at roughly the same time. In 1974,
Davies [42] surveyed the prospects for GW detection with deep space probes. He carefully noted the
sensitivity advantages of Doppler (as contrasted with ranging), identified several competing error
sources, and presented the GW response for two-way Doppler in the special case of GWs incident
normal to the earth-spacecraft line. In 1975, Estabrook and Wahlquist [52*] derived the general
GW response for arbitrary angle-of-arrival and for a detector large compared with the GW
wavelength (see Section 3) and derived the spectral distribution of Doppler fluctuations due to an
isotropic GW background. With colleagues they considered signal and noise transfer functions, the
sensitivity of Doppler tracking to GWs (including the prospects for improving it), and the utility of
simultaneous tracking of several spacecraft [52*, 128*, 46*, 50, 47*]. In 1976, Thorne and Braginsky [109]
estimated event rates for low-frequency GW bursts and discussed the prospects for observing these
bursts with spacecraft Doppler tracking. The first systematic GW observations with deep-space
Doppler tracking were made in the 1980s; those observations – and technical developments in
the following two decades resulting in thousand-fold improved GW sensitivity – are discussed
below.
Update*