3 Strong Need for Strong Gravity
The need for NR is almost as old as GR itself, but the real push to develop these tools came primarily from the necessity to understand conceptual issues such as the end-state of collapse and the two-body problem in GR as well as from astrophysics and GW astronomy. The breakthroughs in the last years have prompted a serious reflexion and examination of the multitude of problems and fields that stand to gain from NR tools and results, if extended to encompass general spacetimes. The following is a brief description of each of these topics. The range of fundamental issues for which accurate strong-gravity simulations are required will hopefully become clear.
3.1 Astrophysics
3.1.1 Gravitational wave astronomy
GWs are one of the most fascinating predictions of GR. First conceived by Einstein [294*, 296], it was unclear for a long time whether they were truly physical. Only in the 1960s were their existence and properties founded on a sound mathematical basis (see [450, 451] and references therein). In the same period, after the seminal work of Weber [770], the scientific community was starting a growing experimental effort to directly detect GWs. The first detectors were resonant antennas; their sensitivity was far too low to detect any signal (unless a nearby galactic supernova exploded when the detector was taking data), and they were eventually replaced by interferometric detectors. The first generation of such detectors (LIGO, Virgo, GEO600, TAMA) did not reveal any gravitational signal, but the second generation (Advanced LIGO/Virgo [517, 761]) should be operative by 2015 and is expected to make the first detection of GWs. In parallel, Pulsar Timing Arrays are promising to detect ultra-low frequency GWs [507], whereas the polarization of the cosmic microwave background can be used as a detector of GWs from an inflationary epoch in the very early universe [690, 369, 725, 659, 7]. In the subsequent years more sensitive detectors, such as the underground cryogenic interferometer KAGRA [462] (and, possibly, ET [299]) and possibly a space-based detector such as LISA/eLISA [302], will allow us to know the features of the signal in more detail, and then to use this information to learn about the physics of the emitting sources, and the nature of the gravitational interaction.Soon after the beginning of the experimental efforts to build a GW detector, it became clear that the detection of GWs emitted by astrophysical sources would open a new window of observational astronomy, in addition to the electromagnetic spectrum, neutrinos, cosmic rays, etc. The impact of such a detection would be similar to that of X-rays from astrophysical sources, i.e., the birth of a new branch of astronomy: “GW astronomy” [628, 370, 686]. In this new field, source modelling is crucial, since a theoretical understanding of the expected GW sources is needed to enhance the chances of detection and to extract the relevant physics. Indeed, template-matching techniques – frequently used in data analysis – can be helpful to extract the signal from the detector noise, but they require an a-priori knowledge of the waveforms [752].
A wide scientific community formed, with the aim to model the physical processes that are expected to produce a detectable GW signal, and to compute the emitted gravitational waveform (which depends on the unknown parameters of the source and of the emitting process). Together with the understanding of the two-body problem in GR, this effort was one of the main driving forces leading to the development of NR. Indeed, many promising GW sources can only be modeled by solving the fully nonlinear Einstein equations numerically.
Ground-based interferometers are (and are expected to be in the next decades) sensitive to signals with frequencies ranging from some tens of Hz to about one kHz. Space-based interferometers would be sensitive at much lower frequencies: from some mHz to about one tenth of Hz. GW astronomy, of course, is presently concerned with sources emitting GWs in these frequency bands.
Many astrophysical processes are potential sources for GW detectors. In the following, we shall briefly discuss only some of them, i.e., those that require NR simulations to be modeled: compact binary inspirals, and instabilities of rotating NSs. We shall not discuss supernova core collapse – one of the first GW sources that have been studied with NR, and one of the most problematic to model – since it will be discussed in Section 3.1.2.
Compact binary inspirals, i.e., the inspiral and merger of binary systems formed by BHs and/or NSs, are the most promising GW sources to be detected. Advanced LIGO/Virgo are expected to detect some tens of these sources per year [5]. While the inspiral phase of a compact binary system can be accurately modeled through PN approaches, and the final (“ringdown”) phase, when the BH resulting from the coalescence oscillates in its characteristic proper modes, can be accurately described through perturbative approaches, the intermediate merger phase can only be modeled by NR. This task has posed formidable theoretical and computational challenges to the scientific community.
The numerical simulation of the merger phase of a BH-BH binary coalescence, and the determination of the emitted gravitational waveform, had been an open problem for decades, until it was solved in 2005 [629*, 159*, 65*]. This challenge forced the gravitational community to reflect on deep issues and problems arising within Einstein’s theory, such as the role of singularities and horizons, and the possible ways to locally define energy and momentum.
BH-NS and NS-NS binary coalescences pose a different sort of problems than those posed by BH-BH coalescences. They are not a “clean” system such as purely vacuum BH spacetimes, characterized by the gravitational interaction only. An accurate numerical modeling involves various branches of physics (nuclear physics, neutrinos, electromagnetic fields), and requires the understanding of many different processes. Typically, NR simulations of BH-NS and NS-NS mergers make simplifying assumptions, both because taking into account all aspects at the same time would be too complicated, and because some of them are not fully understood. Currently, the behaviour of matter in the inner core of a NS is one of the challenges to be tackled. Indeed, nuclear physicists still do not understand which is the equation of state of matter at such extreme conditions of density and temperature (see, e.g., [501] and references therein). This uncertainty reflects our ignorance on the behaviour of the hadronic interactions in the non-perturbative regime. On the other hand, understanding the NS equation of state is considered one of the main outcomes expected from the detection of a GW signal emitted by NSs, for instance in compact binary coalescences [583, 640, 80, 738].
Neutron star oscillations are also a candidate GW source for ground-based interferometers. When
perturbed by an external or internal event, a NS can be set into non-radial damped oscillations, which are
associated to the emission of GWs. The characteristic frequencies of oscillation, the QNMs, are
characterized by their complex frequency 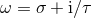 , where
, where 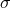 is the pulsation frequency, and
is the pulsation frequency, and 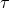 is
the damping time of the oscillation (for detailed discussions on the QNMs of NSs and BHs see
[487*, 580, 316*, 95*] and references therein).
is
the damping time of the oscillation (for detailed discussions on the QNMs of NSs and BHs see
[487*, 580, 316*, 95*] and references therein).
If a NS rotates, its oscillations can become unstable. In this case, the oscillation grows until the instability is suppressed by some damping mechanism or by nonlinear effects; this process can be associated to a large GW emission (see, e.g., [34] and references therein). These instabilities may explain the observed values of the NS rotation rates [101]. Their numerical modeling, however, is not an easy task. Perturbative approaches, which easily allow one to compute the QNMs of non-rotating NSs, become very involved in the presence of rotation. Therefore, the perturbation equations can only be solved with simplifying assumptions, which make the model less accurate. Presently, NR is the only way to model stationary, rapidly rotating NSs (see, e.g., [728*] and references therein), and it has recently been applied to model their oscillations [842].
3.1.2 Collapse in general relativity
Decades before any observation of supermassive compact objects, and long before BHs were understood, Chandrasekhar showed that the electron degeneracy pressure in very massive white dwarfs is not enough to prevent them from imploding [193]. Similar conclusions were reached later by Oppenheimer and Volkoff, for neutron degeneracy pressure in NSs [590]. We can use Landau’s original argument to understand these results [498, 499, 691]: consider a star of radius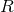 composed of
composed of 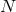 fermions, each of mass
fermions, each of mass 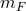 . The momentum of each
fermion is
. The momentum of each
fermion is 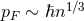 , with
, with 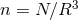 the number density of fermions. In the relativistic
regime, the Fermi energy per particle then reads
the number density of fermions. In the relativistic
regime, the Fermi energy per particle then reads 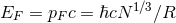 . The gravitational
energy per fermion is approximately
. The gravitational
energy per fermion is approximately 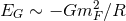 , and the star’s total energy is thus,
For small
, and the star’s total energy is thus,
For small 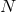 , the total energy is positive, and we can decrease it by increasing
, the total energy is positive, and we can decrease it by increasing 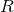 . At some point the
fermion becomes non-relativistic and
. At some point the
fermion becomes non-relativistic and 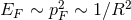 . In this regime, the gravitational binding energy
. In this regime, the gravitational binding energy
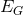 dominates over
dominates over 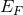 , the total energy is negative and tends to zero as
, the total energy is negative and tends to zero as 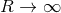 . Thus there is a
local minimum and the star is stable. However, for large
. Thus there is a
local minimum and the star is stable. However, for large 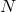 in the relativistic regime the total energy is
negative, and can be made even more negative by decreasing
in the relativistic regime the total energy is
negative, and can be made even more negative by decreasing 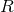 : it is energetically favoured for the star
to continually collapse! The threshold for stability occurs at a zero of the total energy, when
For neutrons, stars with masses above
: it is energetically favoured for the star
to continually collapse! The threshold for stability occurs at a zero of the total energy, when
For neutrons, stars with masses above 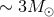 cannot attain equilibrium.
cannot attain equilibrium.
What is the fate of massive stars whose pressure cannot counter-balance gravity? Does the star’s material continually collapse to a single point, or is it possible that pressure or angular momentum become so important that the material bounces back? The answer to these questions would take several decades more, and was one of the main driving forces to develop solid numerical schemes to handle Einstein’s equations.
Other developments highlighted the importance of understanding gravitational collapse in GR. One was the advent of GW detectors. The strongest sources of GWs are compact and moving relativistically, and supernovae are seemingly ideal: they occur frequently and are extremely violent. Unfortunately, Birkhoff’s theorem implies that spherically symmetric sources do not radiate. Thus a careful, and much more complex analysis of collapse is required to understand these sources.
In parallel, BH physics was blooming. In the 1970s one key result was established: the uniqueness theorem, stating that – under general regularity assumptions – the only stationary, asymptotically flat, vacuum solution of Einstein’s field equations is the Kerr BH. Thus, if a horizon forms, the final stationary configuration is expected to be of the Kerr family. This important corollary of Einstein’s field equations calls for a dynamical picture of BH formation through collapse and an understanding of how the spacetime multipolar structure dynamically changes to adapt to the final Kerr solution as a BH forms.
3.1.3 Kicks
It has been known since the early 1960s that GWs emitted by accelerated particles do not only carry energy but also momentum away from the system on which thus is imparted a kick or recoil. This effect was first studied by Bonnor & Rotenberg [119] for the case of a system of oscillating particles, and has been identified by Peres [612] to be at leading order due to the interference of the mass quadrupole radiation with the mass octupole or flow quadrupole.From an astrophysical point of view, the most important processes generating such gravitational recoil are
the collapse of a stellar core to a compact object and the inspiral and merger of compact binaries.
Supermassive BHs with masses in the range of 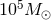 to
to 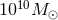 in particular are known to reside at
the centre of many galaxies and are likely to form inspiralling binary systems as a consequence of galaxy
mergers. Depending on the magnitude of the resulting velocities, kicks can in principle displace or eject BHs
from their hosts and therefore play an important role in the formation history of these supermassive
BHs.
in particular are known to reside at
the centre of many galaxies and are likely to form inspiralling binary systems as a consequence of galaxy
mergers. Depending on the magnitude of the resulting velocities, kicks can in principle displace or eject BHs
from their hosts and therefore play an important role in the formation history of these supermassive
BHs.
The first calculations of recoil velocities based on perturbative techniques have been applied to gravitational collapse scenarios by Bekenstein [84] and Moncrief [556]. The first analysis of GW momentum flux generated by binary systems was performed by Fitchett [322] in 1983 for two masses in Keplerian orbit. The following two decades saw various (semi-)analytic calculations for inspiraling compact binary systems using the particle approximation, post-Newtonian techniques and the close-limit approach (see Section 5 for a description of these techniques and main results). In conclusion of these studies, it appeared likely that the gravitational recoil from non-spinning binaries was unlikely to exceed a few hundred km/s. Precise estimates, however, are dependent on an accurate modeling of the highly nonlinear late inspiral and merger phase and therefore required NR simulations. Furthermore, the impact of spins on the resulting velocities remained essentially uncharted territory until the 2005 breakthroughs of NR made possible the numerical simulations of these systems. As it turned out, some of the most surprising and astrophysically influencial results obtained from NR concern precisely the question of the gravitational recoil of spinning BH binaries.
3.1.4 Astrophysics beyond Einstein gravity
Although GR is widely accepted as the standard theory of gravity and has survived all experimental and observational (weak field) scrutiny, there is convincing evidence that it is not the ultimate theory of gravity: since GR is incompatible with quantum field theory, it should be considered as the low energy limit of some, still elusive, more fundamental theory. In addition, GR itself breaks down at small length scales, since it predicts singularities. For large scales, on the other hand, cosmological observations show that our universe is filled with dark matter and dark energy, of as yet unknown nature.This suggests that the strong-field regime of gravity – which has barely been tested so far – could be
described by some modification or extension of GR. In the next few years both GW detectors [786, 826*]
and astrophysical observations [635*] will provide an unprecedented opportunity to probe the strong-field
regime of the gravitational interaction, characterized by large values of the gravitational field 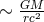 or of
the spacetime curvature
or of
the spacetime curvature 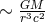 (it is a matter of debate which of the two parameters is the most
appropriate for characterizing the strong-field gravity regime [635, 826*]). However, our present theoretical
knowledge of strong-field astrophysical processes is based, in most cases, on the a-priori assumption that
GR is the correct theory of gravity. This sort of theoretical bias [825*] would strongly limit our possibility of
testing GR.
(it is a matter of debate which of the two parameters is the most
appropriate for characterizing the strong-field gravity regime [635, 826*]). However, our present theoretical
knowledge of strong-field astrophysical processes is based, in most cases, on the a-priori assumption that
GR is the correct theory of gravity. This sort of theoretical bias [825*] would strongly limit our possibility of
testing GR.
It is then of utmost importance to understand the behaviour of astrophysical processes in the strong gravity regime beyond the assumption that GR is the correct theory of gravity. The most powerful tool for this purpose is probably NR; indeed, although NR has been developed to solve Einstein’s equations (possibly coupled to other field equations), it can in principle be extended and modified, to model physical processes in alternative theories of gravity. In summary, NR can be applied to specific, well motivated theories of gravity. These theories should derive from – or at least be inspired by – some more fundamental theories or frameworks, such as for instance SMT [366, 624] (and, to some extent, Loop Quantum Gravity [657]). In addition, such theories should allow a well-posed initial-value formulation of the field equations. Various arguments suggest that the modifications to GR could involve [826] (i) additional degrees of freedom (scalar fields, vector fields); (ii) corrections to the action at higher order in the spacetime curvature; (iii) additional dimensions.
Scalar-tensor theories for example (see, e.g., [337, 783*] and references therein), are the most natural and simple generalizations of GR including additional degrees of freedom. In these theories, which include for instance Brans–Dicke gravity [128], the metric tensor is non-minimally coupled with one or more scalar fields. In the case of a single scalar field (which can be generalized to multi-scalar-tensor theories [242*]), the action can be written as
where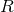 is the Ricci scalar associated to the metric
is the Ricci scalar associated to the metric 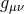 ,
, 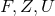 are arbitrary functions of the scalar
field
are arbitrary functions of the scalar
field 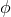 , and
, and 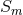 is the action describing the dynamics of the other fields (which we call “matter fields”,
is the action describing the dynamics of the other fields (which we call “matter fields”,
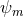 ). A more general formulation of scalar-tensor theories yielding second order equations of motion has
been proposed by Horndeski [435] (see also Ref. [260]).
). A more general formulation of scalar-tensor theories yielding second order equations of motion has
been proposed by Horndeski [435] (see also Ref. [260]).
Scalar-tensor theories can be obtained as low-energy limits of SMT [342]; this provides motivation for studying these theories on the grounds of fundamental physics. An additional motivation comes from the recently proposed “axiverse” scenario [49*, 50*], in which ultra-light axion fields (pseudo-scalar fields, behaving under many respects as scalar fields) arise from the dimensional reduction of SMT, and play a role in cosmological models.
Scalar-tensor theories are also appealing alternatives to GR because they predict new phenomena, which
are not allowed in GR. In these theories, the GW emission in compact binary coalescences has a dipolar
( ) component, which is absent in GR; if the scalar field has a (even if extremely small) mass,
superradiant instabilities occur [183, 604*, 794*], which can determine the formation of floating orbits in
extreme mass ratio inspirals [165*, 824*], and these orbits affect the emitted GW signal; last but not least,
under certain conditions isolated NSs can undergo a phase transition, acquiring a nontrivial
scalar-field profile (spontaneous scalarization [242*, 243]) while dynamically evolving NSs –
requiring full NR simulations to understand – may display a similar effect (dynamical scalarization
[73*, 596*]). A detection of one of these phenomena would be a smoking gun of scalar-tensor
gravity.
) component, which is absent in GR; if the scalar field has a (even if extremely small) mass,
superradiant instabilities occur [183, 604*, 794*], which can determine the formation of floating orbits in
extreme mass ratio inspirals [165*, 824*], and these orbits affect the emitted GW signal; last but not least,
under certain conditions isolated NSs can undergo a phase transition, acquiring a nontrivial
scalar-field profile (spontaneous scalarization [242*, 243]) while dynamically evolving NSs –
requiring full NR simulations to understand – may display a similar effect (dynamical scalarization
[73*, 596*]). A detection of one of these phenomena would be a smoking gun of scalar-tensor
gravity.
These theories, whose well-posedness has been proved [669, 670*], are a perfect arena for NR. Recovering some of the above smoking-gun effects is extremely challenging, as the required timescales are typically very large when compared to any other timescales in the problem.
Other examples for which NR can be instrumental include theories in which the Einstein–Hilbert action
is modified by including terms quadratic in the curvature (such as 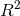 ,
, 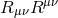 ,
, 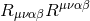 ,
,
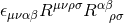 ), possibly coupled with scalar fields, or theories which explicitly break Lorentz
invariance. In particular, Einstein-Dilaton-Gauss–Bonnet gravity and Dynamical Chern–Simons
gravity [602*, 27*] can arise from SMT compactifications, and Dynamical Chern–Simons gravity also arises in
Loop Quantum Gravity; theories such as Einstein-Aether [456] and “Hořava–Lifshitz” gravity [433],
which break Lorentz invariance (while improving, for instance, renormalizability properties of GR), allow
the basic tenets of GR to be challenged and studied in depth.
), possibly coupled with scalar fields, or theories which explicitly break Lorentz
invariance. In particular, Einstein-Dilaton-Gauss–Bonnet gravity and Dynamical Chern–Simons
gravity [602*, 27*] can arise from SMT compactifications, and Dynamical Chern–Simons gravity also arises in
Loop Quantum Gravity; theories such as Einstein-Aether [456] and “Hořava–Lifshitz” gravity [433],
which break Lorentz invariance (while improving, for instance, renormalizability properties of GR), allow
the basic tenets of GR to be challenged and studied in depth.
3.2 Fundamental and mathematical issues
3.2.1 Cosmic censorship
Spacetime singularities signal the breakdown of the geometric description of the spacetime, and can be diagnosed by either the blow-up of observer-invariant quantities or by the impossibility to continue timelike or null geodesics past the singular point. For example, the Schwarzschild geometry has a curvature invariant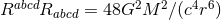 in Schwarzschild coordinates, which
diverges at
in Schwarzschild coordinates, which
diverges at 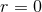 , where tidal forces are also infinite. Every timelike or null curve crossing the horizon
necessarily hits the origin in finite proper time or affine parameter and, therefore, the theory breaks down at
these points: it fails to predict the future development of an object that reaches the singular point.
Thus, the classical theory of GR, from which spacetimes with singularities are obtained, is
unable to describe these singular points and contains its own demise. Adding to this classical
breakdown, it is likely that quantum effects take over in regions where the curvature radius becomes
comparable to the scale of quantum processes, much in the same way as quantum electrodynamics
is necessary in regions where EM fields are large enough (as characterized by the invariant
, where tidal forces are also infinite. Every timelike or null curve crossing the horizon
necessarily hits the origin in finite proper time or affine parameter and, therefore, the theory breaks down at
these points: it fails to predict the future development of an object that reaches the singular point.
Thus, the classical theory of GR, from which spacetimes with singularities are obtained, is
unable to describe these singular points and contains its own demise. Adding to this classical
breakdown, it is likely that quantum effects take over in regions where the curvature radius becomes
comparable to the scale of quantum processes, much in the same way as quantum electrodynamics
is necessary in regions where EM fields are large enough (as characterized by the invariant
 ) that pair creation occurs. Thus, a quantum theory of gravity might be needed close to
singularities.
) that pair creation occurs. Thus, a quantum theory of gravity might be needed close to
singularities.
It seems therefore like a happy coincidence that the Schwarzschild singularity is cloaked by an event horizon, which effectively causally disconnects the region close to the singularity from outside observers. This coincidence introduces a miraculous cure to GR’s apparently fatal disease: one can continue using classical GR for all practical purposes, while being blissfully ignorant of the presumably complete theory that smoothens the singularity, as all those extra-GR effects do not disturb processes taking place outside the horizon.
Unfortunately, singularities are expected to be quite generic: in a remarkable set of works, Hawking and Penrose have proved that, under generic conditions and symmetries, collapse leads to singularities [608, 402, 408, 570]. Does this always occur, i.e., are such singularities always hidden to outside observers by event horizons? This is the content of Penrose’s “cosmic censorship conjecture”, one of the outstanding unsolved questions in gravity. Loosely speaking, the conjecture states that physically reasonable matter under generic initial conditions only forms singularities hidden behind horizons [767].
The cosmic-censorship conjecture and the possible existence of naked singularities in our universe has triggered interest in complex problems which can only be addressed by NR. This is a very active line of research, with problems ranging from the collapse of matter to the nonlinear stability of “black” objects.
3.2.2 Stability of black hole interiors
As discussed in Section 3.1.2, the known fermionic degeneracies are unable to prevent the gravitational collapse of a sufficiently massive object. Thus, if no other (presently unkown) physical effect can prevent it, according to GR, a BH forms. From the uniqueness theorems (cf. Section 4.1.1), this BH is described by the Kerr metric. Outside the event horizon, the Kerr family – a 2-parameter family described by mass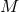 and
angular momentum
and
angular momentum 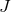 – varies smoothly with its parameters. But inside the event horizon a
puzzling feature occurs. The interior of the
– varies smoothly with its parameters. But inside the event horizon a
puzzling feature occurs. The interior of the 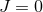 solution – the Schwarzschild geometry – is
qualitatively different from the
solution – the Schwarzschild geometry – is
qualitatively different from the 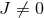 case. Indeed, inside the Schwarzschild event horizon a
point-like, spacelike singularity creates a boundary for spacetime. Inside the
case. Indeed, inside the Schwarzschild event horizon a
point-like, spacelike singularity creates a boundary for spacetime. Inside the 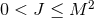 Kerr event horizon, by contrast, there is a ring-like, timelike singularity, beyond which another
asymptotically flat spacetime region, with
Kerr event horizon, by contrast, there is a ring-like, timelike singularity, beyond which another
asymptotically flat spacetime region, with  in Boyer–Lindquist coordinates, may be reached by
causal trajectories. The puzzling feature is then the following: according to these exact solutions,
the interior of a Schwarzschild BH, when it absorbes an infinitesimal particle with angular
momentum, must drastically change, in particular by creating another asymptotically flat region of
spacetime.
in Boyer–Lindquist coordinates, may be reached by
causal trajectories. The puzzling feature is then the following: according to these exact solutions,
the interior of a Schwarzschild BH, when it absorbes an infinitesimal particle with angular
momentum, must drastically change, in particular by creating another asymptotically flat region of
spacetime.
This latter conclusion is quite unreasonable. It is more reasonable to expect that the internal structure of an eternal Kerr BH must be very different from that of a Kerr BH originating from gravitational collapse. Indeed, there are arguments, of both physical [609] and mathematical nature [198], indicating that the Cauchy horizon (i.e., inner horizon) of the eternal charged or rotating hole is unstable against small (linear) perturbations, and therefore against the accretion of any material. The natural question is then, what is the endpoint of the instability?
As a toy model for the more challenging Kerr case, the aforementioned question was considered in the context of spherical perturbations of the RN BH by Poisson and Israel. In their seminal work, the phenomenon of mass inflation was unveiled [621*, 622*]: if ingoing and outgoing streams of matter are simultaneously present near the inner horizon, then relativistic counter-streaming2 between those streams leads to exponential growth of gauge-invariant quantities such as the interior (Misner–Sharp [552]) mass, the center-of-mass energy density, or curvature scalar invariants. Since this effect is causally disconnected from any external observers, the mass of the BH measured by an outside observer remains unchanged by the mass inflation going on in the interior. But this inflation phenomenon causes the spacetime curvature to grow to Planckian values in the neighbourhood of the Cauchy horizon. The precise nature of this evolution for the Kerr case is still under study. For the simpler RN case, it has been argued by Dafermos, using analytical methods, that the singularity that forms is not of space-like nature [234]. Fully nonlinear numerical simulations will certainly be important for understanding this process.
3.2.3 Most luminous events in the universe
The most advanced laser units on the planet can output luminosities as high as [301], while at
[301], while at  the Tsar Bomba remains the most powerful
artificial explosion ever [732]. These numbers pale in comparison with strongly dynamical astrophysical
events: a
the Tsar Bomba remains the most powerful
artificial explosion ever [732]. These numbers pale in comparison with strongly dynamical astrophysical
events: a 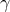 -ray burst, for instance, reaches luminosities of approximately
-ray burst, for instance, reaches luminosities of approximately 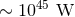 . A simple order of
magnitude estimate can be done to estimate the total luminosity of the universe in the EM spectrum, by
counting the total number of stars, roughly
. A simple order of
magnitude estimate can be done to estimate the total luminosity of the universe in the EM spectrum, by
counting the total number of stars, roughly 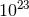 [443]. If all of them have a luminosity equal to
our Sun, we get a total luminosity of approximately
[443]. If all of them have a luminosity equal to
our Sun, we get a total luminosity of approximately 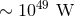 , a number which can also be
arrived at through more careful considerations [781]. Can one possibly surpass this astronomical
number?
, a number which can also be
arrived at through more careful considerations [781]. Can one possibly surpass this astronomical
number?
In four spacetime dimensions, there is only one constant with dimensions of energy per second
that can be built out of the classical universal constants. This is the Planck luminosity 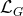 ,
,
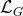 should control gravity-dominated dynamical processes; as such it is no wonder
that these events release huge luminosities. Take the gravitational collapse of a compact star
with mass
should control gravity-dominated dynamical processes; as such it is no wonder
that these events release huge luminosities. Take the gravitational collapse of a compact star
with mass 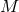 and radius
and radius 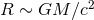 . During a collapse time of the order of the infall
time,
. During a collapse time of the order of the infall
time, 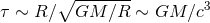 , the star can release an energy of up to
, the star can release an energy of up to 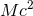 . The process
can therefore yield a power as large as
. The process
can therefore yield a power as large as 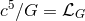 . It was conjectured by Thorne [751] that
the Planck luminosity is in fact an upper limit for the luminosity of any process in the
universe.3
The conjecture was put on a somewhat firmer footing by Gibbons who has shown that there is an upper
limit to the tension of
. It was conjectured by Thorne [751] that
the Planck luminosity is in fact an upper limit for the luminosity of any process in the
universe.3
The conjecture was put on a somewhat firmer footing by Gibbons who has shown that there is an upper
limit to the tension of 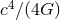 , implying a limit in the luminosity of
, implying a limit in the luminosity of 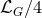 [349].
[349].
Are such luminosities ever attained in practice, is there any process that can reach the Planck luminosity and outshine the entire universe? The answer to this issue requires once again a peek at gravity in strongly dynamical collisions with full control of strong-field regions. It turns out that high energy collisions of BHs do come close to saturating the bound (6*) and that in general colliding BH binaries are more luminous than the entire universe in the EM spectrum [719*, 720*, 717, 716*].
3.2.4 Higher dimensions
Higher-dimensional spacetimes are a natural framework for mathematicians and have been of general interest in physics, most notably as a tool to unify gravity with the other fundamental interactions. The quest for a unified theory of all known fundamental interactions is old, and seems hopeless in four-dimensional arenas. In a daring proposal however, Kaluza and Klein, already in 1921 and 1926 showed that such a programme might be attainable if one is willing to accept higher-dimensional theories as part of the fundamental picture [463*, 476] (for a historical view, see [283*]).Consider first for simplicity the 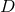 -dimensional Klein–Gordon equation
-dimensional Klein–Gordon equation 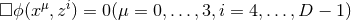 ,
where the
,
where the 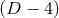 extra dimensions are compact of size
extra dimensions are compact of size 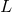 . Fourier decompose in
. Fourier decompose in 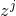 , i.e,
, i.e,
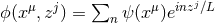 , to get
, to get 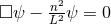 , where here
, where here  is the four-dimensional
d’Alembertian operator. As a consequence,
is the four-dimensional
d’Alembertian operator. As a consequence,
i) the fundamental, homogenous mode  is a massless four-dimensional field obeying the same
Klein–Gordon equation, whereas
is a massless four-dimensional field obeying the same
Klein–Gordon equation, whereas
ii) even though we started with a higher-dimensional massless theory, we end up with a tower of massive
modes described by the four-dimensional massive Klein–Gordon equation, with mass terms proportional to
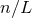 . One important, generic conclusion is that the higher-dimensional (fundamental) theory imparts
mass terms as imprints of the extra dimensions. As such, the effects of extra dimensions are in principle
testable. However, for very small
. One important, generic conclusion is that the higher-dimensional (fundamental) theory imparts
mass terms as imprints of the extra dimensions. As such, the effects of extra dimensions are in principle
testable. However, for very small 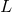 these modes have a very high-energy and are very difficult to excite
(to “see” an object of length
these modes have a very high-energy and are very difficult to excite
(to “see” an object of length 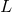 one needs wavelengths of the same order or smaller), thereby providing
a plausible explanation for the non-observation of extra dimensions in everyday laboratory
experiments.
one needs wavelengths of the same order or smaller), thereby providing
a plausible explanation for the non-observation of extra dimensions in everyday laboratory
experiments.
The attempts by Kaluza and Klein to unify gravity and electromagnetism considered five-dimensional Einstein field equations with the metric appropriately decomposed as,
Here,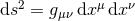 is a four-dimensional geometry,
is a four-dimensional geometry, 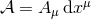 is a gauge field and
is a gauge field and 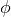 is a scalar;
the constant
is a scalar;
the constant 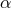 can be chosen to yield the four-dimensional theory in the Einstein frame. Assuming
all the fields are independent of the extra dimension
can be chosen to yield the four-dimensional theory in the Einstein frame. Assuming
all the fields are independent of the extra dimension  , one finds a set of four-dimensional
Einstein–Maxwell-scalar equations, thereby almost recovering both GR and EM [283*]. This is the basic idea
behind the Kaluza–Klein procedure, which unfortunately failed due to the presence of the (undetected)
scalar field.
, one finds a set of four-dimensional
Einstein–Maxwell-scalar equations, thereby almost recovering both GR and EM [283*]. This is the basic idea
behind the Kaluza–Klein procedure, which unfortunately failed due to the presence of the (undetected)
scalar field.
The idea of using higher dimensions was to be revived decades later in a more sophisticated model,
eventually leading to SMT. The development of the gauge/gravity duality (see Section 3.3.1 below) and
TeV-scale scenarios in high-energy physics (see Section 3.3.2) highlighted the importance of understanding
Einstein’s equations in a generic number of dimensions. Eventually, the study of Einstein’s field equations in
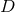 -dimensional backgrounds branched off as a subject of its own, where
-dimensional backgrounds branched off as a subject of its own, where 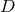 is viewed as just another
parameter in the theory. This area has been extremely active and productive and provides very important
information on the content of the field equations and the type of solutions it admits. Recently, GR in the
large
is viewed as just another
parameter in the theory. This area has been extremely active and productive and provides very important
information on the content of the field equations and the type of solutions it admits. Recently, GR in the
large 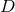 limit has been suggested as a new tool to gain insight into the
limit has been suggested as a new tool to gain insight into the 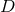 dependence of physical
processes [309].
dependence of physical
processes [309].
The uniqueness theorems, for example, are known to break down in higher dimensions, at least in the sense that solutions are uniquely characterized by asymptotic charges. BHs of spherical topology – the extension of the Kerr solution to higher dimensions – can co-exist with black rings [307*]. In fact, a zoo of black objects are known to exist in higher dimensions, but the dynamical behavior of this zoo (of interest to understand stability of the solutions and for collisions at very high energies) is poorly known, and requires NR methods to understand.
One other example requiring NR tools is the instability of black strings. Black strings are one of the simplest vacuum solutions one can construct, by extending trivially a four-dimensional Schwarzschild BH along an extra, flat direction. Such solutions are unstable against long wavelength perturbations in the fifth dimension, which act to fragment the string. This instability is known as the Gregory–Laflamme instability [367*]. The instability is similar in many aspects to the Rayleigh–Plateau instability seen in fluids, which does fragment long fluid cylinders [167]. However, the same scenario in the black string case would seem to lead to cosmic censorship violation, since the pinch-off would be accompanied by (naked) regions of unbounded curvature.4 Evidence that the Gregory–Laflamme does lead to disruption of strings was recently put forward [511*].
3.3 High-energy physics
3.3.1 The gauge/gravity duality
The gauge/gravity duality, or AdS/CFT correspondence, is the conjecture, first proposed by Maldacena in 1998 [536], and further developed in [798, 372], that string theory on an AdS spacetime (times a compact space) is dual (i.e., equivalent under an appropriate mapping) to a CFT defined on the boundary of the AdS space. Since its proposal, this conjecture has been supported by impressive and compelling evidence, it has branched off to, e.g., the AdS/Condensed Matter correspondence [396*], and it has inspired other proposals of duality relations with a similar spirit, such as the dS/CFT correspondence [731] and the Kerr/CFT correspondence [373]. All these dualities are examples of the holographic principle, which has been proposed in the context of quantum gravity [737, 734], stating that the information contained in a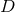 -dimensional gravitational system is encoded in some boundary of the system. The
paradigmatic example of this idea is a BH spacetime, whose entropy is proportional to the horizon
area.
-dimensional gravitational system is encoded in some boundary of the system. The
paradigmatic example of this idea is a BH spacetime, whose entropy is proportional to the horizon
area.
These dualities – in which strong gravity systems play a crucial role – offer tools to probe strongly coupled
gauge theories (in  dimensions) by studying classical gravity (in
dimensions) by studying classical gravity (in 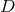 dimensions). For
instance, the confinement/deconfinement phase transition in quantum chromodynamics-like
theories has been identified with the Hawking–Page phase transition for AdS BHs [799]. Away
from thermal equilibrium, the quasi-normal frequencies of AdS BHs have been identified with
the poles of retarded correlators describing the relaxation back to equilibrium of a perturbed
dual field theory [439, 104]. The strongly coupled regime of gauge theories is inaccessible to
perturbation theory and therefore this new tool has created expectations for understanding
properties of the plasma phase of non-Abelian quantum field theories at non-zero temperature,
including the transport properties of the plasma and the propagation and relaxation of plasma
perturbations, experimentally studied at the Relativistic Heavy Ion Collider and now also at the
LHC [189]. Strong coupling can be tackled by lattice-regularized thermodynamical calculations of
quantum chromodynamics, but the generalization of these methods beyond static observables to
characterizing transport properties has limitations, due to computational costs. An example of an
experimentally accessible transport property is the dimensionless ratio of the shear viscosity
to the entropy density. Applying the gauge/gravity duality, this property can be computed
by determining the absorption cross section of low-energy gravitons in the dual geometry (a
BH/black brane) [490], obtaining a result compatible with the experimental data. This has
offered the holographic description of heavy ion collisions phenomenological credibility. An
outstanding theoretical challenge in the physics of heavy ion collisions is the understanding of the
‘early thermalization problem’: the mechanism driving the short – less than 1 fm/c [414] –
time after which experimental data agrees with a hydrodynamic description of the quark-gluon
plasma. Holography uses
dimensions). For
instance, the confinement/deconfinement phase transition in quantum chromodynamics-like
theories has been identified with the Hawking–Page phase transition for AdS BHs [799]. Away
from thermal equilibrium, the quasi-normal frequencies of AdS BHs have been identified with
the poles of retarded correlators describing the relaxation back to equilibrium of a perturbed
dual field theory [439, 104]. The strongly coupled regime of gauge theories is inaccessible to
perturbation theory and therefore this new tool has created expectations for understanding
properties of the plasma phase of non-Abelian quantum field theories at non-zero temperature,
including the transport properties of the plasma and the propagation and relaxation of plasma
perturbations, experimentally studied at the Relativistic Heavy Ion Collider and now also at the
LHC [189]. Strong coupling can be tackled by lattice-regularized thermodynamical calculations of
quantum chromodynamics, but the generalization of these methods beyond static observables to
characterizing transport properties has limitations, due to computational costs. An example of an
experimentally accessible transport property is the dimensionless ratio of the shear viscosity
to the entropy density. Applying the gauge/gravity duality, this property can be computed
by determining the absorption cross section of low-energy gravitons in the dual geometry (a
BH/black brane) [490], obtaining a result compatible with the experimental data. This has
offered the holographic description of heavy ion collisions phenomenological credibility. An
outstanding theoretical challenge in the physics of heavy ion collisions is the understanding of the
‘early thermalization problem’: the mechanism driving the short – less than 1 fm/c [414] –
time after which experimental data agrees with a hydrodynamic description of the quark-gluon
plasma. Holography uses 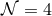 Super Yang–Mills theory as a learning ground for the real
quark-gluon plasma. Then, the formation of such plasma in the collision of high-energy ions has been
modeled, in its gravity dual, by colliding gravitational shock waves in five-dimensional AdS
space [205*]. These strong gravity computations have already offered some insight into the early
thermalization problem, by analyzing the formation and settling down of an AdS BH in the
collision process. But the use of shock waves is still a caricature of the process, which could be
rendered more realistic, for instance, by colliding other highly boosted lumps of energy or BHs in
AdS.
Super Yang–Mills theory as a learning ground for the real
quark-gluon plasma. Then, the formation of such plasma in the collision of high-energy ions has been
modeled, in its gravity dual, by colliding gravitational shock waves in five-dimensional AdS
space [205*]. These strong gravity computations have already offered some insight into the early
thermalization problem, by analyzing the formation and settling down of an AdS BH in the
collision process. But the use of shock waves is still a caricature of the process, which could be
rendered more realistic, for instance, by colliding other highly boosted lumps of energy or BHs in
AdS.
Another example of gauge/gravity duality is the AdS/Condensed Matter correspondence,
between field theories that may describe superconductors and strong gravity [396*, 437, 397]. The
simplest gravity theory in this context is Einstein–Maxwell-charged scalar theory with negative
cosmological constant. The RN-AdS BH solution of this theory, for which the scalar field vanishes, is
unstable for temperatures 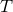 below a critical temperature
below a critical temperature 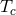 . If triggered, the instability
leads the scalar field to condense into a non-vanishing profile creating a scalar hair for the BH
and breaking the
. If triggered, the instability
leads the scalar field to condense into a non-vanishing profile creating a scalar hair for the BH
and breaking the 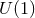 -gauge symmetry spontaneously. The end point of the instability is
a static solution that has been constructed numerically and has properties similar to those
of a superconductor [398*]. Thus, this instability of the RN-AdS BH at low temperature was
identified with a superconducting phase transition, and the RN-AdS and hairy BHs in the
gravitational theory, respectively, were identified with the normal and superconducting phases of a
holographic superconductor, realized within the dual field theory. Holographic superconductors are
a promising approach to understanding strongly correlated electron systems. In particular,
non-equilibrium processes of strongly correlated systems, such as superconductors, are notoriously
difficult and this holographic method offers a novel tool to tackle this longstanding problem. In
the gauge/gravity approach, the technical problem is to solve the classical dynamics of strong
gravitational systems in the dual five-dimensional spacetime. Using the AdS/CFT dictionary, one then
extracts the dynamics of the phase transition for the boundary theory and obtains the time
dependence of the superconducting order parameter and the relaxation time scale of the boundary
theory.
-gauge symmetry spontaneously. The end point of the instability is
a static solution that has been constructed numerically and has properties similar to those
of a superconductor [398*]. Thus, this instability of the RN-AdS BH at low temperature was
identified with a superconducting phase transition, and the RN-AdS and hairy BHs in the
gravitational theory, respectively, were identified with the normal and superconducting phases of a
holographic superconductor, realized within the dual field theory. Holographic superconductors are
a promising approach to understanding strongly correlated electron systems. In particular,
non-equilibrium processes of strongly correlated systems, such as superconductors, are notoriously
difficult and this holographic method offers a novel tool to tackle this longstanding problem. In
the gauge/gravity approach, the technical problem is to solve the classical dynamics of strong
gravitational systems in the dual five-dimensional spacetime. Using the AdS/CFT dictionary, one then
extracts the dynamics of the phase transition for the boundary theory and obtains the time
dependence of the superconducting order parameter and the relaxation time scale of the boundary
theory.
3.3.2 Theories with lower fundamental Planck scale
As discussed in Section 3.2.4, higher-dimensional theories have been suggested since the early days of GR as a means to achieve unification of fundamental interactions. The extra dimensions have traditionally been envisaged as compact and very small (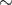 Planck length), in order to be compatible with high energy experiments. Around the turn of the
millennium, however, a new set of scenarios emerged wherein the extra dimensions are only probed by the
gravitational interaction, because a confining mechanism ties the standard model interactions to a
3 + 1-dimensional subspace (which is called the “brane”, while the higher-dimensional spacetime is called
the “bulk”). These models – also called “braneworld scenarios” – can be considered SMT inspired. The
main ideas behind them are provided by SMT, including the existence of extra dimensions and also the
existence of subspaces, namely Dirichlet-branes, on which a well defined mechanism exists to confine the
standard model fields.
Planck length), in order to be compatible with high energy experiments. Around the turn of the
millennium, however, a new set of scenarios emerged wherein the extra dimensions are only probed by the
gravitational interaction, because a confining mechanism ties the standard model interactions to a
3 + 1-dimensional subspace (which is called the “brane”, while the higher-dimensional spacetime is called
the “bulk”). These models – also called “braneworld scenarios” – can be considered SMT inspired. The
main ideas behind them are provided by SMT, including the existence of extra dimensions and also the
existence of subspaces, namely Dirichlet-branes, on which a well defined mechanism exists to confine the
standard model fields.
Our poor knowledge of the gravitational interaction at very short scales (below the millimeter at the time of
these proposals, below 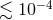 meters at the time of writing [802, 783*]), allows large [40, 46, 279*] or
infinitely large extra dimensions [638, 639]. The former are often called ADD models, whereas the latter are
known as Randall–Sundrum scenarios. Indeed these types of extra dimensions are compatible with high
energy phenomenology. Besides being viable, these models (or at least some of them) have the conceptual
appeal of providing an explanation for the “hierarchy problem” of particle physics: the large hierarchy
between the electroweak scale (
meters at the time of writing [802, 783*]), allows large [40, 46, 279*] or
infinitely large extra dimensions [638, 639]. The former are often called ADD models, whereas the latter are
known as Randall–Sundrum scenarios. Indeed these types of extra dimensions are compatible with high
energy phenomenology. Besides being viable, these models (or at least some of them) have the conceptual
appeal of providing an explanation for the “hierarchy problem” of particle physics: the large hierarchy
between the electroweak scale (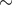 250 GeV) and the Planck scale (
250 GeV) and the Planck scale (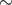
 ), or
in other words, why the gravitational interaction seems so feeble as compared to the other
fundamental interactions. The reason would be that whereas nuclear and electromagnetic interactions
propagate in 3 + 1 dimensions, gravity propagates in
), or
in other words, why the gravitational interaction seems so feeble as compared to the other
fundamental interactions. The reason would be that whereas nuclear and electromagnetic interactions
propagate in 3 + 1 dimensions, gravity propagates in 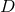 dimensions. A 3 + 1-dimensional
application of Gauss’s law then yields an incomplete account of the total gravitational flux.
Thus, the apparent (3 + 1-dimensional) gravitational coupling appears smaller than the real
(
dimensions. A 3 + 1-dimensional
application of Gauss’s law then yields an incomplete account of the total gravitational flux.
Thus, the apparent (3 + 1-dimensional) gravitational coupling appears smaller than the real
(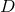 -dimensional) one. Or, equivalently, the real fundamental Planck energy scale becomes much smaller
than the apparent one. An estimate is obtained considering the
-dimensional) one. Or, equivalently, the real fundamental Planck energy scale becomes much smaller
than the apparent one. An estimate is obtained considering the 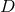 -dimensional gravitational
action and integrating the compact dimensions by assuming the metric is independent of them:
-dimensional gravitational
action and integrating the compact dimensions by assuming the metric is independent of them:
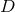 -dimensional one by the volume of the
compact dimensions
-dimensional one by the volume of the
compact dimensions 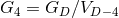 .
.
In units such that 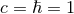 (different from the units
(different from the units  used in the rest of
this paper), the mass-energy Planck scale in four dimensions
used in the rest of
this paper), the mass-energy Planck scale in four dimensions 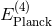 is related to Newton’s
constant by
is related to Newton’s
constant by 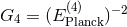 , since
, since 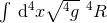 has the dimension of length squared;
similar dimensional arguments in Eq. (8*) show that in
has the dimension of length squared;
similar dimensional arguments in Eq. (8*) show that in 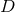 dimensions
dimensions 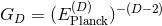 .
Therefore, the
.
Therefore, the 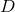 -dimensional Planck energy
-dimensional Planck energy 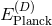 is related to the four-dimensional one by
is related to the four-dimensional one by
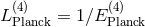 . For instance, for
. For instance, for
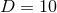 and taking the six extra dimensions of the order of the Fermi, Eq. (9*) shows that the
fundamental Planck scale would be of the order of a TeV. For a more detailed account of the braneworld
scenario, we refer the reader to the reviews [658, 532].
and taking the six extra dimensions of the order of the Fermi, Eq. (9*) shows that the
fundamental Planck scale would be of the order of a TeV. For a more detailed account of the braneworld
scenario, we refer the reader to the reviews [658, 532].
The real fundamental Planck scale sets the regime in particle physics beyond which gravitational
phenomena cannot be ignored and actually become dominant [736]; this is the trans-Planckian
regime in which particle collisions lead to BH formation and sizeable GW emission. A Planck
scale at the order of TeV (TeV gravity scenario) could then imply BH formation in particle
accelerators, such as the LHC, or in ultra high-energy cosmic rays [69, 279, 353]. Well into the
trans-Planckian regime, i.e., for energies significantly larger than the Planck scale, classical gravity
described by GR in 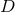 -dimensions is the appropriate description for these events, since the
formed BHs are large enough so that quantum corrections may be ignored on and outside the
horizon.
-dimensions is the appropriate description for these events, since the
formed BHs are large enough so that quantum corrections may be ignored on and outside the
horizon.
In this scenario, phenomenological signatures for BH formation would be obtained from the Hawking
evaporation of the micro BHs, and include a large multiplicity of jets and large transverse momentum as
compared to standard model backgrounds [1]. Preliminary searches of BH formation events in
the LHC data have already been carried out, considering 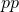 collisions with center-of-mass
energies up to 8 TeV; up to now, no evidence of BH creation has been found [201, 3, 202, 4].
To filter experimental data from particle colliders, Monte Carlo event generators have been
coded, e.g., [336], which need as input the cross section for BH formation and the inelasticity in
the collisions (gravitationally radiated energy). The presently used values come from apparent
horizon (AH) estimates, which in
collisions with center-of-mass
energies up to 8 TeV; up to now, no evidence of BH creation has been found [201, 3, 202, 4].
To filter experimental data from particle colliders, Monte Carlo event generators have been
coded, e.g., [336], which need as input the cross section for BH formation and the inelasticity in
the collisions (gravitationally radiated energy). The presently used values come from apparent
horizon (AH) estimates, which in 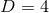 are known to be off by a factor of 2 (at least). In
are known to be off by a factor of 2 (at least). In
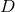 -dimensions, these values must be obtained from numerical simulations colliding highly boosted
lumps of energy, BHs or shock waves, since it is expected that in this regime ‘matter does not
matter’; all that matters is the amount of gravitational charge, i.e., energy, carried by the colliding
objects.
-dimensions, these values must be obtained from numerical simulations colliding highly boosted
lumps of energy, BHs or shock waves, since it is expected that in this regime ‘matter does not
matter’; all that matters is the amount of gravitational charge, i.e., energy, carried by the colliding
objects.

![1 ∫ √ --- S = ------ d4x − g[F (ϕ)R − 8πGZ (ϕ)gμν∂ μϕ∂νϕ − U (ϕ)] + Sm (ψm, gμν) (5 ) 16πG](article79x.gif)