List of Footnotes
| 1 | Notice that “strong field” is not synonymous with Planck-scale physics in this context. In fact, a stationary black hole would not serve as a probe of the strong field, even if one were to somehow acquire information about the gravitational potential close to the singularity. This is because any such observation would necessarily be lacking information about the dynamical sector of the gravitational interaction. Planck- scale physics is perhaps more closely related to strong-curvature physics. | |
| 2 | Stability and well-posedness are not the same concepts and they do not necessarily imply each other. For example, a well-posed theory might have stable and unstable solutions. For ill-posed theories, it does not make sense to talk about stability of solutions. | |
| 3 | The process of spontaneous scalarization in a particular type of scalar-tensor theory [129*, 130*] does introduce strong-field modifications because it induces non-perturbative corrections that can affect the structure of neutron stars. This subclass of scalar-tensor theories would satisfy Property (4). | |
| 4 | The model considered by [174*] is not phenomenological, but it contains a ghost mode. | |
| 5 | Technically, Einstein-Dilaton-Gauss–Bonnet gravity has a very particular set of coupling functions
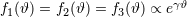 , where , where 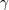 is a constant. However, in most cases one can expand about is a constant. However, in most cases one can expand about 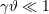 , so that the
functions become linear in the scalar field. , so that the
functions become linear in the scalar field. |
|
| 6 | Formally, as 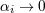 , one recovers GR with a dynamical scalar field. However, the latter does not couple to the metric or
the matter sector, so it does not lead to any observable effects that distinguish it from GR. , one recovers GR with a dynamical scalar field. However, the latter does not couple to the metric or
the matter sector, so it does not lead to any observable effects that distinguish it from GR. |
|
| 7 | The tensor 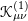 is sometimes written as is sometimes written as 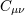 and referred to as the C-tensor. and referred to as the C-tensor. |
|
| 8 | A modern interpretation in terms of effective field theory can be found in [198, 199]. | |
| 9 | One should note in passing that more general black-hole solutions in scalar-tensor theories have been found [264, 91].
However, these usually violate the weak-energy condition, and sometimes they require unreasonably small values of 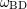 that
have already been ruled out by observation. that
have already been ruled out by observation. |
|
| 10 | The scalar field of Horbatsch and Burgess satisfies 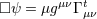 , and thus , and thus 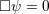 for stationary and axisymmetric
spacetimes, since the metric is independent of time an azimuthal coordinate. However, notice that is not necessarily needed for
Jacobson’s construction [246] to be possible. for stationary and axisymmetric
spacetimes, since the metric is independent of time an azimuthal coordinate. However, notice that is not necessarily needed for
Jacobson’s construction [246] to be possible. |
|
| 11 | All LISA bounds refer to the classic LISA configuration. | |
| 12 | Even if it is not linear, the effect should scale with positive powers of 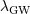 . It is difficult to think of any parity-violating
theory that would lead to an inversely proportional relation. . It is difficult to think of any parity-violating
theory that would lead to an inversely proportional relation. |
|
| 13 | Notice that these relations are independent of the polytropic constant 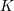 , where , where 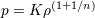 , as shown
in [452*]. , as shown
in [452*]. |

