Volume 4,
Issue 3 (GI8), 2003
Article
50
|
CONVOLUTION INEQUALITIES AND APPLICATIONS
SABUROU
SAITOH, VU KIM TUAN AND MASAHIRO YAMAMOTO
DEPARTMENT OF MATHEMATICS,
FACULTY OF ENGINEERING,
GUNMA UNIVERSITY,
KIRYU 376-8515, JAPAN
E-Mail: ssaitoh@math.sci.gunma-u.ac.jp
DEPARTMENT OF MATHEMATICS AND COMPUTER SCIENCE,
FACULTY OF SCIENCE,
KUWAIT UNIVERSITY,
P.O. BOX 5969,
SAFAT 13060 KUWAIT
E-Mail: vu@mcs.sci.kuniv.edu.kw
GRADUATE SCHOOL OF MATHEMATICAL SCIENCES,
THE UNIVERSITY OF TOKYO,
3-8-1 KOMABA MEGURO
TOKYO 153-8914, JAPAN
E-Mail: myama@ms.u-tokyo.ac.jp
Received 09 December, 2002; Accepted 15 April, 2003.
Communicated by: L.-E. Persson
|
ABSTRACT.
We introduce various convolution inequalities obtained
recently and at the same time, we give new type of reverse
convolution inequalities and their important applications to
inverse source problems. We consider the inverse problem of
determining 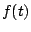 , , 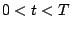 , in the heat source of
the heat equation , in the heat source of
the heat equation
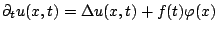 , , 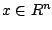 , , 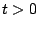 from the observation from the observation 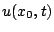 , , 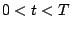 , at a remote
point , at a remote
point 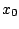 away from the support of away from the support of 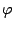 . Under an a priori assumption
that . Under an a priori assumption
that 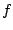 changes the signs at most changes the signs at most  -times, we give a conditional
stability of Hölder type, as an example of applications. -times, we give a conditional
stability of Hölder type, as an example of applications.
Key words:
Convolution, Heat source, Weighted convolution inequalities, Young's
inequality, Hölder's inequality, Reverse Hölder's inequality,
Green's function, Stability in inverse problems, Volterra's equation, Conditional stability of
Hölder type, Analytic semigroup, Interpolation inequality, Sobolev inequality.
2000 Mathematics Subject
Classification:
Primary 44A35; Secondary 26D20.
|
|
|
Download this article (PDF):
|
Suitable for a printer: 
Suitable for a monitor:
 |
To view these files we
recommend you save them to your file system and then view by using
the Adobe Acrobat Reader.
That is, click on the icon using the 2nd mouse button and
select "Save Target As..." (Microsoft Internet
Explorer) or "Save Link As..." (Netscape
Navigator).
See our PDF pages for more
information.
|
|
|
|
Other issues
-
Volume 1, Issue 1, 2000
-
Volume 1, Issue
2, 2000
-
Volume 2, Issue
1, 2001
-
Volume 2, Issue
2, 2001
-
Volume 2, Issue
3, 2001
-
Volume 3, Issue
1, 2002
-
Volume 3, Issue
2, 2002
-
Volume 3, Issue
3, 2002
-
Volume 3, Issue
4, 2002
-
Volume 3, Issue
5, 2002
-
Volume 4, Issue
1, 2003
-
Volume 4, Issue
2, 2003
-
Volume 4, Issue
3, 2003
-
Volume 4, Issue
4, 2003
-
Volume 4, Issue
5, 2003
|
|

