The idea of conformal symmetry was given in the monograph Colored Symmetry, its Generalizations and Applications by A.M. Zamorzaev, E.I. Galyarski and A.F. Palistrant (1978) as a generalization of similarity symmetry. Along with it, there was established the isomorphism between the symmetry groups of non-polar rods G31 and the conformal symmetry groups of the category C2. The isomorphism between the similarity symmetry groups S20 and the symmetry groups of polar rods G31 is pointed out within the analysis of the similarity symmetry groups in E2 (Chapter 3). According to the same isomorphism, the category C2 consists of the conformal symmetry groups isomorphic to the symmetry groups of non-polar rods G31.
In conformal symmetry groups of the category C2, besides the isometries and similarity transformations, which are the elements of the similarity symmetry groups S20, there will be, as basic transformations, three more conformal symmetry transformations: inversion RI in a circle with the center in the singular point O of the plane E2, inversional reflection ZI and inversional rotation SI. An inversion circle can be denoted by mI, inversional reflection center by 2I and inversional rotation center by nI.
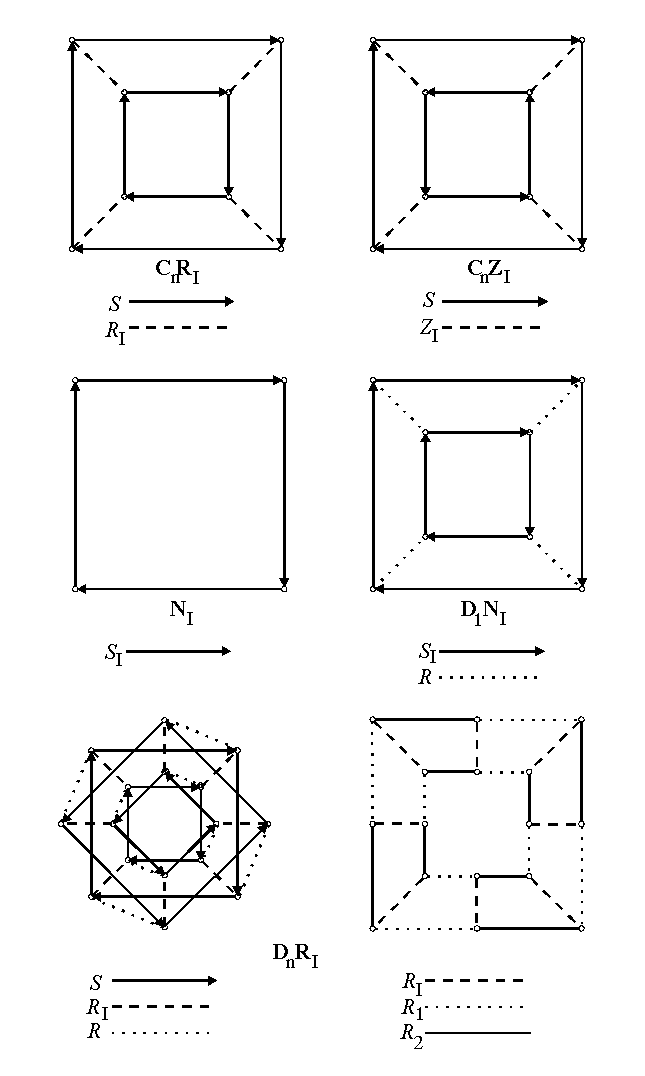
According to the isomorphism existing between the types of the discrete symmetry groups of non-polar rods G31 (A.V. Shubnikov, V.A. Koptsik, 1974) and the corresponding types of discrete conformal symmetry groups of the category C2, it is possible to conclude that there will be ten types of discrete conformal symmetry groups of the category C2.
Isomorphism between discrete symmetry groups of non-polar rods G31 and discrete conformal symmetry groups of category C2:
| KNI | (a)([2ñ]) | MNI | (a)([2ñ])ã |
| KCnRI | (a)n:m | KDnRI | (a)mn:m |
| KCnZI | (a)n:2 | MCnRI | (a)ãn:m |
| LCnZI | (at)n:2 | LCnRI | (a)(2n)n:m |
| KD1NI | (a)([2ñ])m | LDnRI | (a)m(2n)n:m |
Besides conformal symmetry groups of the category C2, there exist five types of discrete conformal symmetry groups of the category C21, isomorphic to the five existing types of the discrete symmetry groups of tablets G320 (A.V. Shubnikov, V.A. Koptsik, 1974): CnZ I @ n:2, CnRI @ n:m, NI @ ([2ñ]), D1NI @ ([2ñ])m and DnRI @ mn:m.
Those isomorphisms make possible the defining of the continuous conformal symmetry groups of the categories C2 and C21, which in these isomorphisms correspond, respectively, to the continuous symmetry groups of non-polar rods G31 and tablets G320.
Owing to the existence of a singular point, any figure the symmetry group of which is a conformal symmetry group is called a conformal symmetry rosette. All the conformal symmetry groups of the category C2 can be derived as the extensions of the conformal symmetry groups of the category C21 by the similarity transformations K, L, M. Therefore, every conformal symmetry rosette with a conformal symmetry group of the category C2 can be constructed, multiplying by those similarity transformations a conformal symmetry rosette with the corresponding conformal symmetry group of the category C21. Developing our analysis further we will consider first the conformal symmetry groups of the category C21. They will be used to generate conformal symmetry groups of the category C2. An analogous approach to the symmetry groups of rods G31 treated as extensions of the symmetry groups of tablets G320, is given by A.V. Shubnikov and V.A. Koptsik (1974).
According to the criterion of subordination, for the conformal symmetry groups of the category C2, it is possible to consider a certain type as the subtype of the more general type (e.g., the type KCnZI as the subtype of the type LCnZI, according to the relationship K = L(k,0) = L0). That and similar problems can be solved in the same way as with the similarity symmetry groups S20.
Presentations and structures of conformal symmetry groups of category C21:
NI {SI} SI2n = E C2n
D1NI {SI,RI} SI2n = E RI2 = E R2 = E RSI = SIR C2n×D1
CnRI {S,RI} Sn = E SRI = RIS Cn×D1
CnZI
{S,ZI}
Sn = ZI2 = (SZI)2 = E
Dn
{ZI,ZI'} ZI2 = ZI'2 = (ZIZI')n = E
DnRI
{S,R,RI}
Sn = R2 = (SR)2 = E RI2 = E
SRI = RIS
RRI = RIR Dn×D1
{R,R1,RI} R2 = R12 = (RR1)n = E RI2 = E
RRI = RIR R1RI = RIR1
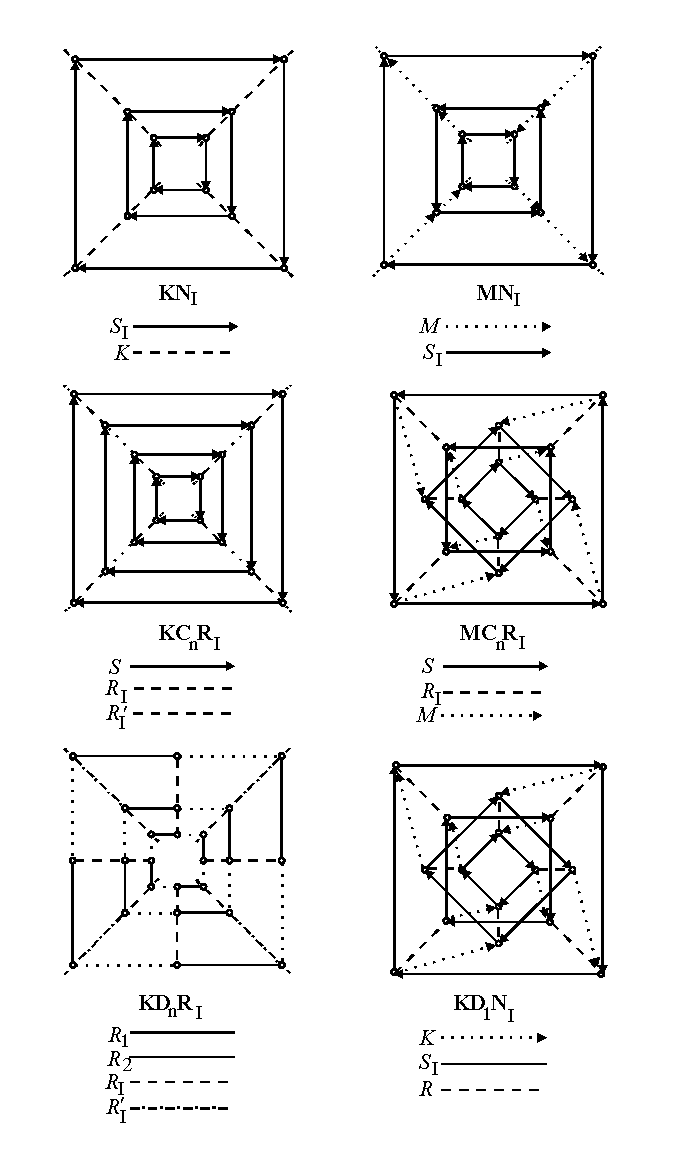
Presentations of conformal symmetry groups of category C2:
KNI {K,SI} SI2n = E KSIK = SI
MNI {M,SI} SI2n = E (MSI)2 = E
KD1NI {K,SI,R} SI2n = R2 = (RSI)2 = E KR = RK (KSI)2n = E
KCnRI {K,S,RI} Sn = RI2 = E SRI = RIS KS = SK (KRI)2 = E
KCnZI {K,S,ZI} Sn = ZI2 = (SZI)2 = E KS = SK (KZI)2 = E
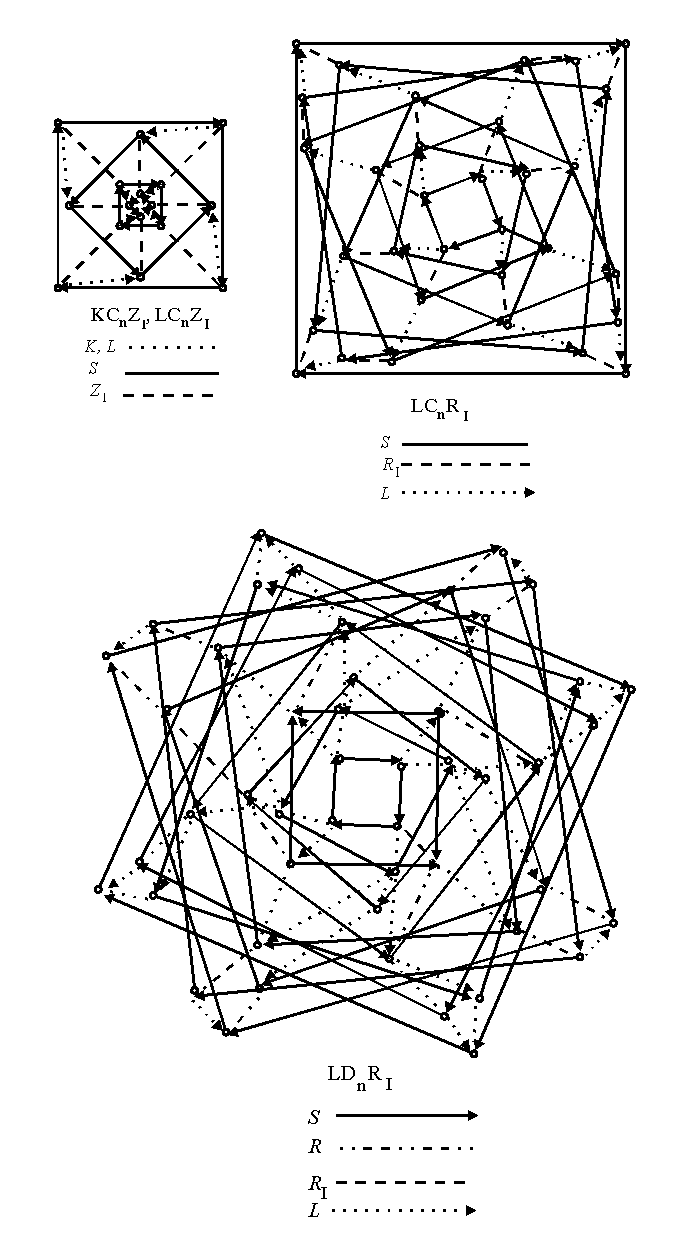
LCnRI {L,S,RI}
Sn = RI2 = E
SRI = RIS SL = LS
LRILRI = RILRIL = S (L = L2n = L(k,p/n))
LCnZI {L,S,ZI} Sn = ZI2 = (SZI)2 = E SL = LS (LZI)2 = E
MCnRI {M,S,RI} Sn = RI2 = E SRI = RIS SMS = M (MRI)2 = E
KDnRI {K,S,R,RI} Sn = R2 = (SR)2 = E
RI2 = E
SRI = RIS
RRI = RIR KS = SK KR = RK (KRI)2 = E
LDnRI
{L,S,R,RI} Sn = R2 = (SR)2 = E RI2 = E
SRI = RIS
RRI = RIR LS = LS LRLR = RLRL
LRILRI = RILRIL = S RLR = LS
(L = L2n = L(k,p/n))
Besides these presentations of conformal symmetry C2, it is possible to have presentations with a different choice of generators. For example, groups of the types KCnRI, KCnZI, KDnRI offer the following possibilities:
KCnRI {S,RI,RI'}
Sn = RI2 = RI'2 = E SRI = RIS
SRI' = RI'S
(RI' = RIK)
KCnZI {S,ZI,ZI'} Sn = ZI2 = ZI'2 = E
(SZI)2 = (SZI')2 = E
(ZI' = ZIK)
KDnRI {S,R,RI,RI'} Sn = R2 = (SR)2 = E
SRI = RIS SRI' = RI'S
RI2 = RI'2 = E RRI = RIR RRI' = RI'R
(RI' = RIK)
Similar possibilities exist in all the other conformal symmetry groups. Besides indicating the different presentation possibilities, certain choices of generators and corresponding presentations make possible a direct recognition of the structure of a group considered (e.g., the structure of groups of the type KCnRI is D¥×Cn, of groups of the type KDnRI is D¥×Dn, etc.).
Enantiomorphism:
the enantiomorphism exists in the conformal symmetry
groups C21
of the type CnZI type and
conformal symmetry groups of the
category C2 of
the types KCnZI and LCnRI.
Polarity of rotations:
polar rotations - NI, BCnRI,
KNI, KCnRI, LCnRI;
bipolar rotations - CnZI, MNI,
MCnRI,
KCnZI, LCnZI;
non-polar rotations - D1NI, DnRI,
KD1NI,
KDnRI, LnRI.
Polarity of radial rays:
polar - KNI;
bipolar - MNI,
KD1NI, KCnZI, LCnZI (if they
exist, i.e. if the dilative
rotation angle is a rational one);
non-polar - KCnRI, KDnRI,
MCnRI, LCnRI, LDnRI.
Form of the fundamental region:
unbounded in the conformal symmetry groups
C21; bounded in conformal
symmetry groups of the category C2. There exists
the possibility of varying
the shape of boundaries that do not belong to
reflection lines or inversion
circles. In conformal symmetry groups of the
category C21, variation of
all the boundaries is possible in groups of the
types NI, CnZI, of
non-reflectional boundaries in groups
of the type D1NI, of non-inversional
boundaries in groups of the type
CnRI, while in groups of the type DnRI,
it is not possible at all.
Regaring the changes of the form of a fundamental
region, conformal symmetry
groups of the category C2 offer similar
possibilities as generating
conformal symmetry groups of the category C21.
Number of edges of the fundamental region:
KCnRI,
KDnRI - 4;
KNI - 4,6;
MNI, KD1NI,
KCnZI,
LCnRI,
LCnZI, MCnRI,
LDnRI - 3,4,5,6.
Among the continuous conformal symmetry groups, visually presentable are groups D¥ RI of the category C21 and groups KD¥ RI, K1CnRI, K1DnRI, K1D¥ RI, L1CnZI of the category C2.
Cayley diagrams (Figure 4.1):


