| |
|
|
| |
Volume 9, Issue 1, Article 4 |
|
|
|
|
|
| |
On Rank Subtractivity Between Normal Matrices
|
| |
|
Authors: |
Jorma K. Merikoski, Xiaoji Liu, |
|
| |
|
Keywords:
|
Rank subtractivity, Minus partial ordering, Star partial ordering, Sharp partial ordering, Normal matrices, EP matrices. |
|
| |
|
Date Received:
|
13/07/2007 |
|
| |
|
Date Accepted:
|
05/02/2008 |
|
| |
|
Subject Codes: |
15A45, 15A18.
|
|
| |
|
Editors: |
Fuzhen Zhang, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
The rank subtractivity partial ordering is defined on 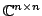 ( (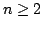 ) by ) by 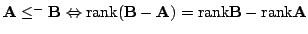 , and the star partial ordering by , and the star partial ordering by 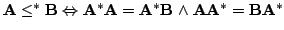 . If . If 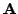 and and 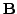 are normal, we characterize are normal, we characterize  . We also show that then . We also show that then 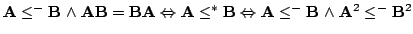 . Finally, we remark that some of our results follow from well-known results on EP matrices. . Finally, we remark that some of our results follow from well-known results on EP matrices.
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|



