| |
|
Abstract: |
It is known that in a strictly convex normed space, the 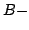 orthogonality (Birkhoff orthogonality) has the property, `` orthogonality (Birkhoff orthogonality) has the property, ``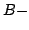 orthogonality is unique to the left``. Using this property, we introduce the definition of the so-called orthogonality is unique to the left``. Using this property, we introduce the definition of the so-called 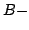 angle between two vectors, in a smooth and uniformly convex space. Also, we define the so-called angle between two vectors, in a smooth and uniformly convex space. Also, we define the so-called 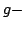 angle between two vectors. It is demonstrated that the angle between two vectors. It is demonstrated that the 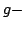 angle in a unilateral triangle, in a quasi-inner product space, is angle in a unilateral triangle, in a quasi-inner product space, is 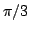 . The . The 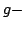 angle between a side and a diagonal, in a so-called angle between a side and a diagonal, in a so-called 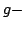 quandrangle, is quandrangle, is 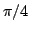 . .
|



