| |
|
|
| |
Volume 8, Issue 1, Article 6 |
|
|
|
|
|
| |
General System of Strongly Pseudomonotone Nonlinear Variational Inequalities Based on Projection Systems
|
| |
|
Authors: |
Ram U. Verma, |
|
| |
|
Keywords:
|
Strongly pseudomonotone mappings, Approximation solvability, Projection methods, System of nonlinear variational inequalities. |
|
| |
|
Date Received:
|
26/02/06 |
|
| |
|
Date Accepted:
|
11/12/06 |
|
| |
|
Subject Codes: |
49J40, 65B05, 47H20.
|
|
| |
|
Editors: |
Ram N. Mohapatra, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
Let 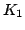 and and 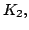 respectively, be non empty closed convex subsets of real Hilbert spaces respectively, be non empty closed convex subsets of real Hilbert spaces 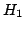 and and 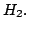 The The 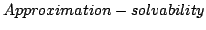 of a generalized system of nonlinear variational inequality of a generalized system of nonlinear variational inequality 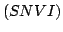 problems based on the convergence of projection methods is discussed. The SNVI problem is stated as follows: find an element problems based on the convergence of projection methods is discussed. The SNVI problem is stated as follows: find an element 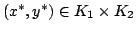 such that such that 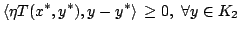 and for 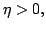 where 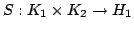 and and 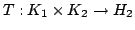 are nonlinear mappings. are nonlinear mappings.
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|




