|
JIPAM
| Gateaux Derivative and Orthogonality in $C_{p}$-classes |
|
|
|
|
| |
| |
Authors: |
Mecheri Salah, |
|
| |
Keywords:
|
Elementary operators, Schatten $p$-classes, orthogonality, Gateaux derivative. |
|
| |
Date Received:
|
01/09/05 |
|
| |
Date Accepted:
|
27/01/06 |
|
| |
Subject Codes: |
Primary 47B47, 47A30, 47B20; Secondary 4
|
|
| |
Editors: |
Alexander G. Babenko, |
|
| |
|
|
|
|
| |
Abstract: |
The general problem in this paper is minimizing the 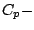 norm of suitable affine mappings from norm of suitable affine mappings from 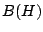 to to 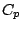 , using convex and differential analysis (Gateaux derivative) as well as input from operator theory. The mappings considered generalize the so-called elementary operators and in particular the generalized derivations, which are of great interest by themselves. The main results obtained characterize global minima in terms of (Banach space) orthogonality, and constitute an interesting combination of infinite-dimensional differential analysis, operator theory and duality. Note that the results obtained generalize all results in the literature concerning operator which are orthogonal to the range of a derivation and the techniques used have not been done by other authors.; , using convex and differential analysis (Gateaux derivative) as well as input from operator theory. The mappings considered generalize the so-called elementary operators and in particular the generalized derivations, which are of great interest by themselves. The main results obtained characterize global minima in terms of (Banach space) orthogonality, and constitute an interesting combination of infinite-dimensional differential analysis, operator theory and duality. Note that the results obtained generalize all results in the literature concerning operator which are orthogonal to the range of a derivation and the techniques used have not been done by other authors.;
|
This article was printed from JIPAM
http://jipam.vu.edu.au
The URL for this article is:
http://jipam.vu.edu.au/article.php?sid=692
|