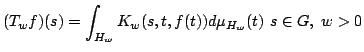JIPAM

On Global Approximation Properties of Abstract Integral Operators in Orlicz Spaces and Applications  
 
  Authors: Carlo Bardaro, Ilaria Mantellini,  
  Keywords: Modular approximation, nonlinear integral operators, regular families, singularity.  
  Date Received: 25/08/05  
  Date Accepted: 01/09/05  
  Subject Codes:

41A25, 41A35, 47G10, 46E30.

  
  Editors: Sever S. Dragomir,  
 
  Abstract:

In this paper we study approximation properties for the class of general integral operators of the form

$displaystyle (T_wf)(s) = int_{H_w} K_w (s,t, f(t)) dmu_{H_w}(t)    sin G, w>0$    

where $ G$ is a locally compact Hausdorff topological space, $ (H_w)_{w>0}$ is a net of closed subsets of $ G$ with suitable properties and, for every $ w>0,$ $ mu_{H_w}$ is a regular measure on $ H_w.$ We give pointwise, uniform and modular convergence theorems in abstract modular spaces and we apply the results to some kinds of discrete operators including the sampling type series.;


This article was printed from JIPAM
http://jipam.vu.edu.au

The URL for this article is:
http://jipam.vu.edu.au/article.php?sid=597