| |
|
|
| |
Volume 10, Issue 1, Article 27 |
|
|
|
|
|
| |
On a Subclass of Harmonic Univalent Functions
|
| |
|
Authors: |
K. K. Dixit, Saurabh Porwal, |
|
| |
|
Keywords:
|
Convex harmonic functions, Starlike harmonic functions, extremal problems. |
|
| |
|
Date Received:
|
04/06/08 |
|
| |
|
Date Accepted:
|
27/09/08 |
|
| |
|
Subject Codes: |
30C45, 31A05.
|
|
| |
|
Editors: |
Hari M. Srivastava, |
|
| |
|
|
|
|
|
| |
|
Abstract: |
The class of univalent harmonic functions on the unit disc satisfying the condition 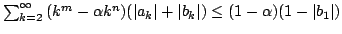 is given. Sharp coefficient relations and distortion theorems are given for these functions. In this paper we find that many results of Özturk and Yalcin [5] are incorrect. Some of the results of this paper correct the theorems and examples of [5]. Further, sharp coefficient relations and distortion theorems are given. Results concerning the convolutions of functions satisfying the above inequalities with univalent, harmonic and convex functions in the unit disc and harmonic functions having positive real part are obtained. is given. Sharp coefficient relations and distortion theorems are given for these functions. In this paper we find that many results of Özturk and Yalcin [5] are incorrect. Some of the results of this paper correct the theorems and examples of [5]. Further, sharp coefficient relations and distortion theorems are given. Results concerning the convolutions of functions satisfying the above inequalities with univalent, harmonic and convex functions in the unit disc and harmonic functions having positive real part are obtained.
|
|
|
|
|
|
| |
|
|
|
|
| |
|
|
|
|
|



